Assume that the force of gravitation $F \propto \frac{1}{r^{n}}$ . Then show that the orbital speed in a circular orbit of radius r is proportional to $\frac{1}{r^{(n - 1) / 2}}$ , while its period T is proportional to $r^{(n + 1) / 2}$

Video Solution

Text Solution

Verified by Experts

Kepler's first law: The orbit of a planet is an ellipse with the Sun at one of the foci.
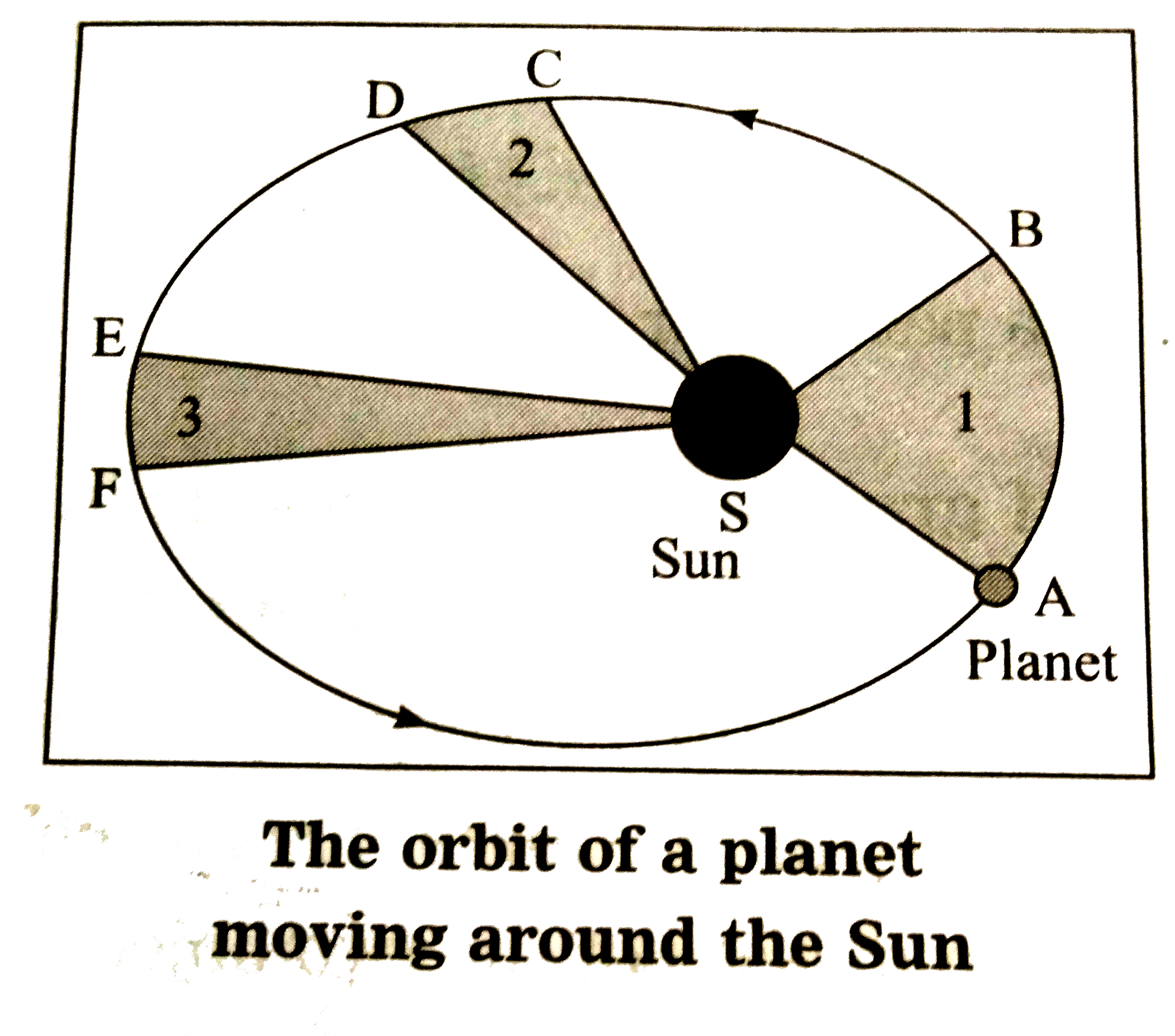
The adjoining figure shows the elliptical orbit of a planet revolving around the Sun. S denotes the position of the Sun.
Kepler's second law : The line joining the planet and the Sun Sweeps equal areas in equal intervals of time.
Area ASB=area CSD=area ESF.
Kepler's third law :
The square of the period of revolution of a plant around the sun is directly proportional to the cube of the mean distance of the planets from the Sun.
Thus , if r is the average distance of the planet from the Sun and T is its period of revolution , then,
$T^{2} \propto r^{3},$ i.e. $\frac{T^{2}}{r^{3}}$ =constant =K
For simplicity we shall assume the orbit to be a circle. In the adjoining figure, S denotes the poistion of the Sun, P denotes the position of a planet at a given instant and r denotes the radius of the orbit ( $\equiv$ the distance of the planet from the Sun ). Here, the speed of the planet is uniform. It is
$v = \frac{circumference of the circle}{period of revolution of the planet} = \frac{2 π r}{T}$
If m is the mass of the planet , the centripetal force exerted on the planet by the Sun ( $\equiv$ gravitational force), $F = \frac{m v^{2}}{r}$
$∴ F = \frac{m {(2 π r / T)}^{2}}{r} = \frac{4 π^{2} m r^{2}}{T^{2} r} = \frac{4 π^{2} m r}{T^{2}}$
Accordign to Kepler's third law,
$T^{2} = K r^{3}$
$∴ F = \frac{4^{2} m r}{K r^{3}} = \frac{4 π^{2} m}{K} (\frac{1}{r^{2}})$
Thus, $F \propto \frac{1}{r^{2}}$ as $\frac{4 π^{2} m}{K}$ is constant in a particular case.

Updated on:21/07/2023

The period of a satellite in a circular orbit of radius R is T. The period of another satellite in circular orbit of radius 4 R is :
A4 T
BT/4
CT/8
D8 T
A planet is revolving around a star in a circular orbit of radius R with a period T. If the gravitational force between the planet and the star is proportional to $R^{- 3 / 2}$ , then
A $T^{2} \propto R^{5 / 2}$
B $T^{2} \propto R^{- 7 / 2}$
C $T^{2} \propto R^{3 / 2}$
D $T^{2} \propto R^{4}$
If the gravitational force were $F \propto \frac{1}{R^{n}}$ , then the time period of a planet around the sun T will be proportional to
A $R^{- n}$
B $R^{\frac{(n - 1)}{2}}$
C $R^{\frac{(n + 1)}{2}}$
D $R^{n}$