D. N. A.:
Text Solution
D. N. A. decide the hereditary characters. They synthesise m-RNA according to its own nucleotide sequence and thereby help in the process of protein synthesis.
|
Topper's Solved these Questions
- CHARACTERISTICS/PROPERTIES USES/ADVANTAGES AND LIMITATIONS/EFFECTS/FUNCTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHARACTERISTICS/PROPERTIES USES/ADVANTAGES AND LIMITATIONS/EFFECTS/FUNCTIONSEXERCISE - LIFE PROCESSES IN LIVING ORGANISMS : PART-2
15 Videos
- CHARACTERISTICS/PROPERTIES USES/ADVANTAGES AND LIMITATIONS/EFFECTS/FUNCTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHARACTERISTICS/PROPERTIES USES/ADVANTAGES AND LIMITATIONS/EFFECTS/FUNCTIONSEXERCISE - SOCIAL HEALTH
1 Videos
- BOARD'S QUESTION PAPER (MARCH 2019)BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - BOARD'S QUESTION PAPER (MARCH 2019)EXERCISE - QUESTIONS
4 Videos
- CHART BASED QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHART BASED QUESTIONSEXERCISE - Disaster Management
3 Videos
Similar Questions
Knowledge Check
Which of the following will not give D.N.P test ?
ACH3−CHOBCH3−CO−CH3CCH3−O−CH3D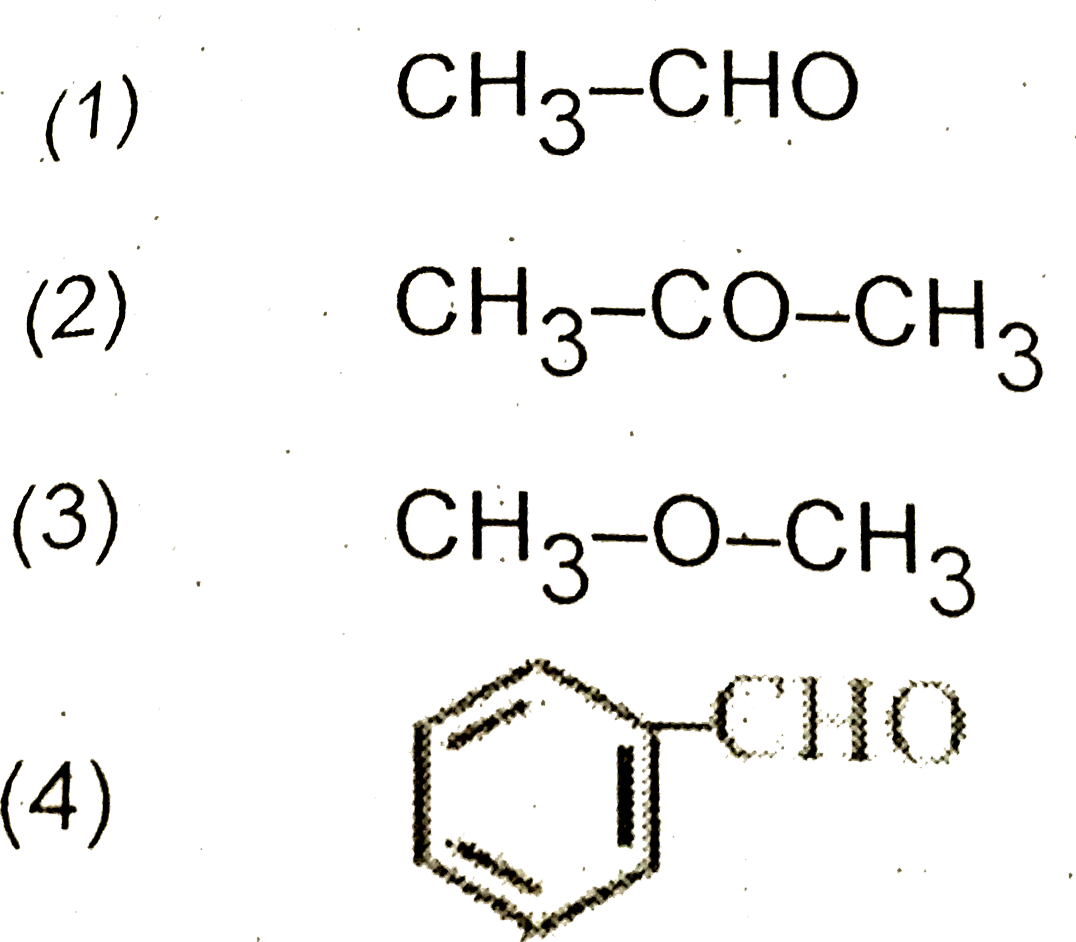
M is brother of N.B is brother of N.M is brother of D. But N is not brother of D. How is N related to D?
ANephewBCousinCSisterDBrotherIn the formulae Sn=n2{2a+(n−1)d} make d as the subject
The following steps are involved in solving the above problem. Arrange them in sequential order.
(A) (n−1)d=2Snn−2a
(B) Given, Sn=n2[2a+(n−1)d]⇒n[2a+(n−1)d]
=2Sn
(C) ⇒d=2n−1[Snn−a]
(D) 2a+(n−1)d=2SnnADBACBBDACCABDCDBDCA
In fig., D is a point on hypotenuse AC of ΔABC,DM⊥BCand DN⊥AB. Prove that
(i) DM2=DN⋅MC
(ii) DN2=DM⋅AN
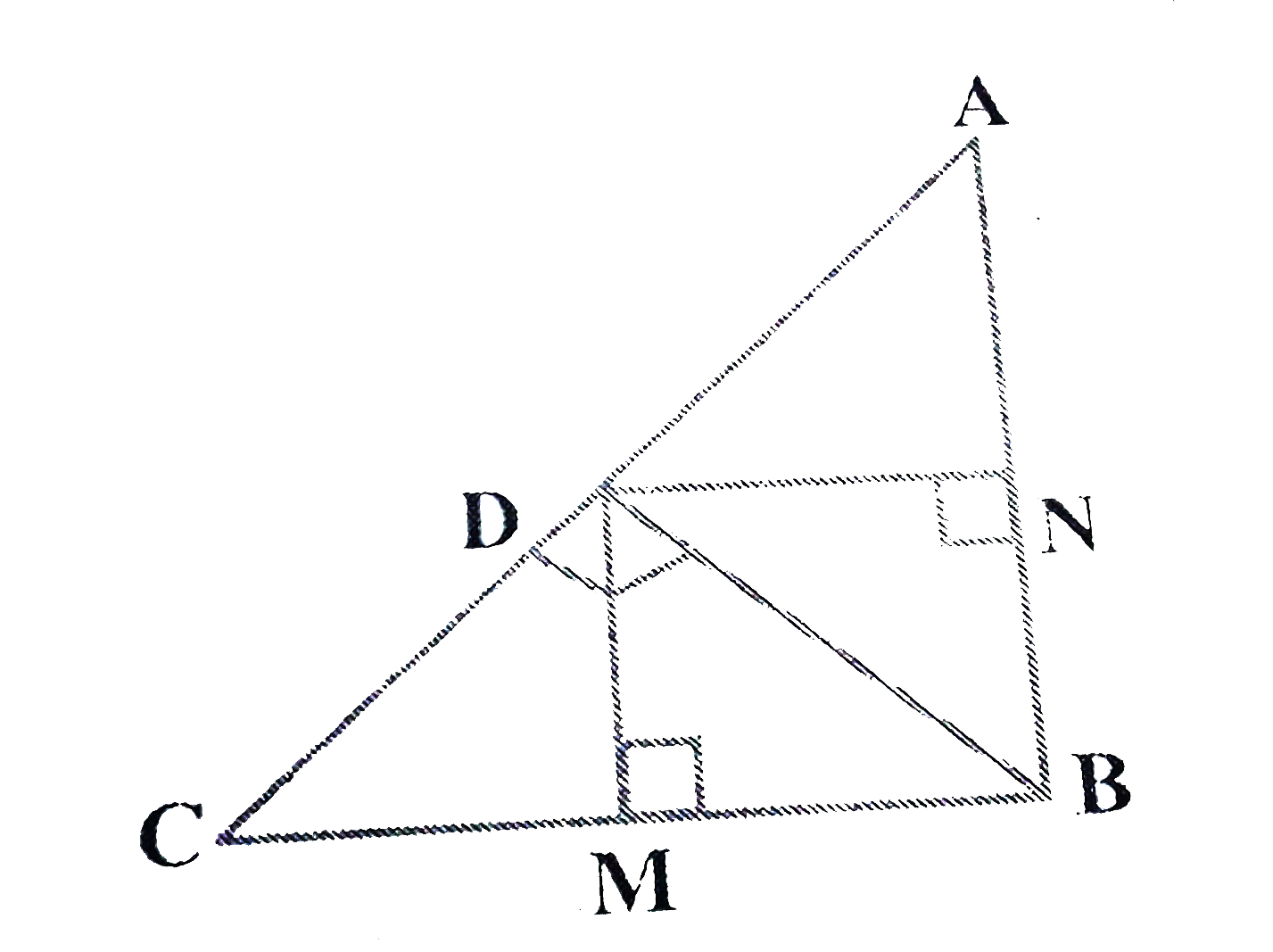
View SolutionIn fig., D is a point on hypotenuse AC of ΔABC,DM⊥BCand DN⊥AB. Prove that
(i) DM2=DN⋅MC
(ii) DN2=DM⋅AN

View SolutionIn Figure, ABC is a right triangle right angled at B and D is the foot of the the perpendicular drawn from B on AC . If DM⊥BC and DN⊥AB , prove that: (i)DM2=DN×MC (ii)DN2=DM×AN
View SolutionThe mean deviation of the series a,a+d,a+2d,.....,a+2n from its mean is (a)(n+1)d2n+1 (b) nd2n+1 (c) n(n+1)d2n+1 (d) (2n+1)dn(n+1)
View SolutionMean deviation of the series a,a+d,a+2d,.......,a+2nd from its mean is (a) (n+1)d(2n+1) (b) nd2n+1 (c) (2n+1)dn(n+1) (d) n(n+1)d2n+1
View Solution
