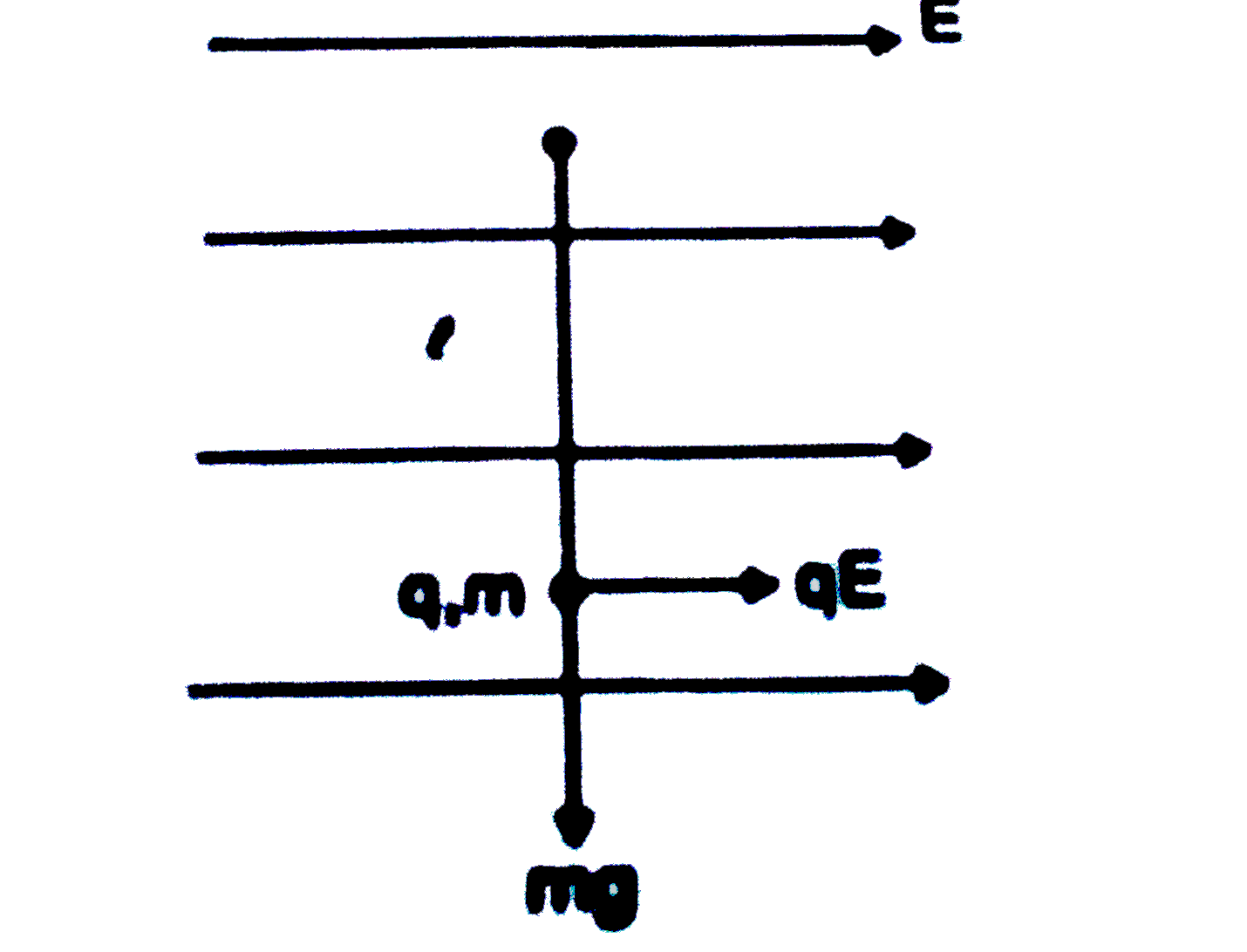
The bob of a simple pendulum has mass 2g and a charge of 5.0μC. It is at rest in a uniform horizontal electric field of intensity 2000V/m. At equilibrium, the angle that the pendulum makes with the vertical is: (take g=10m/s2)
tan−1(2.0)
tan−1(0.2)
tan−1(5.0)
tan−1(0.5)
The correct Answer is:B
To solve the problem of finding the angle that the pendulum makes with the vertical when it is in equilibrium in a uniform horizontal electric field, we can follow these steps:
1. Identify the Forces Acting on the Bob:
- The forces acting on the bob are:
- The gravitational force (weight) acting downwards, Fg=mg.
- The electric force acting horizontally due to the electric field, Fe=qE.
2. Calculate the Gravitational Force:
- The mass of the bob is given as 2g=2×10−3kg.
- The acceleration due to gravity g=10m/s2.
- Therefore, the gravitational force is:
Fg=mg=(2×10−3kg)(10m/s2)=2×10−2N=0.02N
3. Calculate the Electric Force:
- The charge on the bob is given as q=5.0μC=5.0×10−6C.
- The electric field intensity is E=2000V/m.
- Therefore, the electric force is:
Fe=qE=(5.0×10−6C)(2000V/m)=1.0×10−3N=0.001N
4. Set Up the Equilibrium Condition:
- At equilibrium, the horizontal electric force Fe is balanced by the horizontal component of the tension T in the string, and the vertical component of the tension balances the weight of the bob.
- Let T be the tension in the string and θ be the angle with the vertical.
- The components of the tension can be expressed as:
- Vertical component: Tcos(θ)=mg
- Horizontal component: Tsin(θ)=Fe
5. Divide the Two Equations:
- Dividing the horizontal and vertical components gives:
Tsin(θ)Tcos(θ)=Femg
- This simplifies to:
tan(θ)=Femg
6. Substitute the Values:
- Substitute Fe=0.001N and mg=0.02N:
tan(θ)=0.0010.02=120
7. Calculate the Angle θ:
- To find θ, take the arctangent:
θ=tan−1(120)
Final Answer:
The angle θ that the pendulum makes with the vertical is:
θ≈2.86∘
- The forces acting on the bob are:
- The gravitational force (weight) acting downwards, Fg=mg.
- The electric force acting horizontally due to the electric field, Fe=qE.
- The mass of the bob is given as 2g=2×10−3kg.
- The acceleration due to gravity g=10m/s2.
- Therefore, the gravitational force is:
Fg=mg=(2×10−3kg)(10m/s2)=2×10−2N=0.02N
- The charge on the bob is given as q=5.0μC=5.0×10−6C.
- The electric field intensity is E=2000V/m.
- Therefore, the electric force is:
Fe=qE=(5.0×10−6C)(2000V/m)=1.0×10−3N=0.001N
- At equilibrium, the horizontal electric force Fe is balanced by the horizontal component of the tension T in the string, and the vertical component of the tension balances the weight of the bob.
- Let T be the tension in the string and θ be the angle with the vertical.
- The components of the tension can be expressed as:
- Vertical component: Tcos(θ)=mg
- Horizontal component: Tsin(θ)=Fe
- Dividing the horizontal and vertical components gives:
Tsin(θ)Tcos(θ)=Femg
- This simplifies to:
tan(θ)=Femg
- Substitute Fe=0.001N and mg=0.02N:
tan(θ)=0.0010.02=120
- To find θ, take the arctangent:
θ=tan−1(120)
The angle θ that the pendulum makes with the vertical is:
θ≈2.86∘
Topper's Solved these Questions
Similar Questions
A pendulum bob of mass 80mg and carrying a charge of 2×10−8C is at rest in a uniform, horizontal electric field of 20k Vm−1. Find the tension in the thread.
View SolutionA pendulum bob of mass m = 80 mg, carrying a charge of q=2×10−8C, is at rest in a horizontal, uniform electric field of E = 20,000 V/m. The tension T in the thread of the pendulum and the angle α it makes with vertical, is (take g=9.8m/s2)
View Solution
Knowledge Check
- Question 1 - Select One
The bob of a pendulum of mass 8μg carries an electric charge of 39.2×10−10 coulomb in an electric field of 20×103 volt/meter and it is at rest. The angle made by the pendulum with the vertical will be
A27∘B45∘C87∘D127∘ - Question 2 - Select One
A pendulum bob of mass 30.7×10−6kg and carrying a chargee 2×10−8C is at rest in a horizontal uniform electric field of 20000V/m. The tension in the thread of the pendulum is (g=9.8m/s2)
A3×104NB4×10−4NC5×10−4ND6×10−4N - Question 3 - Select One
A pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθ
A pendulum bob of mass 80mg and carrying a charge of 2×10−4 coulomb is at rest in a uniform horizontal electric field of 20kVm.The tension in the thread of the pendulum is x times10−4 N.Find value of x?
View SolutionA pendulum bob of mass 80 mg and carrying charge of 3×10−8C is placed in an horizontal electric field. It comes to equilibrium position at an angle of 37∘ with the vertical. Calculate the intensity of electric field. (g = 10m/s2 )
View SolutionA charged particle is suspended in equilibrium in a uniform vertical electric field of intensity 20000 V/m. If mass of the particle is 9.6×10−16 kg the charge on it and excess number of electrons on the particle are respectivly (g=10m/s2)
View SolutionA simple pendulum is suspended from the ceilling of a car taking a trun of radius 10 m at a seed of 36 km/h. Findte angle made by the string of the pendulum with the vertical if this angle does not dchange during the turn. Take g=10ms2.
View SolutionA simple pendulum has a length l & mass of bob m. The bob is given a charge q coulomb. The pendulum is suspended in a uniform horizontal electric field of strength E as shown in figure, then calculate the time period of oscillation when bob is slightly displaced from its mean position.
 View Solution
View Solution

