A rectangular coil (Dimension 5cm×2.5cm) with 100 turns, carrying a current of 3A in the origin and in the X-Z plane. A magnetic field of 1 T is applied along X-axis. If the coil is tilted through 45∘ about Z-axis, then the torque on the coil is :
0.38Nm
0.55Nm
0.42Nm
0.27Nm
The correct Answer is:A
To solve the problem, we need to calculate the torque (τ) on the rectangular coil when it is tilted at an angle of 45∘ about the Z-axis in a magnetic field. The formula for torque on a current-carrying coil in a magnetic field is given by:
τ=n⋅I⋅A⋅B⋅sin(θ)
Where:
- n = number of turns of the coil
- I = current flowing through the coil
- A = area of the coil
- B = magnetic field strength
- θ = angle between the normal to the coil and the magnetic field
Step 1: Calculate the area of the coil
The dimensions of the coil are given as 5cm×2.5cm. First, we convert these dimensions into meters:
5cm=0.05mand2.5cm=0.025m
Now, we calculate the area A:
A=length×width=0.05m×0.025m=0.00125m2
Step 2: Identify the other parameters
From the problem statement, we have:
- Number of turns, n=100
- Current, I=3A
- Magnetic field, B=1T
- Angle, θ=45∘
Step 3: Calculate the torque
Now we can substitute these values into the torque formula:
τ=n⋅I⋅A⋅B⋅sin(θ)
Substituting the known values:
τ=100⋅3⋅0.00125⋅1⋅sin(45∘)
We know that sin(45∘)=√22≈0.707.
Now substituting this value:
τ=100⋅3⋅0.00125⋅1⋅0.707
Calculating step-by-step:
1. 100⋅3=300
2. 300⋅0.00125=0.375
3. 0.375⋅0.707≈0.265125
Thus, the torque τ≈0.265N m.
Step 4: Rounding to appropriate significant figures
Rounding 0.265N m gives us approximately 0.27N m.
Final Answer
The torque on the coil when tilted at 45∘ is:
0.27N m
- n = number of turns of the coil
- I = current flowing through the coil
- A = area of the coil
- B = magnetic field strength
- θ = angle between the normal to the coil and the magnetic field
The dimensions of the coil are given as 5cm×2.5cm. First, we convert these dimensions into meters:
From the problem statement, we have:
- Number of turns, n=100
- Current, I=3A
- Magnetic field, B=1T
- Angle, θ=45∘
Now we can substitute these values into the torque formula:
2. 300⋅0.00125=0.375
3. 0.375⋅0.707≈0.265125
Rounding 0.265N m gives us approximately 0.27N m.
The torque on the coil when tilted at 45∘ is:
Topper's Solved these Questions
Similar Questions
A rectangular coil (Dimension 6cm × 4.5 cm ) with 200 turns, carrying a current 3A in clock-wise direction, is kept centered at the origin and in the X – Z plane. A magnetic field of 1T is applied along X-axis. If the coil is tilted through 30∘ about Z-axis, then what will be the numerical value of torque on the coil in Nm?
View SolutionA rectangular coil is made of 100 turns and has dimension of 50cm×20cm, carrying a current of 10A It is placed inside a magnetic field of 5 T making an angle of 30∘ with the field. What is the torque acting on the coil?
View Solution
Knowledge Check
A rectangular coil of area of cross-section is placed as shown. A magnetic field B is applied along Z-axis. The torque on the coil is
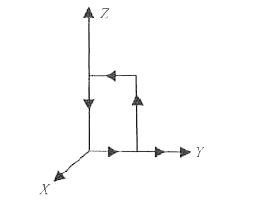 AABI along + Y-axisBABI along - Y-axisCABI along + X-axisDABI along - X-axis
AABI along + Y-axisBABI along - Y-axisCABI along + X-axisDABI along - X-axisA rectangular coil of 50 turns, each of area 0.1m2and carrying a current of 1 A is held perpendicular to a magnetic field of 0.1 T. What is the torque acting on the coil?
A0.5NmB1NmCzeroD0.001NmA coil of radius 2 cm and 500 turns, carries of a current of 2A. It is placed in a uniform magnetic field of induction 0.5T. What is the torque acting on the coil if theta=30◦?
A0.314N−mB0.628NmC0.157N−mD6.28Nm
A rectangular coil of sides 8cm and 6cm haivng 2000 turns and carrying a current of 200mA is placed in a uniform magnetic field of 0⋅2T directed along the positive x-axis. (a) What is the maximum torque the coil can experience? In which orientation does it experience the maximum Torque? (b) For which orientations of the coil is the torque zero? When is this equilibrium stable and unstable?
View SolutionA circular coil of 20 turns and radius 10cm is placed in uniform magnetic field of 0.10T normal to the plane of the coil.If the current coil is 5A ,then the torque acting on the coil will be: ?
View SolutionA circular coil of radius 10cmand100 turns carries a current 1A. What is the magnetic moment of the coil?
View SolutionA rectangular coil 20cm×20cm has 100 turns and carries a current of 1A. It is placed in a uniform magnetic field B=0.5T with the direction of magnetic field parallel to the plane of the coil. The magnitude of the torque required to hold this coil in this position is
View SolutionA circular coil of radius 10 cm having 100 turns carries a current of 3.2 A. The magnetic field at the center of the coil is
View Solution
