An X-ray tube operates at 40 kV. Suppose the electron converts 70% of its energy into a photon at each collision. Find the lowest three wavelengths emitted from the tube. Neglect the energy imparted to the atom with which the electron collides.

Video Solution

Text Solution

Verified by Experts

Given $v = 40 K V = 40 \times 10^{3}$
$E \neq r g y = 40 \times 10^{3} e V$
$E \neq r g y U t i l i s e d = \frac{70}{100} \times 40 \times 10^{3}$
$= 28 \times 10^{3} e V$
$λ = \frac{h c}{E} = \frac{1242 - e V n m}{28 \times 10^{3} e V}$
$= 44.35 \times 10^{3} n m$
$44.35 p m$
For other wavelength
$E = 70 % (L e f t o v e r e \neq r g y)$
$= \frac{70}{100} \times (40 - 28) 10^{3}$
$= \frac{70}{100} \times 12 \times 10^{3}$
$84 \times 10^{2}$
$λ = \frac{h c}{E} = \frac{1242}{8.4 \times 10^{3}}$
$= 147.86 \times 10^{3} n m$
$147.86 p m = 148 p m$
for third wavelength ,
$E = \frac{70}{100} (12 - 8.4) \times 10^{3}$
$= 7 \times 3.6 \times 10^{2} = 25.2 \times 10^{2}$
$λ = \frac{h c}{E} = \frac{1242}{25.2 \times 10^{2}} ~ < b r >$ =49.2857xx10^-2 $< b r$ =493p m

Updated on:21/07/2023

Consider the electron energy level diagram of H-atom Photons associated with shortest and longest wavelength would be emitted from the atom by the transitions labeled

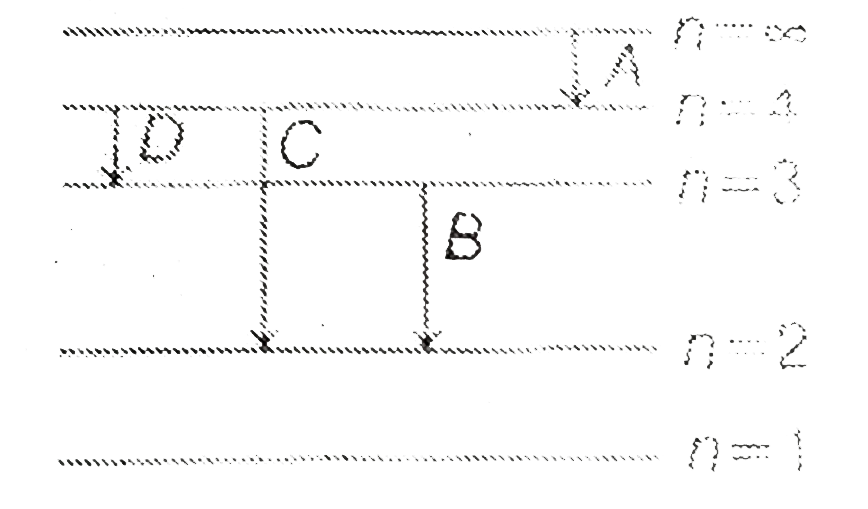AD and C respcitively
BC and A respectively
CC and D respectivley
DA and C respectively
An X-ray tube operating at 50kV converts $1 %$ energy in the form of X-ray. If the amount of heat produced is 495 watt, then the number of electrons colliding with the target per second is
A $6.25 \times 10^{16}$
B $4.15 \times 10^{16}$
C $3.2 \times 10^{16}$
D $1.2 \times 10^{16}$