Theorem 6.8 : If a side of a triangle is produced, then the exterior angle so formed is equal to the sum of the two interior opposite angles.
To prove Theorem 6.8, which states that if a side of a triangle is produced, then the exterior angle formed is equal to the sum of the two interior opposite angles, we can follow these steps:
1. Draw Triangle and Extend a Side:
- Let triangle ABC be given, and extend side BC to a point D.
- This creates an exterior angle ∠ACD.
2. Identify Angles:
- We need to prove that ∠ACD = ∠A + ∠B, where ∠A and ∠B are the interior opposite angles to the exterior angle ∠ACD.
3. Use the Triangle Angle Sum Property:
- According to the triangle angle sum property, the sum of the angles in triangle ABC is:
∠A+∠B+∠ACB=180∘
4. Identify Straight Line Angles:
- Since D is a point on the line extended from BC, we know that:
∠ACB+∠ACD=180∘
- This is because angles on a straight line sum up to 180 degrees.
5. Set Up the Equation:
- Now we have two equations:
1. ∠A+∠B+∠ACB=180∘
2. ∠ACB+∠ACD=180∘
6. Equate the Two Equations:
- Since both expressions equal 180 degrees, we can set them equal to each other:
∠A+∠B+∠ACB=∠ACB+∠ACD
7. Cancel Out the Common Angle:
- Subtract ∠ACB from both sides:
∠A+∠B=∠ACD
8. Conclusion:
- Therefore, we have proved that:
∠ACD=∠A+∠B
- This confirms Theorem 6.8.
- Let triangle ABC be given, and extend side BC to a point D.
- This creates an exterior angle ∠ACD.
- We need to prove that ∠ACD = ∠A + ∠B, where ∠A and ∠B are the interior opposite angles to the exterior angle ∠ACD.
- According to the triangle angle sum property, the sum of the angles in triangle ABC is:
∠A+∠B+∠ACB=180∘
- Since D is a point on the line extended from BC, we know that:
∠ACB+∠ACD=180∘
- This is because angles on a straight line sum up to 180 degrees.
- Now we have two equations:
1. ∠A+∠B+∠ACB=180∘
2. ∠ACB+∠ACD=180∘
- Since both expressions equal 180 degrees, we can set them equal to each other:
∠A+∠B+∠ACB=∠ACB+∠ACD
- Subtract ∠ACB from both sides:
∠A+∠B=∠ACD
- Therefore, we have proved that:
∠ACD=∠A+∠B
- This confirms Theorem 6.8.
Topper's Solved these Questions
- NCERT THEOREMSBOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 7.1
1 Videos
- NCERT THEOREMSBOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 7.2
1 Videos
- NCERT THEOREMSBOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 6.7
1 Videos
- LINES AND ANGLESBOOK - NCERTCHAPTER - LINES AND ANGLESEXERCISE - SOLVED EXAMPLES
8 Videos
- NUMBER SYSTEMSBOOK - NCERTCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.4
2 Videos
Similar Questions
Exterior angle theorem : If a side of a triangle is produced; the exterior angle so formed is equal to the sum of the two interior opposite angles.
View Solution(Exterior Angle Theorem): If a side of a triangle is produced, the exterior angle so formed is equal to the sum of the two interior opposite angles. GIVEN : A triangle ABC.D is a point of BC produced, forming exterior angle ∠4. TO PROVE : ∠4=∠1+∠2 i.e. , ∠ACD=∠CAB+∠CBA.
View Solution
Knowledge Check
one side of a triangle is produced and the exterior angle so formed is 140∘. If the interior opposite angles are in the ratio. 2:5, find the measure of each angle of the triangle.
A40∘,75∘,60∘B40∘,70∘,70∘C40∘,100∘,40∘D40∘,75∘,65∘
THEOREM (Exterior Angle Property of a Triangle) : If a side of a triangle is produced the exterior angle so formed is equal to the sum of two interior opposite angles.
View Solution27.Prove that an exterior angle of a triangle is equal to the sum of its interior opposite angles.
View SolutionIf one side of a cyclic quadrilateral is produced, then the exterior angle is equal to the interior opposite angle.
View SolutionIf one side of a cyclic quadrilateral is produced; then the exterior angle is equal to the interior opposite angle.
View SolutionIf a side of the cyclic quadrlateral is produced ; then the external angle is equal to the interior opposite angles.
View Solutionone side of a triangle is produced and the exterior angle so formed is 120∘.if the interior opposite angles be in the ratio 3:5.find the measure of each angles of triangle .
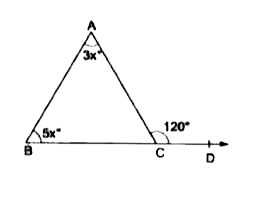
View SolutionThe measure of any exterior angle of a triangle is equal to the sum of the measures of its two interior opposite angles
View Solution
