Question

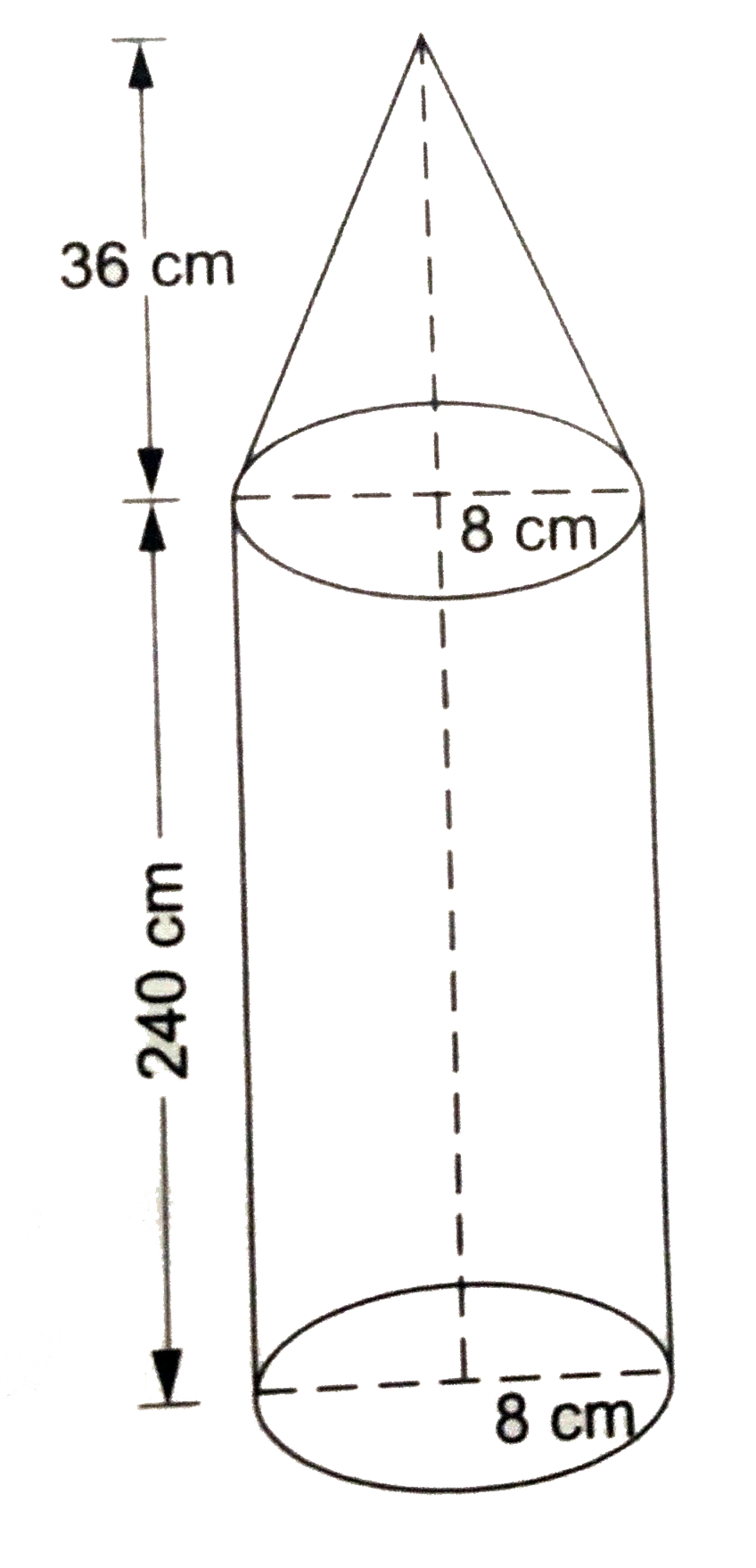
An iron pillar has some part in the from of right circular cylinder and the remaining in the from of a right circular cone .The radius of the base of each of the cone and the cylinder is 8 cm . The cylindrical part is 240 cm hight and the conical part is 36 cm hight .Find the weight of the pillar if 1 cubic centimetre of iron weighs 7.5 g .

Options

A. 480. 16 kg
B. `380. 16 kg`
C. 580. 16 kg
D. 680. 16 kg

Answer

B

Explanation

Radius of the cylinder , r= 8 cm
Radius of the cone r= 8 cm
Height of the cylinder h= 240 cm
Height of the cone H= 36 cm
Total volume of the iron
= volume of the cylinder + volume of the cone
` pi r^2h+1/3 pi r^2 H= pi r^2 (h+1/3 H)`
`=[22/7 xx 8 xx ( 240 + 1/3 xx 36 )] cm^3`
`= 50688 cm^3`
`therefore` weight of the pillar = volume in `cm^3 xx ` weight per `cm^3`
`=((50688 xx 7.5)/(1000))kg = 380.16 kg `
Hence , the weight of the pillar is 380. 16 kg .