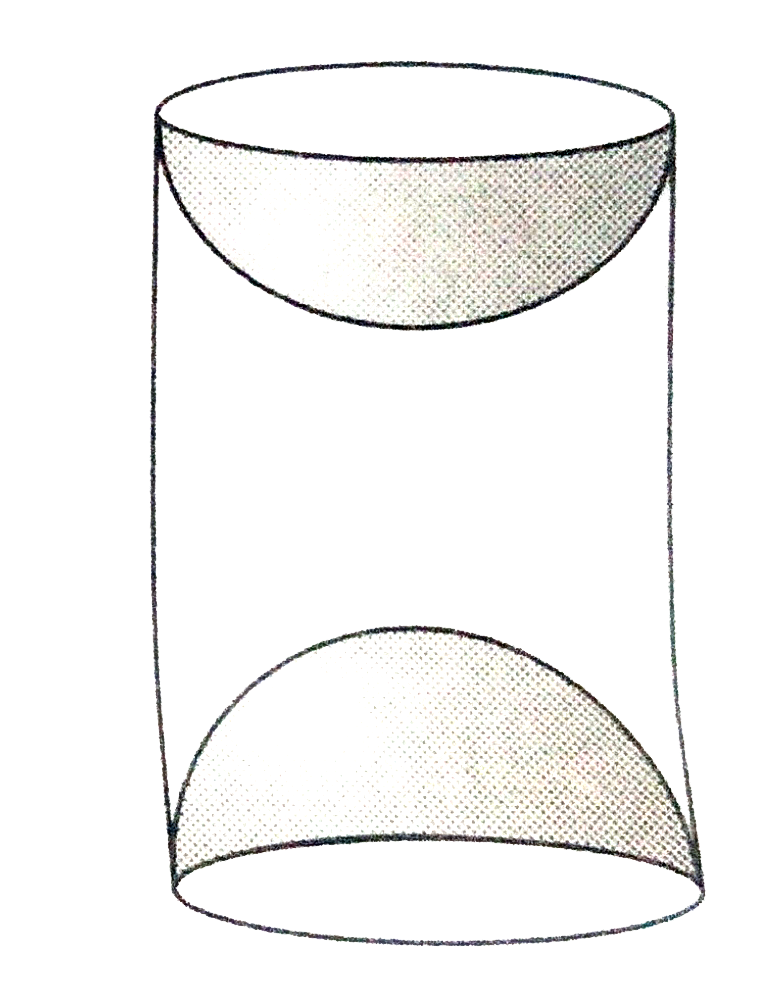
From each end of a solid metal cylinder, meral was scooped out in hemispherical form same diameter. The height of the cylinder is 10 cm and its base is of radius 4.2 cm. The rest the cylinder is melted and converted into a cylindrical wire of 1.4 cm thickness. Find the length of the wire.

Video Solution

Text Solution

Verified by Experts

Height of the cylnder, h = 10 cm.
Radius of each of the cylinder and hemisphere, r = 4.2 cm.
Volume of the solid
= volume of the cyliner - volume of 2 hemispheres
$= π r^{3} h - 2 \times \frac{2}{3} π r^{3} = π r^{3} (h - \frac{4}{3} r)$
$= [π \times 4.2 \times 4.2 \times (10 - \frac{4}{3} \times \frac{4}{2}) c m^{3}] .$
$= π \times 4.2 \times 4.2 \times 4.4) c m^{3}$ .
Let the length of the wire be H.
$Radius of the wire, R = (\frac{1.4}{2}) c m = 0.7 c m = \frac{7}{10} c m .$
$Volume of hte wire = π r^{2} H = (π \times \frac{7}{10} \times \frac{7}{10} \times H) c m^{3}$ .
Now, volume of the wire = volume of the solid
$\Rightarrow π \times \frac{7}{10} \times \frac{7}{10} \times H = π \times 4.2 \times 4.2 \times 4.4$
$\Rightarrow H = (\frac{4.2 \times 4.2 \times 4.4 \times 10 \times 10}{7 \times 7}) c m = (\frac{42 \times 42 \times 4.4}{7 \times 7}) c m$
$= 158.4 c m .$

Updated on:21/07/2023