In throwing of two dice, the number of exhaustive events that '5' will never appear on any one of the dice is
A
5
B
18
C
25
D
36
Video Solution
Text Solution
The correct Answer is:C
n(S)=6×6=36
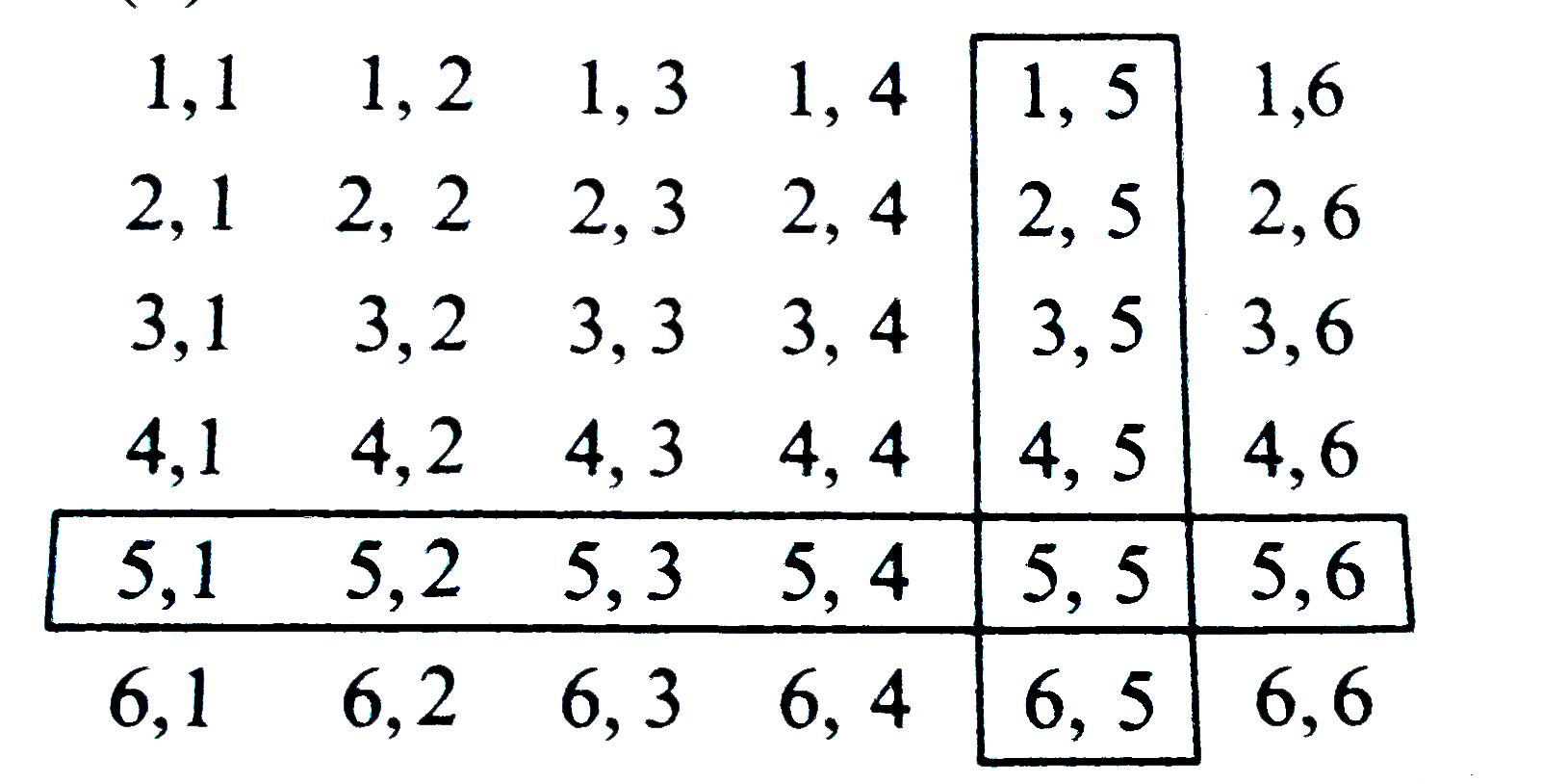
∴ Required number of exhausistance events
=(6−1)×(6−1)=5×5=25
∴ Option (c) is correct.
|
Topper's Solved these Questions
- POLYNOMIAL,QUADRATIC EQUATION & INEQUALITIESBOOK - NDA PREVIOUS YEARSCHAPTER - POLYNOMIAL,QUADRATIC EQUATION & INEQUALITIESEXERCISE - Math
170 Videos
- PROPERTIES OF TRIANGLE, INVERSE TRIGONOMETRIC FUNCTIONBOOK - NDA PREVIOUS YEARSCHAPTER - PROPERTIES OF TRIANGLE, INVERSE TRIGONOMETRIC FUNCTIONEXERCISE - MCQ
103 Videos
Similar Questions
Knowledge Check
In throwing of two dice, what is the number of exhaustive events ?
A6B12C36D18In throwing of two dice, what is the number of exhaustive events ?
A6B12C36D18In throwing of two dice, what is the number of exhaustive events ?
A6B12C36D18
Two different dice are tossed together. Find the probability that (i) the number on each die is even, (ii) the sum of the numbers appearing on the two dice is 5.
View SolutionGiven that the two numbers appearing on throwing two dice are different. Find the probability of the event the sum of numbers on the dice is 4.
View SolutionIn throwing of two dice, what is the number of exhaustive events ?
View SolutionIn throwing of two dice, the probability of getting a multiple of 4 is
View SolutionIn one throw of two dice, the probability of getting a number on first dice smaller than number on second dice, is,
View Solution
