Doubtnut Promotions Sticky

Let f(x)={1−|x|,|x|≤10, |x|>1 and, g(x)=f(x-1)+f(x+1) for all x∈R. Then, the value of ∫3−3g(x)dx, is

A

2

B

3

C

4

D

5

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

The graph of f(x) and g(x) are shown in Fig. 14 and 15 respectively.
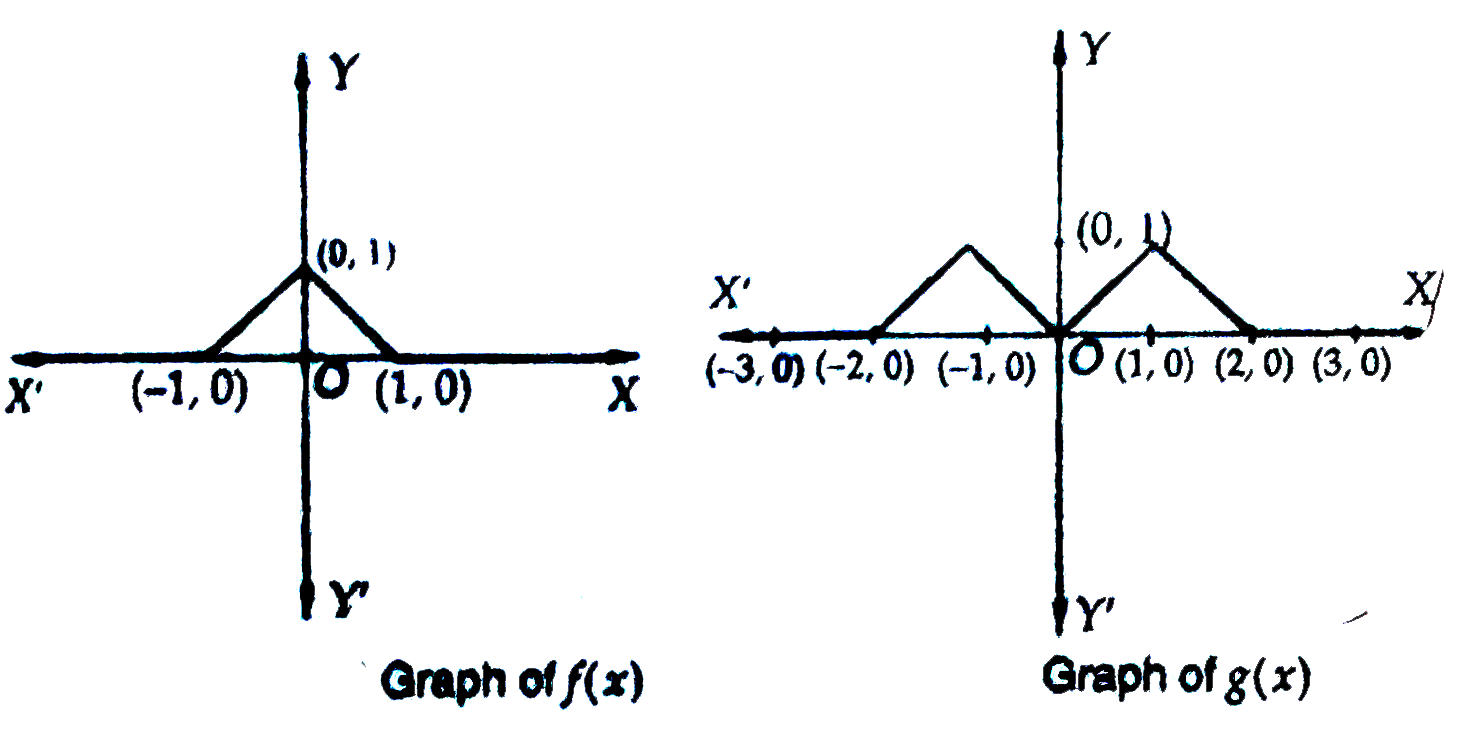
ocr_image
Clearly,
3∫−3g(x)dx=−2∫−30dx+0∫−2f(x)dx+2∫0f(x)dx+3∫20dx
⇒3∫−3g(x)dx=22∫0f(x)dx=2(12×2×1)=2

|
Updated on:21/07/2023
Class 12MATHSDEFINITE INTEGRAL

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    Let f(x)={1−|x|,|x|≤10,|x|>1andg(x)=f(x−1)for allx∈R The graph for g(x) is given by

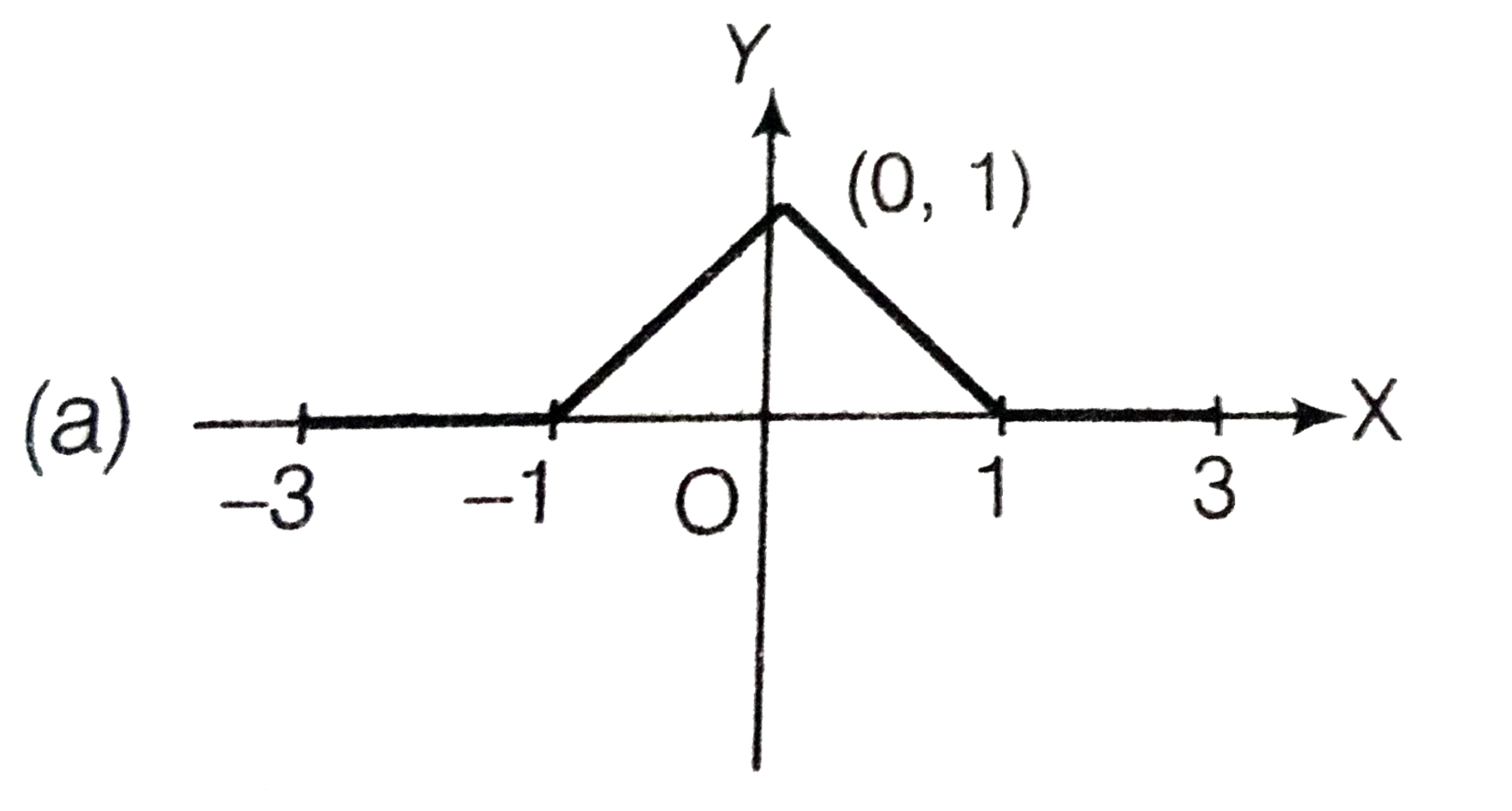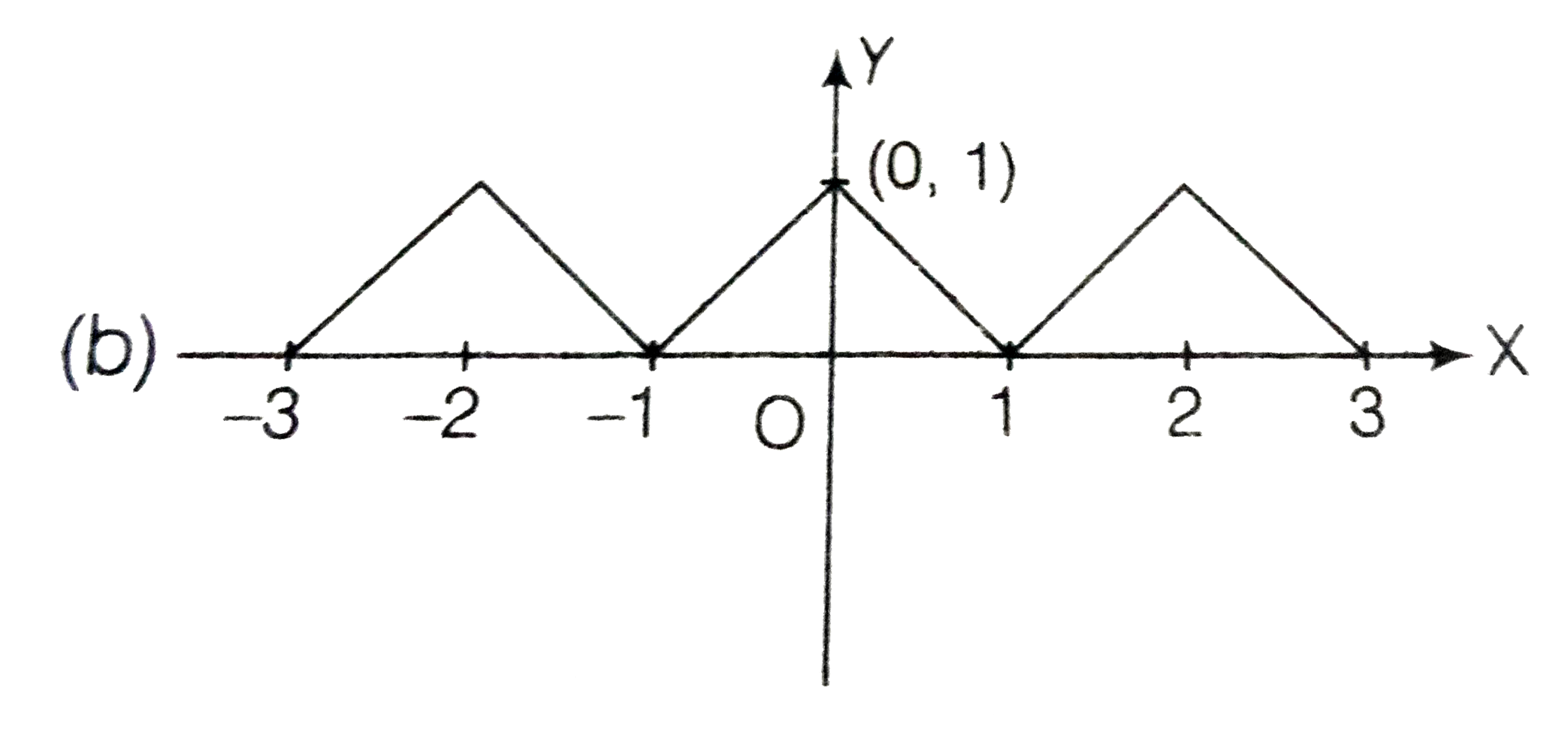    Aocr_image
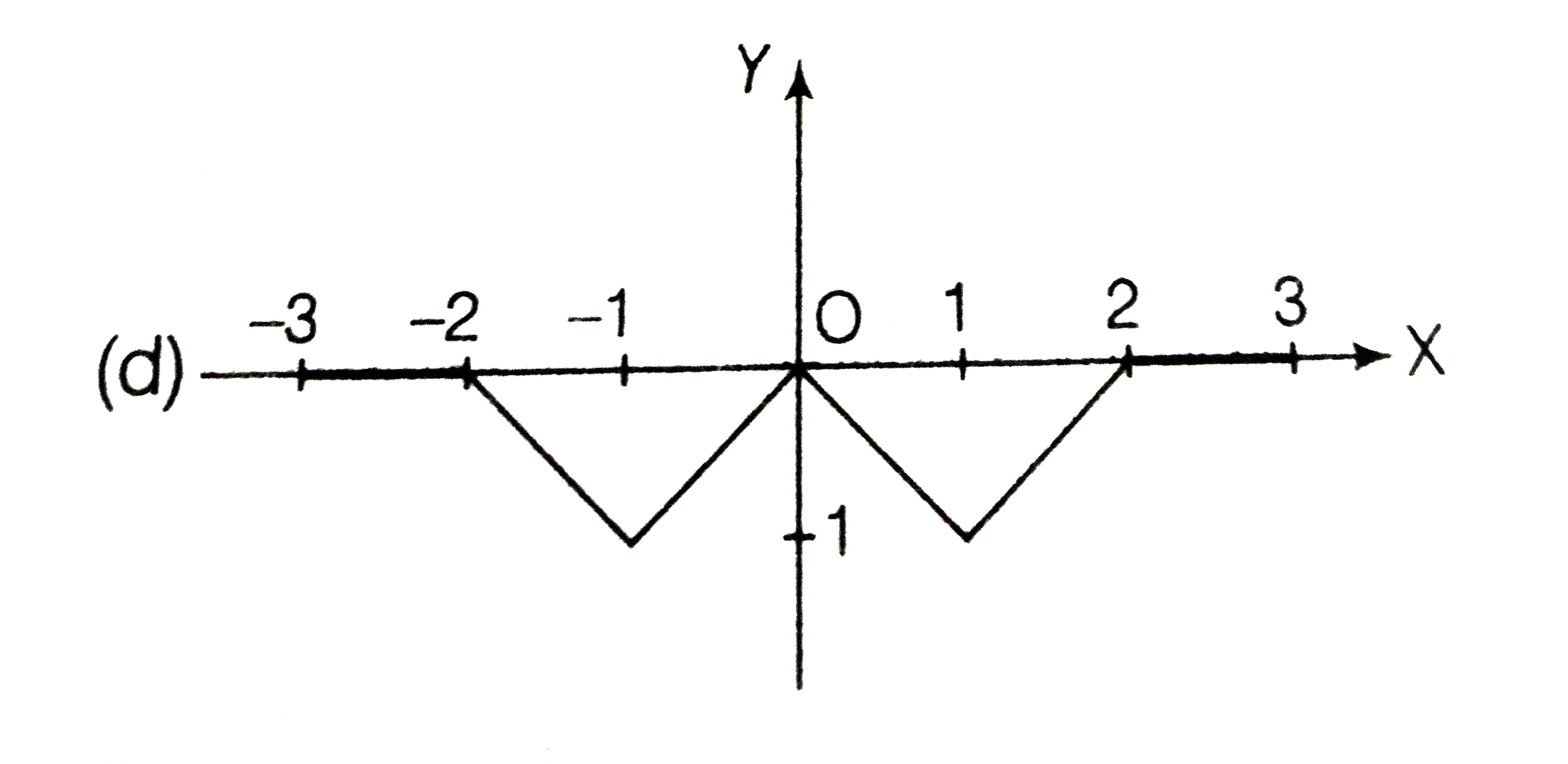
    Bocr_image
    Cocr_image
    Docr_image
  • Question 2 - Select One

    Let f:R→R be continuous function such that f(x)=f(2x) for all x∈R. If f(t)=3, then the value of ∫1−1f(f(x))dx, is

    A3f(0)
    B0
    C3f(3)
    D6
  • Question 3 - Select One

    Let f(x)={−1,−2≤x<0x2−1,0≤x≤2 and g(x)=|f(x)|+f(|x|). Then in the interval (−2,2), g is

    Adifferentiable at all points
    Bnot differentiable at two points
    Cnot continuous
    Dnot differentiable at one point