The equation of the ellipse whose focus is (1,0) and the directrix is x+ y+1=0 and eccentricity is equal to $1 / \sqrt{2}$ is

A

${(x - 1)}^{2} + y^{2} = {(x + y + 1)}^{2}$

B

$2 ∣ ∣ {(x - 1)}^{2} + Y^{2} ∣ ∣ = {(x + y + 1)}^{2}$

C

$4 {{(x - 1)}^{2} + y^{2}} = {(x + y + 1)}^{2}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Let S(1,0) be the focus and $Z Z'$ be the directrix let P(x,y) be any point on the ellipse and PM be perpendicular from P on directrix . Then by definition , we have .
$S P = e P M,$ where $e = 1 / \sqrt{2}$
$\Rightarrow S P^{2} = e^{2} P M^{2}$
$\Rightarrow {(x - 1)}^{2} + {(y - 0)}^{2} = {(\frac{1}{\sqrt{2}})}^{2} {\frac{x + y + 1}{\sqrt{1 + 1}}}^{2}$
$\Rightarrow 4 {{(x - 1)}^{2} + y^{2}} = {(x + y + 1)}^{2}$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

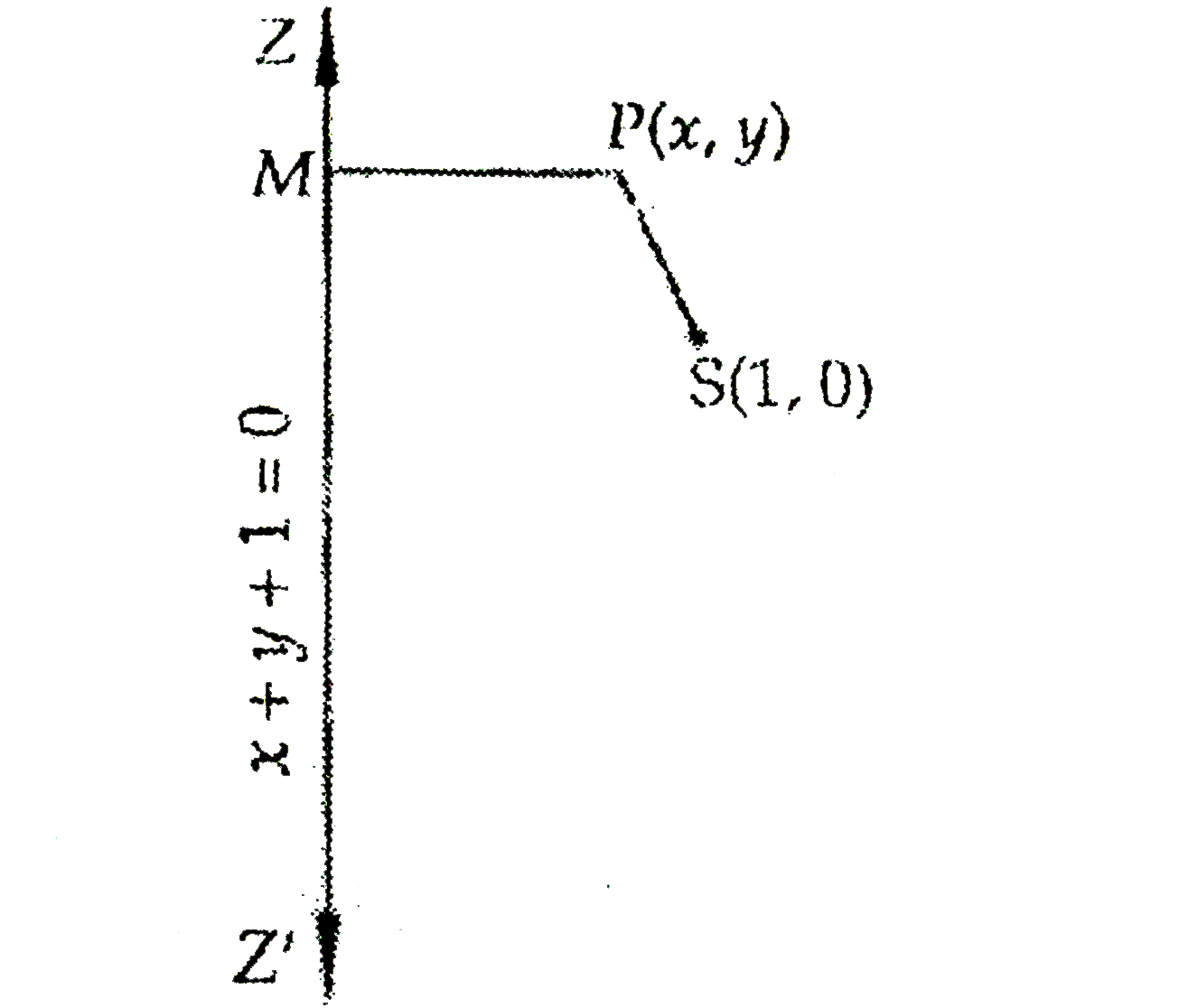
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The equation of the ellipse whose focus is (1,-1), directrix $x - y - 3 = 0$ and eccentricity equals $\frac{1}{2}$ is :
A $7 x^{2} + 2 x y + 7 y^{2} - 10 x + 10 y + 7 = 0$
B $7 x^{2} + 2 x y + 7 y^{2} + 7 = 0$
C $7 x^{2} + 2 x y + 7 y^{2} + 10 x - 10 y - 7 = 0$
D $7 x^{2} + 2 x y + 7 y^{2} + 10 x - 10 y - 7 = 0$
Question 2 - Select One
If question of the ellipse whose focus is $(1, - 1),$ then directrix the line $x - y - 3 = 0$ and eccentricity $\frac{1}{2}$ is
A $7 x^{2} + 2 x y + 7 y^{2} - 10 x + 10 y + 7 = 0$
B $7 x^{2} + 2 x y + 7 y^{2} + 7 = 0$
C $7 x^{2} + 2 x y + 7 y^{2} + 10 x - 10 y - 7 = 0$
D $none of these$
Question 3 - Select One
The equation of an ellipse whose focus is $(- 1, 1),$ directrix is $x - y + 3 = 0$ and eccentricity is $\frac{1}{2},$ is given by
A $7 x^{2} + 2 x y + 7 y^{2} + 10 x - 10 y + 7 = 0$
B $7 x^{2} - 2 x y + 7 y^{2} - 10 x + 19 y + 7 = 0$
C $7 x^{2} - 2 x y + 7 y^{2} - 10 x - 10 y - 7 = 0$
D $7 x^{2} - 2 x y + 7 y^{2} + 10 x 10 y - 7 = 0$

OBJECTIVE RD SHARMA-ELLIPSE-Chapter Test

Home
Ask Doubt
Profile