ABCD is a rhombus in the Argand plane. If the affixes of the vertices are z1,z2,z3 and z4 respectively, and ∠CBA=π/3, then
z1+ωz2+ω2z3=0
z1−ωz2−ω2z3=0
ωz1+z2+ω2z3=0
ω2z1+ωz2+z3=0
The correct Answer is:A
Since the diagonala of a rhombus bisect each other.
Therefore, BC=BA. Also ∠CBA=π3.
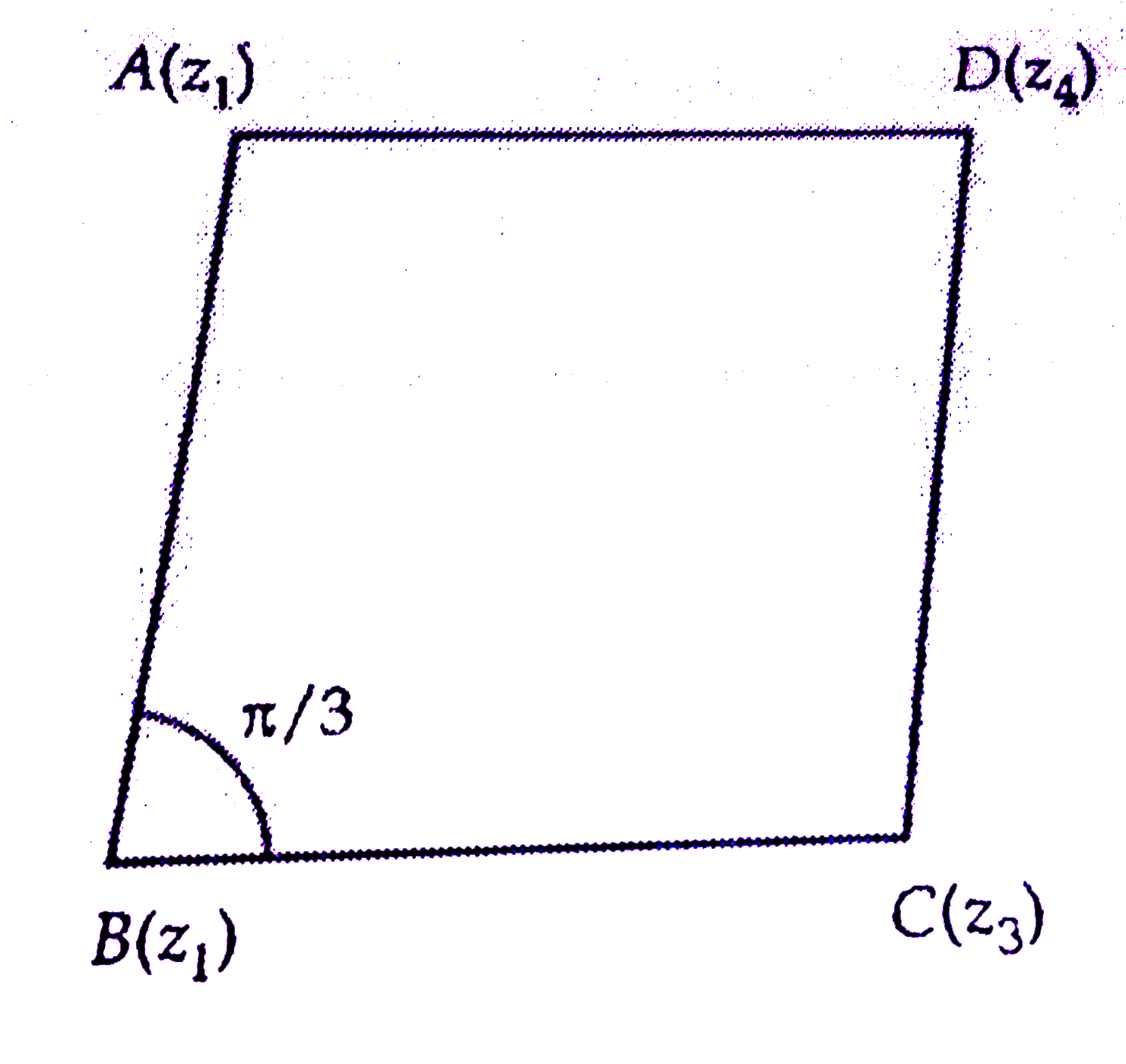
∴¯¯¯¯¯¯BA is obtained by rotating ¯¯¯¯¯¯BC through π/3.
⇒¯¯¯¯¯¯BA=¯¯¯¯¯¯BCeiπ/3.
⇒(z1−z2)=(z3−z2)(cosπ/3)+isinπ/3)
⇒(z1−z2)=(z3−z2)(−ω2)[∴ω2=−12−i√32]
z1−z2(1+ω2)+z3ω2=0 [∴1+ω2=−ω]
Topper's Solved these Questions
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section I - Solved Mcqs
141 Videos
- COMPLEX NUMBERS BOOK - OBJECTIVE RD SHARMACHAPTER - COMPLEX NUMBERS EXERCISE - Section II - Assertion Reason Type
15 Videos
- CIRCLESBOOK - OBJECTIVE RD SHARMACHAPTER - CIRCLESEXERCISE - Chapter Test
55 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - OBJECTIVE RD SHARMACHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise
86 Videos
Similar Questions
ABCD is a rhombus in the argand plane. If the affixes of the vertices are z1,z2,z3,z4 respectively and ∠CBA=π3 then find the value of z1+ωz2+ω2z3 where omega is a complex cube root of the unity
View SolutionABCD is a rhombus in the Argand plane. If the affixes of the vertices be z1,z2,z3,z4 and taken in anti-clockwise sense and △CBA=π3, show that
View Solution
Knowledge Check
Let z1,z2,z3 be three complex numbers satisfying 1z1+1z2+1z3=0. Let zk=rk(cosαk+isinαk) and ωk=cos2αk+isin2αkzk for k=1,2,3. If ω1,ω2 and ω3 are the affixes of points A1,A2 and A3 respectively in the Argand plane, then ΔA1A2A3 has its
Aincenter at the originBcentroid at the originCcircumcenter at the originDorthocenter at the originz1,z2,z3andz4 are the affixes of four points in the argand plane and z affix of a point such that |z−z1|=|z−z2|=|z−z3| then =|z−z4|, z1,z2,z3andz4 are
AconcyclicBtices of parallelogramCvertices of rhombusDin a straight lineIf the points z1,z2,z3 are the vertices of an equilateral triangle in the Argand plane, then which one of the following is not correct?
A1z1−z2+1z2−z3+1z3−z1=0Bz21+z22+z23=z1z2+z2z3+z3z1C(z1−z2)2+(z2−z3)2+(z3−z1)2=0Dz31+z32+z33+3z1z2z3=0
The vertices of a square are z1,z2,z3andz4 taken in the anticlockwise order, then z3=
View SolutionIf z1,z2,z3,z4 are the affixes of four point in the Argand plane, z is the affix of a point such that |z−z1|=|z−z2|=|z−z3|=|z−z4| , then z1,z2,z3,z4 are
View SolutionIf z1,z2andz3 are the vertices of a right angledtriangle in Argand plane such that |z1−z2|=3,|z1−z3|=5andz2 is the vertex with the right angle then
View SolutionGiven that the complex numbers which satisfy the equation ∣∣zz3∣∣+∣∣zz3∣∣=350 form a rectangle in the Argand plane with the length of its diagonal having an integral number of units, then area of rectangle is 48 sq. units if z1,z2,z3,z4 are vertices of rectangle, then z1+z2+z3+z4=0 rectangle is symmetrical about the real axis arg(z1−z3)=π4or3π4
View SolutionLet z1 be a fixed point on the circle of radius 1 centered at the origin the Argand plane and z1≠±1 .Consider an equilaternal triangle inscribed in the circle with z1,z2,z3 as the vertices taken in the counter clockwise direction. Then z1z2z3 is equal to
View Solution
