A compound made of particles A, B, and C forms p lattice. Ions A are at lattice points, B occupy TVsC occupy OVs. If all the ions along one of the edge axis are removed, then formula of the compound is
A3.75B8C3.75
A3.75B4C8
A4B8C3.75
A4B3.75C8
The correct Answer is:A
Since lattice is p,Zeff=4.
∴ Number of A ions =4
(corner + face centre =1+3=4)
Number of B ions = Number of TVs=8
Number of C ions = Number of OVs=4
2TVs are founded at each diagonal of cube.
4TVs are founded on edge centres and body of centre.
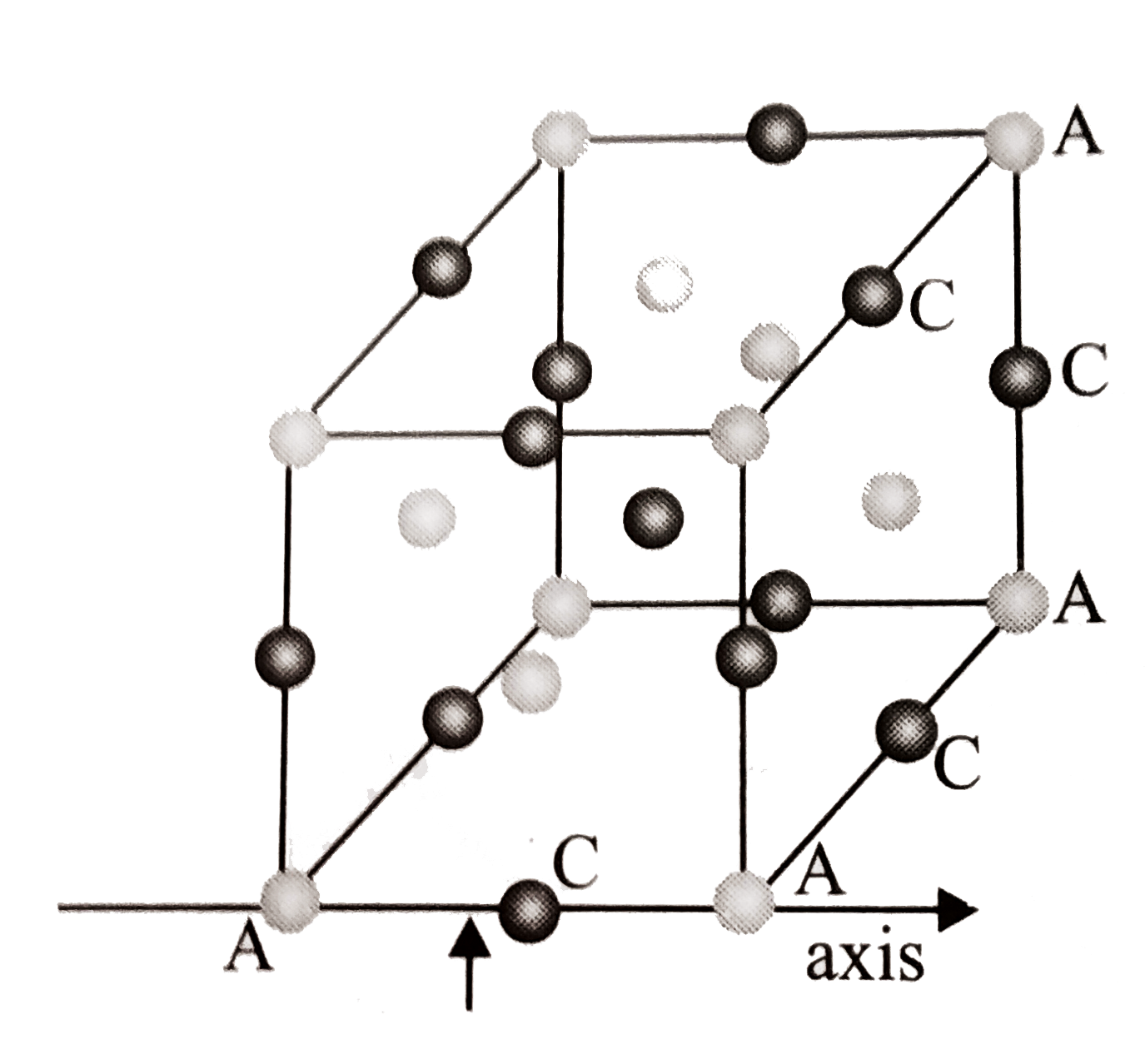
2A ions (at corners) and one C ion (at edge centre are removed.
Number of A ions removed =2×18 (corner share)
=14
Number of C ions removed =1×14 (edge centre share)
=14
Number of A ions left =4−14=3.75
Number of B ions =8
(Since no B ion has been removed)
Number of C ions left =4−14=3.75
Thus, formula is: A3.75B8C3.75
Topper's Solved these Questions
- SOLID STATEBOOK - CENGAGE CHEMISTRYCHAPTER - SOLID STATEEXERCISE - Solved Examples
13 Videos
- SOLID STATEBOOK - CENGAGE CHEMISTRYCHAPTER - SOLID STATEEXERCISE - Exercises (Linked Comprehension)
13 Videos
- REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSBOOK - CENGAGE CHEMISTRYCHAPTER - REDUCTION AND OXIDATION REACTION OF ORGANIC COMPOUNDSEXERCISE - SUBJECTIVE TYPE
3 Videos
- SOLUTIONSBOOK - CENGAGE CHEMISTRYCHAPTER - SOLUTIONSEXERCISE - Ex 2.3 (Objective)
9 Videos
Similar Questions
A solid AB forms rock salt structure in which anions B− forms the lattice. If all particles along one of the tetrad axis is removed the formula of resulting compound is
View SolutionA compound formed by elements A and B crystallises as FCC lattice. If A is present at the lattice points of unit cell and B occupy half of tetrahedral voids as well as one fourth of octahedral voids then the formula of the compound will be
View Solution
Knowledge Check
A compound made of particles A, B, and C forms p lattice. In the lattice, ions A occupy the lattice points and ions B and C occuphy the alternate TVs. If all the ions along one of the body diagonals are removed, then formula of the compound is
AA3.75B3C3BA3.75B3C4CA3B3.75C3DA3B3C3.75A compound made of particles A and B. A forms fcc packing and B occupies all the OVs. If all the particles along the plane as shown in the figure below are removeed, then the simplest formula of the compound is
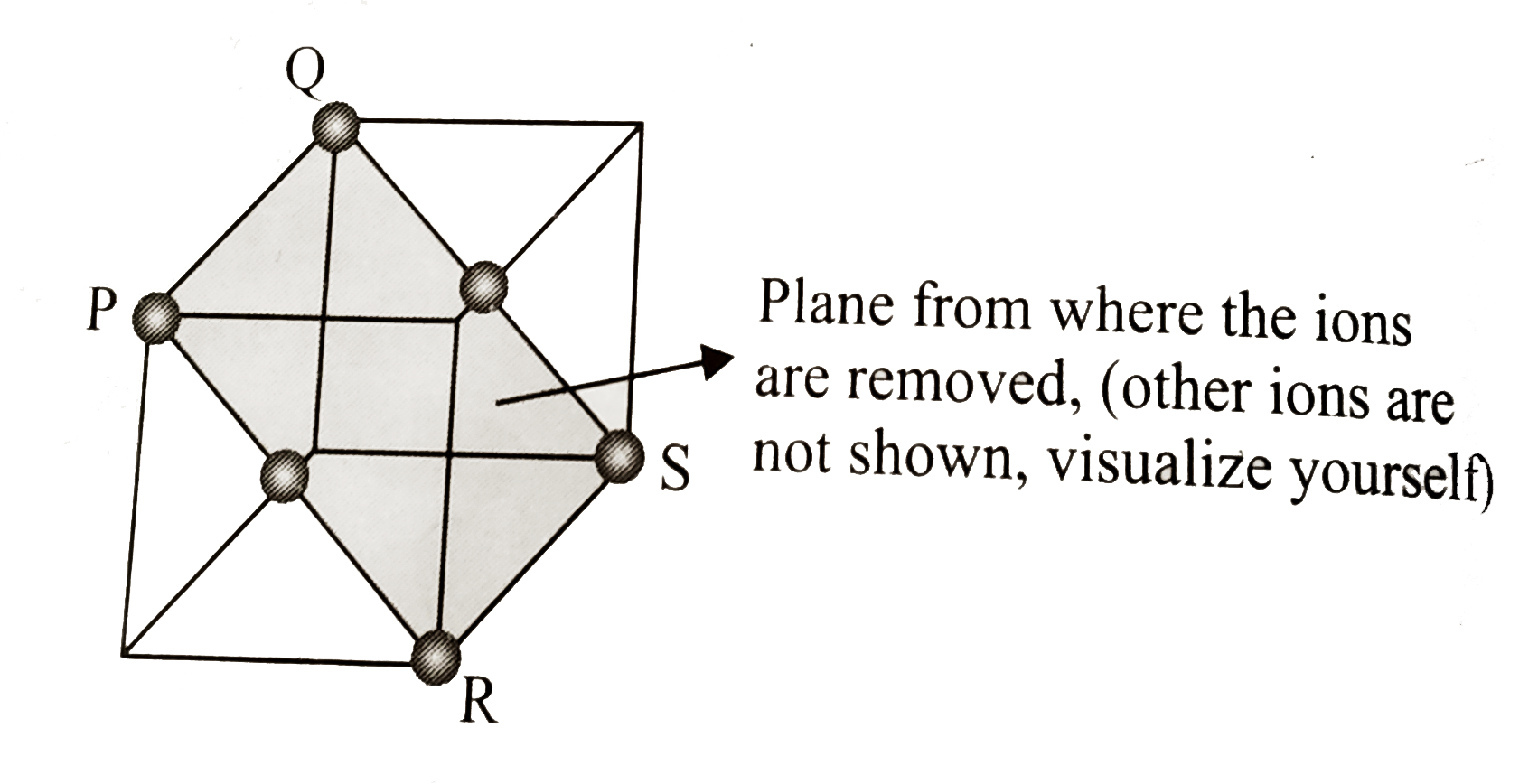 AA5B7BA7B5CABDAB3.75
AA5B7BA7B5CABDAB3.75A crystal is made of particles A and B. A forms FCC packing and B occupies all the octahedral voids. If all the particles along the plane as shown in figure are removed, then the formula of the crystal would be :
AA5B7BA7B5CABDAB3.75
A compound of A and B crystallizes in a cubic lattice in which A atoms occupy the lattice points at the corners of a cube and two atoms of B occupy the center of each of the cube faces. What is the formula of this compound?
View SolutionA crystal is made up of particles A. B and C. A forms fcc packing, B occupies all octahedral voids and C occupies all tetrahedral voids. If all the particles along one body diagonal are removed, then the formula of the crystal would be
View SolutionA2B has antifluorite structure (B forms FCC lattice and A occupies tetrahedral voids).If all ions along any one body diagonal are removed, then new formula of compound will be :
View SolutionA crystal is made of particles A and B. A forms FCC packing and B occupies all the octahedral voids. If all the particles along the plane as shown in figure are removed, then the formula of the crystal would be :
View SolutionA body centred cubic lattice is composed of anions Q and cations P, where ions occupy the corners and ions P occupy the centre, the formula of the compound is
View Solution
