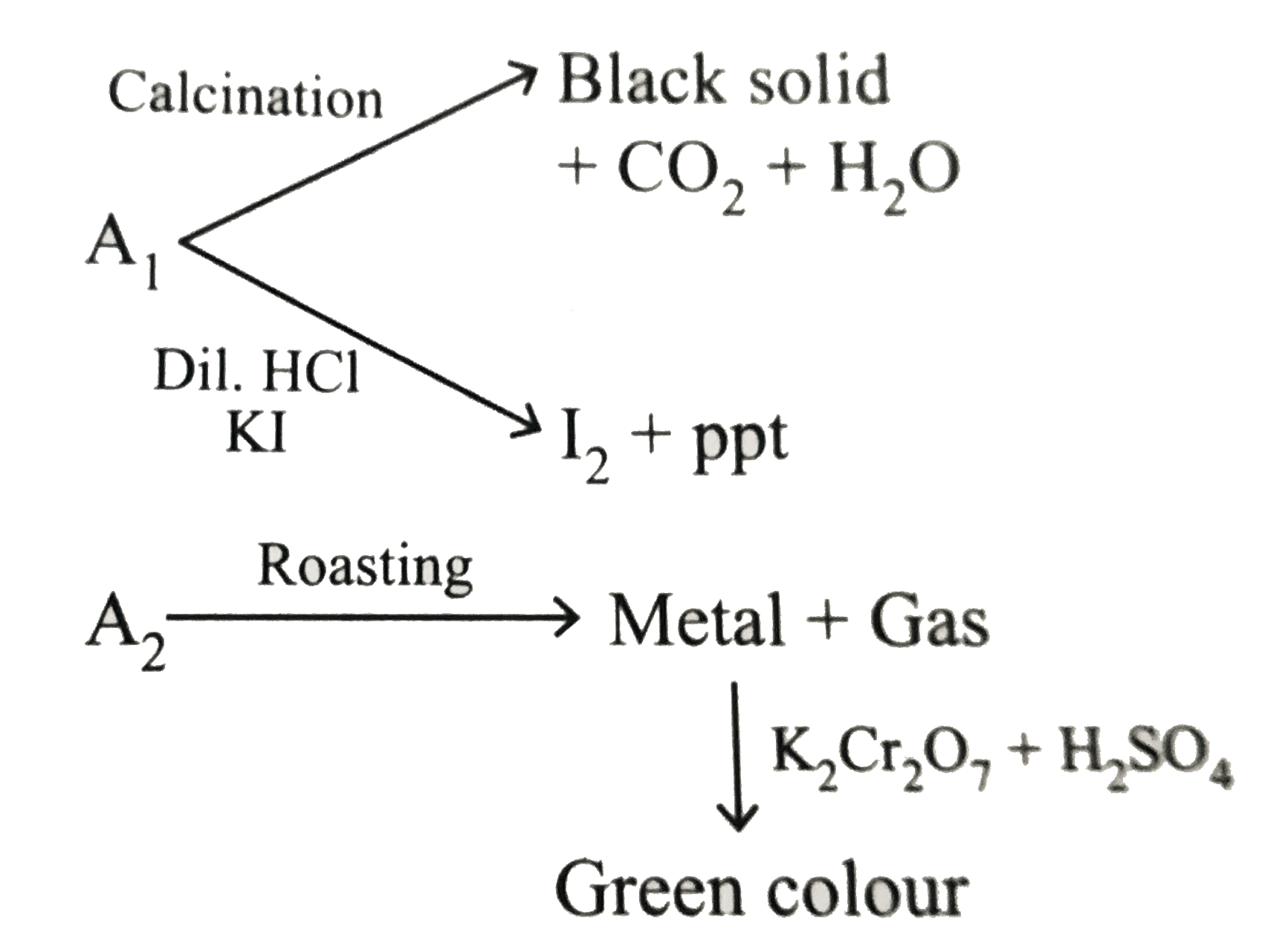
$A_{1}$ and $A_{2}$ are two ores of metal $M . A_{1}$ on calcination gives black precipitate, $C O_{2}$ and water. Identify $A_{1}$ and $A_{2}$ .

Text Solution

Verified by Experts

$A_{1}$ is malachite $C u C O_{3} . C u {(O H)}_{3}$
$C u C O_{3} C u {(O H)}_{2} Δ - - \to C u O + C O_{2} ↑ ⏐ ⏐ + H_{2} O$
$C u O$ is the black solid.
$C u C O_{3} . C u {(O H)}_{2} d i l . H C l - --- \to C u C l_{2} + C O_{2} + H_{2} O$
$C u C l_{2} K I - - \to C u_{2} I_{2} + K C l + I_{2}$
$A_{2}$ is copper glance $C u_{2} S$ , a sulphide ore.
$C u_{2} S O_{2} - - \to Δ C u_{2} O + S O_{2}$
$C u_{2} S + C u_{2} O \to C u + S O_{2}$
The gas $S O_{2}$ gives green colour with acidified $K_{2} C r_{2} O_{7} . K_{2} C r_{2} O_{7} + H_{2} S O_{4} + 3 S O_{4} \to K_{2} S O_{4} + C r_{2} {(S O_{4})}_{3} + 4 H_{2} O$

Updated on:21/07/2023