If the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
To solve the problem of finding the ratio of the area of a circle A1 to the area of a regular pentagon A2 inscribed in the circle, we will follow these steps:
Step 1: Area of the Circle
The area of the circle is given by the formula:
A1=πr2
where r is the radius of the circle.
Step 2: Area of the Regular Pentagon
To find the area of the regular pentagon inscribed in the circle, we need to calculate the area of one of the triangles formed by the center of the circle and two adjacent vertices of the pentagon.
Each triangle (e.g., triangle OAB) has a central angle of:
θ=360∘5=72∘
Step 3: Area of One Triangle
The area Atriangle of triangle OAB can be calculated using the formula:
Atriangle=12r2sin(θ)
Substituting θ=72∘:
Atriangle=12r2sin(72∘)
Step 4: Area of the Pentagon
Since the pentagon consists of 5 such triangles, the total area A2 of the pentagon is:
A2=5×Atriangle=5×12r2sin(72∘)=52r2sin(72∘)
Step 5: Ratio of Areas
Now, we can find the ratio A1A2:
A1A2=πr252r2sin(72∘)
The r2 terms cancel out:
A1A2=π52sin(72∘)=2π5sin(72∘)
Step 6: Final Result
Thus, the ratio of the area of the circle to the area of the pentagon is:
A1A2=2π5sin(72∘)
The area of the circle is given by the formula:
A1=πr2
where r is the radius of the circle.
To find the area of the regular pentagon inscribed in the circle, we need to calculate the area of one of the triangles formed by the center of the circle and two adjacent vertices of the pentagon.
θ=360∘5=72∘
The area Atriangle of triangle OAB can be calculated using the formula:
Atriangle=12r2sin(θ)
Substituting θ=72∘:
Atriangle=12r2sin(72∘)
Since the pentagon consists of 5 such triangles, the total area A2 of the pentagon is:
A2=5×Atriangle=5×12r2sin(72∘)=52r2sin(72∘)
Now, we can find the ratio A1A2:
A1A2=πr252r2sin(72∘)
The r2 terms cancel out:
A1A2=π52sin(72∘)=2π5sin(72∘)
Thus, the ratio of the area of the circle to the area of the pentagon is:
A1A2=2π5sin(72∘)
Topper's Solved these Questions
Similar Questions
Knowledge Check
A body A starts from rest with an acceleration a1. After 2 seconds, another body B starts from rest with an acceleration a2. If they travel equal distances in the 5th second, after the start of A, then the ratio a1:a2 is equal to :
A0.21458333333333B0.21319444444444C0.37847222222222D0.37986111111111
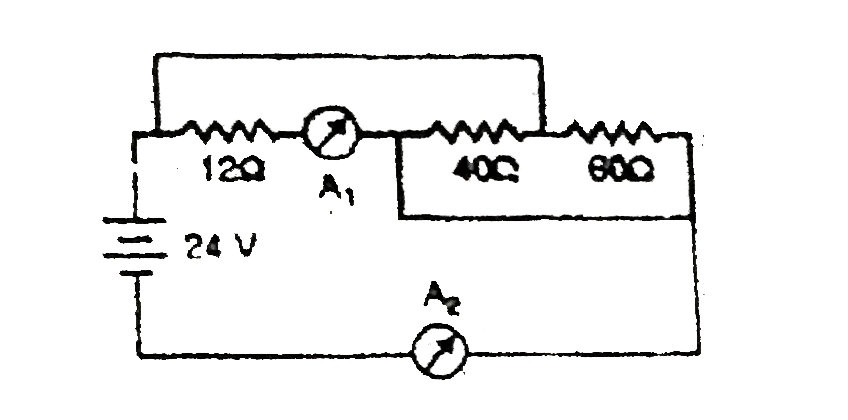
In the circuit of figure A1 and A2 are ideal ammeters. Then the reading in ammeter A2 is
View SolutionA circle is inscribed inside a regular pentagon and another circle is circumscribed about this pentagon.Similarly a circle is inscribed in a regular heptagon and another circumscribed about the heptagon. The area of the regions between the two circles in two cases are A1andA2, respectively. If each polygon has a side length of 2 units then which one of the following is true?
View SolutionIf A,A1,A2 and A3 are the areas of the inscribed and escribed circles of a triangle, prove that 1√A=1√A1+1√A2+1√A3
View SolutionIf A1 is the area of the parabola y2=4ax lying between vertex and the latusrectum and A2 is the area between the latusrectum and the double ordinate x=2a, then A1A2 is equal to
View SolutionThe capacitance of the capacitors of plate areas A1 and A2(A1<A2) at a distance d is

View SolutionThe capacitance of capacitor of plate areas A1 and A2 (A1<A2 at a distance d is

View SolutionLet Ar,r=1,2,3, , be the points on the number line such that OA1,OA2,OA3. are in GP, where O is the origin, and the common ratio of the GP be a positive proper fraction. Let M, be the middle point of the line segment ArAr+1. Then the value of ∞∑r=1OMr is equal to
(a) OA1(OSA1−OA2)2(OA1+OA2) (b) OA1(OA2+OA1)2(OA1−OA2) (c)OA12(OA1−OA2) (d) ∞
View Solution
