For a reaction , the concentration of the reactant C varies with time t as shown by the following graph .
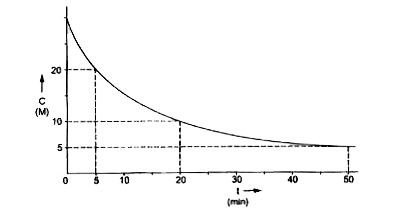
what do you conclude about the order of the reaction ?
The chance in concentration of the reactant from 20 M to 10 M takes 15 minutes and when it changes from 10 M to 5 M , it takes 30 minutes .So , for the given reaction ,t12 in inversely proportional to the initial concentration of the reactant suggesting that the reaction is of second order .
Topper's Solved these Questions
- BOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - PROBLEMS42videos
CHEMICAL KINETICS
- BOOK - RC MUKHERJEECHAPTER - CHEMICAL KINETICSEXERCISE - OBJECTIVE PROBLEMS51videos
CHEMICAL KINETICS
- BOOK - RC MUKHERJEECHAPTER - CHEMICAL EQUIVALENCEEXERCISE - PROBLEMS24videos
CHEMICAL EQUIVALENCE
- BOOK - RC MUKHERJEECHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Objective problems58videos
CHEMICAL THERMODYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
For a first order reaction, the concentration of reactant :
Ais independent of timeBvaries linearly with timeCvaries exponentially with timeDNone - Question 2 - Select One
In a 1st order reaction, reactant concentration C varies with time t as:
A1/C increases linearly with tBlog C decreases linearly with tCC decreases with 1/tDlog C decreases with 1/t - Question 3 - Select One
In a 1st order reaction, reactant concentration C varies with time t as:
A1/C increases linearly with tBlog C decreases linearly with tCC decreases with 1/tDlog C decreases with 1/t
In the reaction, P+Q→R+S
the time taken for 75% reaction of P is twice the time taken for 50% reaction of P. The concentration of Q varies with reaction time as shown in the figure. The overall order of the reaction is
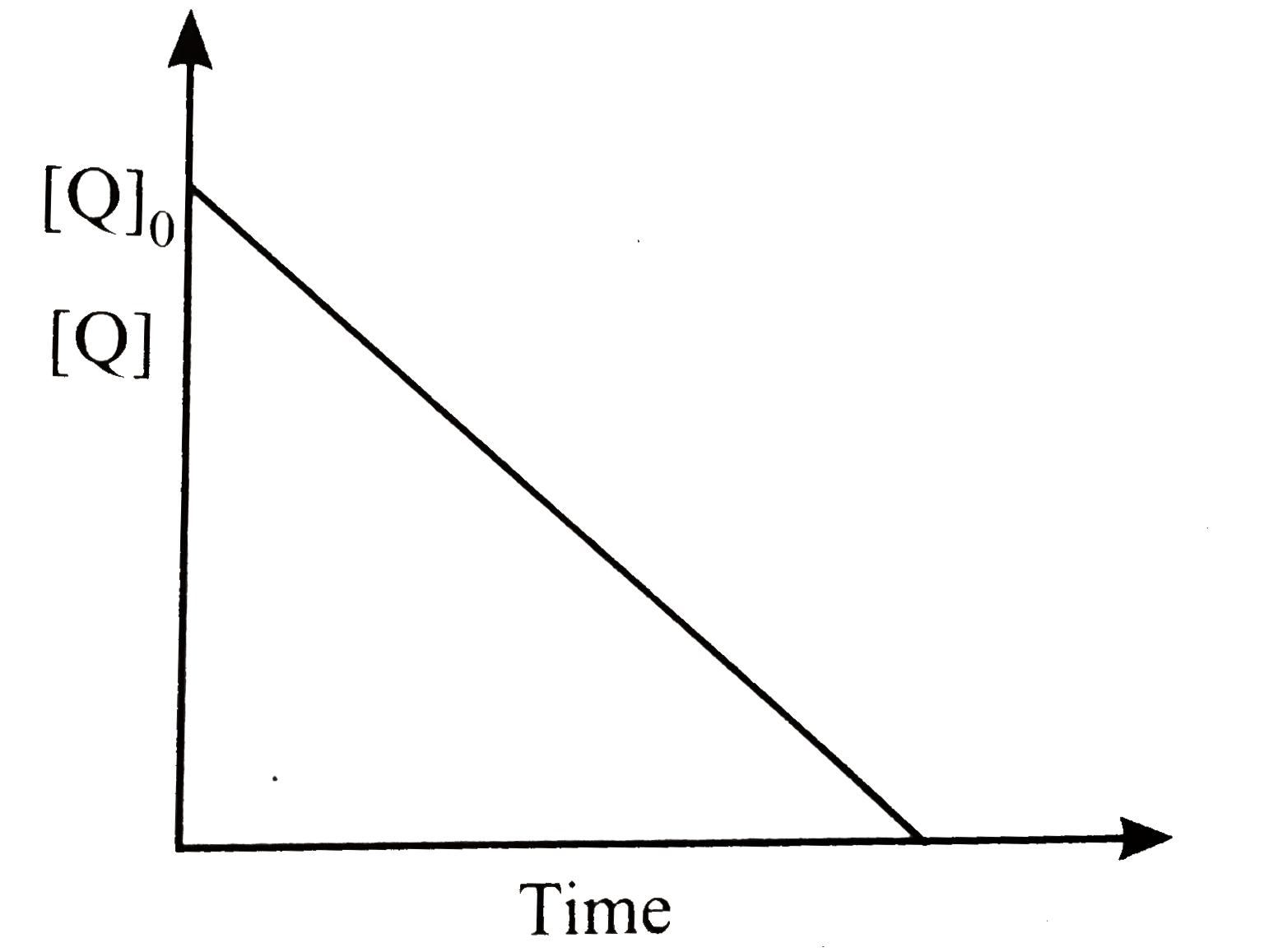 View Solution
View SolutionIn a first order reaction with time the concentration of the reactant decreases
View SolutionIn a first order reaction fraction of the total concentration of the reactant varies with time 't' is equal to
View SolutionAssertion (A) : For a first order, the concentration of a reaction decreases exponentially with time.
Reason (R ): The rate of reaction at any time depends upon the concentration of the reactant at that time.View SolutionAssertion: For a first order reaction, the concentration of the reactant decreases exponentially with time.
Reason: Rate of reaction at any time depend upon the concentration of reactant at that time.View Solution

