Give the names of seven f-orbitals and how they are represented.
To answer the question regarding the names of the seven f-orbitals and their representations, we can follow these steps:
1. Identify the Azimuthal Quantum Number (L):
- For f-orbitals, the azimuthal quantum number (L) is equal to 3. This is a fundamental property of f-orbitals.
2. Determine the Total Number of f-orbitals:
- The formula to calculate the total number of orbitals for a given value of L is \(2L + 1\).
- Substituting L = 3 into the formula gives us:
\(
2(3) + 1 = 6 + 1 = 7
\)
- Therefore, there are 7 f-orbitals.
3. List the Names of the Seven f-orbitals:
- The names of the seven f-orbitals are:
1. \(f_{xyz}\)
2. \(f_{x^3}\)
3. \(f_{y^3}\)
4. \(f_{z^3}\)
5. \(f_{x^2y}\)
6. \(f_{x^2z}\)
7. \(f_{y^2z}\)
4. Representation of the f-orbitals:
- The representation of these orbitals typically involves their mathematical functions or shapes, which can be visualized in diagrams. However, in a textual format, they are represented as shown above.
5. Conditions for the Existence of f-orbitals:
- The principal quantum number (n) must be greater than or equal to 4 for f-orbitals to exist. This means that f-orbitals are found in the fourth energy level and beyond.
Final Answer:
The names of the seven f-orbitals are:
1. \(f_{xyz}\)
2. \(f_{x^3}\)
3. \(f_{y^3}\)
4. \(f_{z^3}\)
5. \(f_{x^2y}\)
6. \(f_{x^2z}\)
7. \(f_{y^2z}\)
These orbitals are represented by their respective mathematical notations as listed above, and they exist when the principal quantum number \(n \geq 4\).
- For f-orbitals, the azimuthal quantum number (L) is equal to 3. This is a fundamental property of f-orbitals.
- The formula to calculate the total number of orbitals for a given value of L is \(2L + 1\).
- Substituting L = 3 into the formula gives us:
\(
2(3) + 1 = 6 + 1 = 7
\)
- Therefore, there are 7 f-orbitals.
- The names of the seven f-orbitals are:
1. \(f_{xyz}\)
2. \(f_{x^3}\)
3. \(f_{y^3}\)
4. \(f_{z^3}\)
5. \(f_{x^2y}\)
6. \(f_{x^2z}\)
7. \(f_{y^2z}\)
- The representation of these orbitals typically involves their mathematical functions or shapes, which can be visualized in diagrams. However, in a textual format, they are represented as shown above.
- The principal quantum number (n) must be greater than or equal to 4 for f-orbitals to exist. This means that f-orbitals are found in the fourth energy level and beyond.
The names of the seven f-orbitals are:
1. \(f_{xyz}\)
2. \(f_{x^3}\)
3. \(f_{y^3}\)
4. \(f_{z^3}\)
5. \(f_{x^2y}\)
6. \(f_{x^2z}\)
7. \(f_{y^2z}\)
Topper's Solved these Questions
- PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYEXERCISE - Ex 1.1
14 Videos
- PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYEXERCISE - Ex 1.1 (Very Short)
22 Videos
- PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - PERIODIC CLASSIFICATION OF ELEMENTS AND GENERAL INORGANIC CHEMISTRYEXERCISE - Exercises (Archives )Subjective
4 Videos
- P-BLOCK GROUP 14 - CARBON FAMILYBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - P-BLOCK GROUP 14 - CARBON FAMILYEXERCISE - Exercises Archives (Subjective)
9 Videos
- PURIFICATION OF ORGANIC COMPOUNDS AND QUALITATIVE AND QUANTITATIVE ANALYSISBOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - PURIFICATION OF ORGANIC COMPOUNDS AND QUALITATIVE AND QUANTITATIVE ANALYSISEXERCISE - Assertion Reasoning Type
5 Videos
Similar Questions
Give the IUPAC name of :
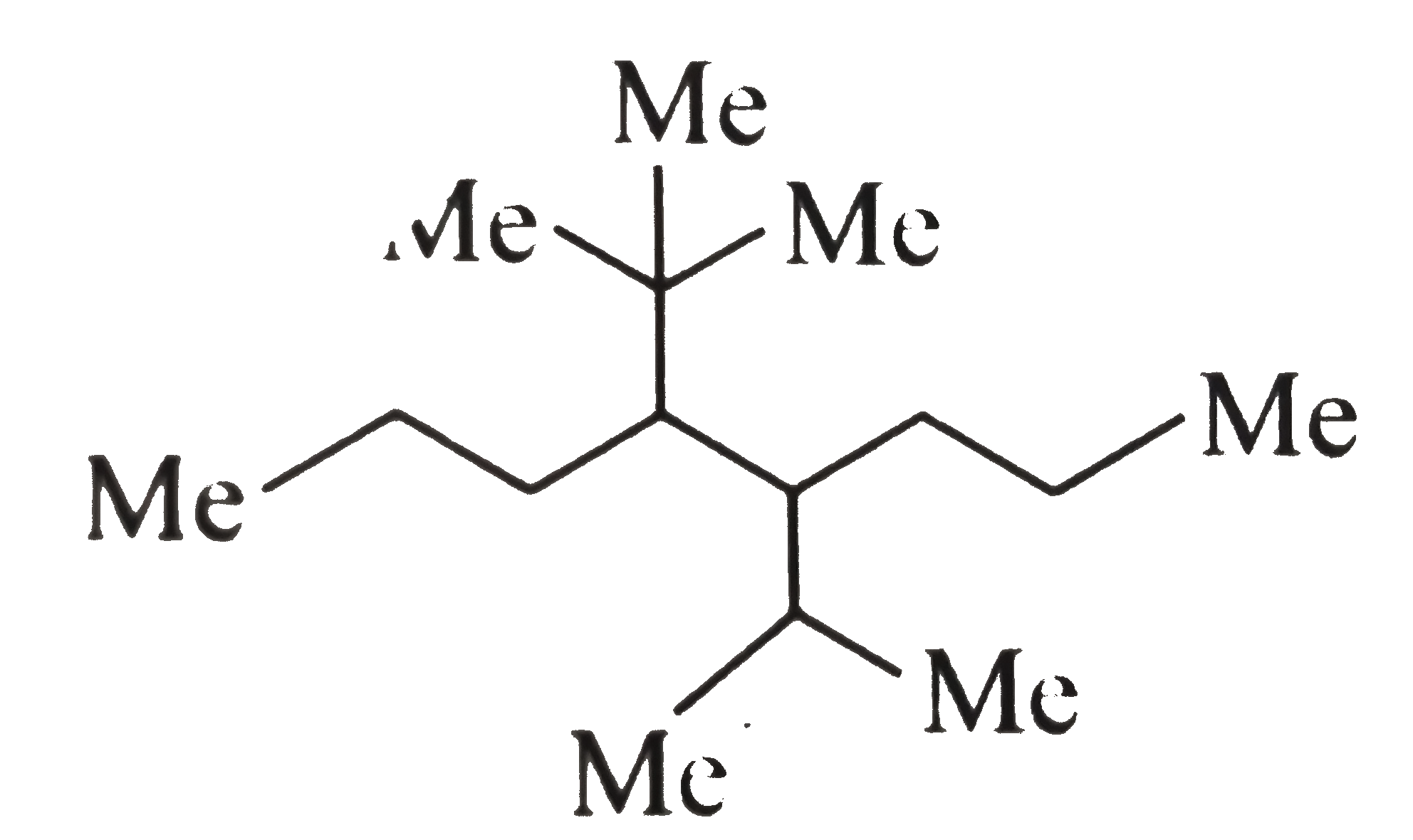
View SolutionGive the name of the KClO ?
View SolutionA 4f orbital has
View SolutionGive the IUPAC name
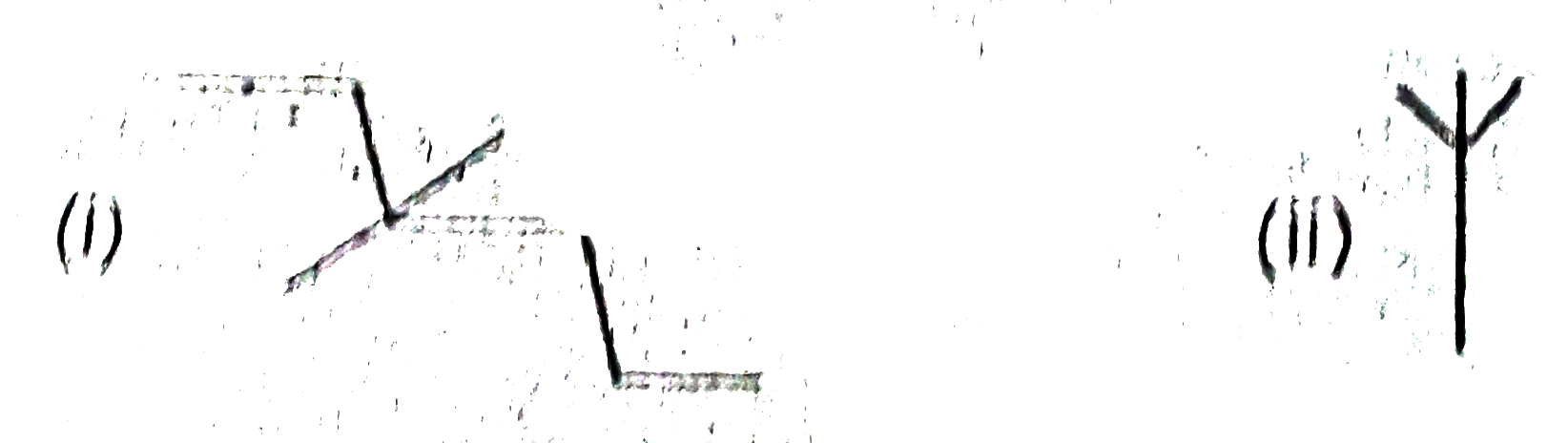
View SolutionGive IUPAC name of:
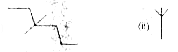
View SolutionGive the name of the KClO4 ?
View SolutionGive the name of the KClO2 ?
View SolutionGive the name of the KClO3 ?
View SolutionGive the name of the first antibiotic.
View SolutionGive the IUPAC names of the following :
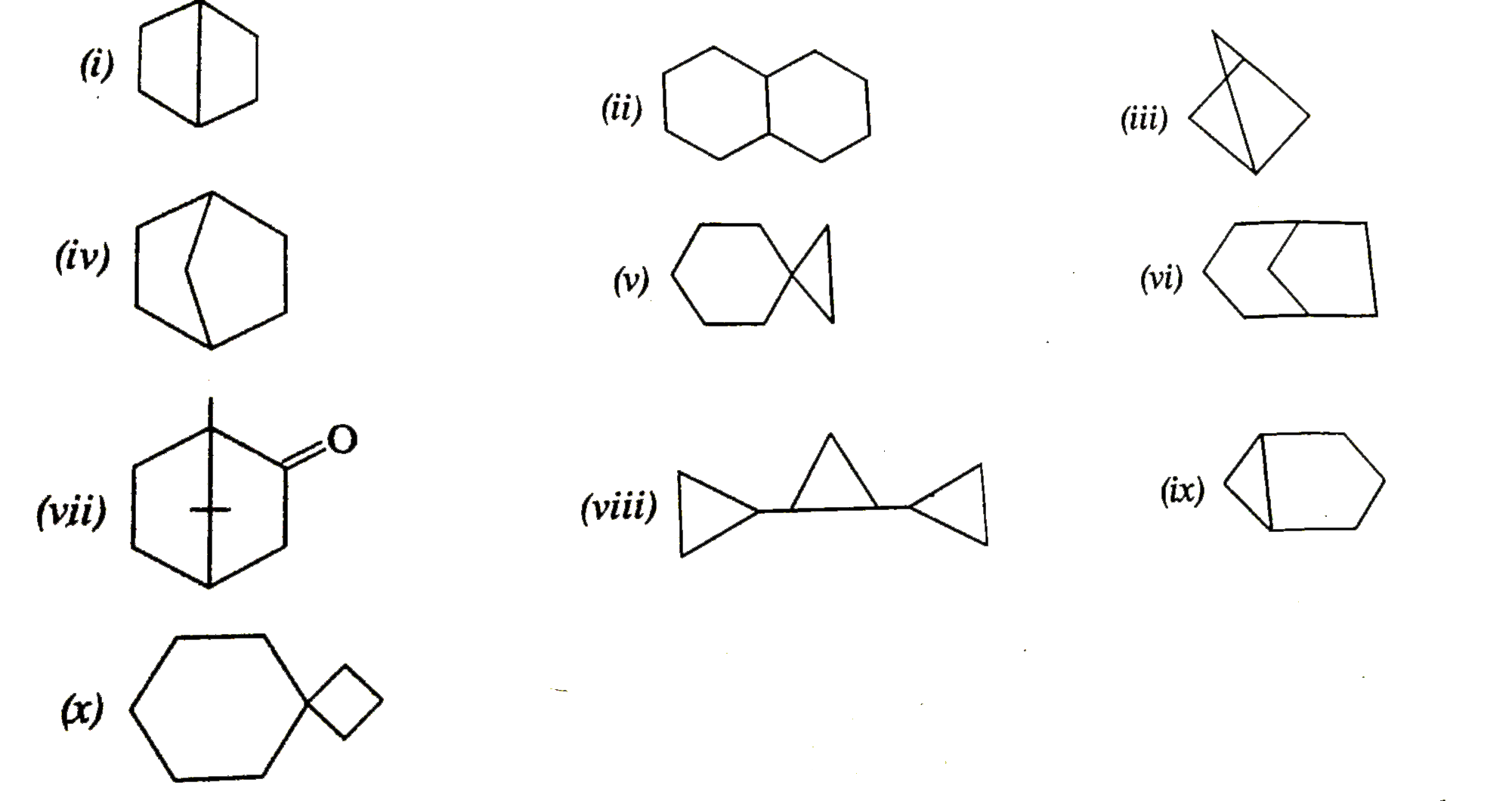
View Solution
