How many moles of helium are produced when 1 mole of .92U238 disintegrate into .82Pb206?
To solve the problem of how many moles of helium are produced when 1 mole of Uranium-238 disintegrates into Lead-206, we can follow these steps:
1. Understand the decay process: Uranium-238 (U-238) undergoes alpha decay. In this process, it emits alpha particles, which are essentially helium nuclei (He-4).
2. Determine the change in atomic and mass numbers:
- The atomic number of U-238 is 92, and its mass number is 238.
- The atomic number of Lead-206 (Pb-206) is 82, and its mass number is 206.
- When U-238 decays to Pb-206, we need to find out how many alpha particles (helium nuclei) are emitted.
3. Calculate the change in atomic number:
- The change in atomic number from U-238 (92) to Pb-206 (82) is:
92−82=10
- This means that 10 protons are lost during the decay process.
4. Calculate the change in mass number:
- The change in mass number from U-238 (238) to Pb-206 (206) is:
238−206=32
- This means that 32 nucleons (protons + neutrons) are lost during the decay process.
5. Determine the number of alpha particles emitted:
- Each alpha particle (He-4) consists of 2 protons and 2 neutrons, which means it reduces the atomic number by 2 and the mass number by 4.
- To find out how many alpha particles are emitted, we can set up the following equations:
- For protons: 2n=10 (where n is the number of alpha particles)
- For nucleons: 4n=32
6. Solve for n:
- From the first equation:
n=102=5
- From the second equation:
n=324=8
- Both equations must be satisfied, and since we need to balance both the loss of protons and nucleons, we find that the total number of alpha particles emitted is 8.
7. Conclusion:
- Therefore, when 1 mole of U-238 disintegrates into Pb-206, it produces 8 moles of helium (He-4).
Final Answer:
8 moles of helium are produced.
- The atomic number of U-238 is 92, and its mass number is 238.
- The atomic number of Lead-206 (Pb-206) is 82, and its mass number is 206.
- When U-238 decays to Pb-206, we need to find out how many alpha particles (helium nuclei) are emitted.
- The change in atomic number from U-238 (92) to Pb-206 (82) is:
92−82=10
- This means that 10 protons are lost during the decay process.
- The change in mass number from U-238 (238) to Pb-206 (206) is:
238−206=32
- This means that 32 nucleons (protons + neutrons) are lost during the decay process.
- Each alpha particle (He-4) consists of 2 protons and 2 neutrons, which means it reduces the atomic number by 2 and the mass number by 4.
- To find out how many alpha particles are emitted, we can set up the following equations:
- For protons: 2n=10 (where n is the number of alpha particles)
- For nucleons: 4n=32
- From the first equation:
n=102=5
- From the second equation:
n=324=8
- Both equations must be satisfied, and since we need to balance both the loss of protons and nucleons, we find that the total number of alpha particles emitted is 8.
- Therefore, when 1 mole of U-238 disintegrates into Pb-206, it produces 8 moles of helium (He-4).
8 moles of helium are produced.
Topper's Solved these Questions
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Solved Example18videos
NUCLEAR CHEMISTRY
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - Ex6.1 Objective15videos
NUCLEAR CHEMISTRY
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - NCERT BASED EXERCISEEXERCISE - Nuclear Chemistry (NCERT Exercise)29videos
NCERT BASED EXERCISE
- BOOK - CENGAGE CHEMISTRY ENGLISHCHAPTER - ORGANIC COMPOUNDS WITH FUNCTIONAL GROUPEXERCISE - Archives Analytical And Descriptive24videos
ORGANIC COMPOUNDS WITH FUNCTIONAL GROUP
Similar Questions
How many moles of NO2 are produced when 1 mole of B reacts with HNO3 ?
View Solution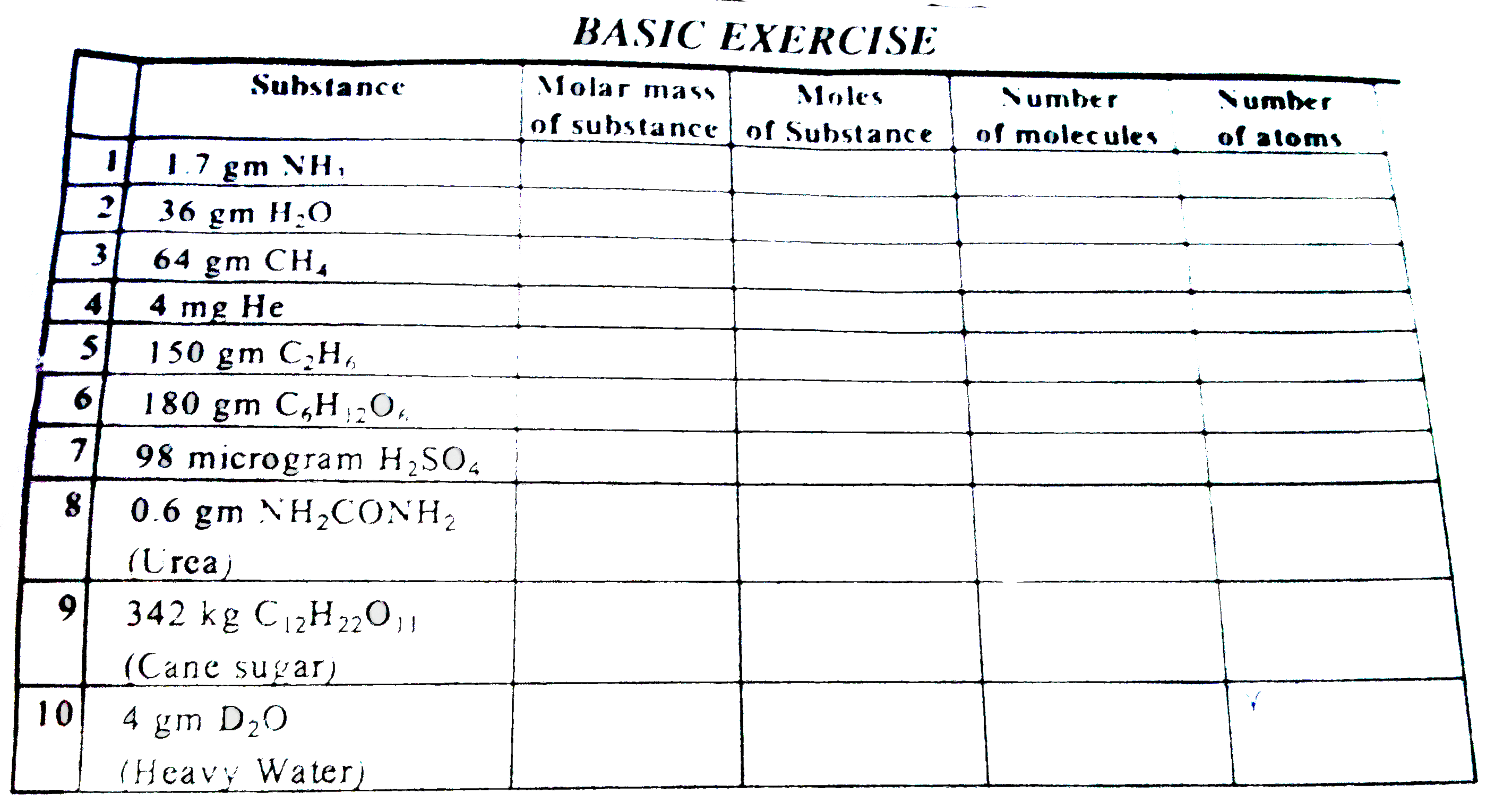
How many moles of O-atoms are there in 1 mole CaCO3View Solution
How many moles of PhNHNH2 react with 1mol(A)?View SolutionHow many moles of PbCO3 are present in whitelead ?
View SolutionHow many moles of ammonia are produced when one mole of calcium nitride reacts with water?
View SolutionHow many moles of 'HI' will react with
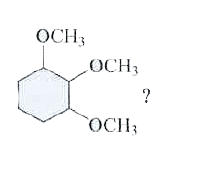 View Solution
View SolutionHow many moles of NaOH is required to neutralize 1 mole of H3PO3
View SolutionHow many moles of H2O are liberated when one mole hydrated MgCl2 is heated?
View SolutionHow many moles of H2O are liberated when one mole hydrated MgCl2 is heated?
View SolutionHow many moles of H2O are liberated when one mole hydrated MgCl2 is heated?
View Solution

