In hexogonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexongonas and three atoms are sandwiched inbetween them. A space filling model of this structure called haxagonal closed packed (HCP) is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible . Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'.
The empty space in this HCP unit cell is
0.74
0.476
0.32
0.26
The correct Answer is:c
To find the empty space in a hexagonal closed packed (HCP) unit cell, we will follow these steps:
Step 1: Understand the structure of HCP
In an HCP structure, we have:
- Two hexagonal faces (top and bottom) and three layers of atoms.
- The first layer consists of one atom at the center and six surrounding atoms, making a total of 7 atoms in the first layer.
- The second layer consists of three atoms positioned in the gaps of the first layer.
- The third layer is identical to the first layer.
Step 2: Calculate the volume of the unit cell
The volume of the unit cell can be calculated using the formula for the volume of a hexagonal prism:
Vcell=3√32a2c
Where:
- a is the distance between the centers of two adjacent spheres in the same layer (which is 2r).
- c is the height of the unit cell, which is 4r√2 for HCP.
Substituting these values:
Vcell=3√32(2r)2(4r√2)
=3√32⋅4r2⋅4r√2
=3√3⋅16r32√2=24√3r3
Step 3: Calculate the volume occupied by the atoms
In HCP, there are 6 atoms in the unit cell (1 from the top face, 1 from the bottom face, and 4 from the three layers). The volume occupied by the atoms is given by:
Vatoms=n⋅43πr3
Where n=6:
Vatoms=6⋅43πr3=8πr3
Step 4: Calculate the empty space
The empty space in the unit cell is the difference between the volume of the unit cell and the volume occupied by the atoms:
Vempty=Vcell−Vatoms
Substituting the values we calculated:
Vempty=24√3r3−8πr3
Final Answer
The empty space in the HCP unit cell is:
Vempty=(24√3−8π)r3
In an HCP structure, we have:
- Two hexagonal faces (top and bottom) and three layers of atoms.
- The first layer consists of one atom at the center and six surrounding atoms, making a total of 7 atoms in the first layer.
- The second layer consists of three atoms positioned in the gaps of the first layer.
- The third layer is identical to the first layer.
The volume of the unit cell can be calculated using the formula for the volume of a hexagonal prism:
Vcell=3√32a2c
Where:
- a is the distance between the centers of two adjacent spheres in the same layer (which is 2r).
- c is the height of the unit cell, which is 4r√2 for HCP.
Vcell=3√32(2r)2(4r√2)
=3√32⋅4r2⋅4r√2
=3√3⋅16r32√2=24√3r3
In HCP, there are 6 atoms in the unit cell (1 from the top face, 1 from the bottom face, and 4 from the three layers). The volume occupied by the atoms is given by:
Vatoms=n⋅43πr3
Where n=6:
Vatoms=6⋅43πr3=8πr3
The empty space in the unit cell is the difference between the volume of the unit cell and the volume occupied by the atoms:
Vempty=Vcell−Vatoms
Substituting the values we calculated:
Vempty=24√3r3−8πr3
The empty space in the HCP unit cell is:
Vempty=(24√3−8π)r3
Topper's Solved these Questions
Similar Questions
In hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space -filling model of this structure, called hexagonal close-packed (HCP), is consituted of a sphere on a flat surface surrouneded in the same plane by six identical spheres as closely possible. Three sphere are then palces over the first layer so that they touch each other and represent second layer is covered with third layer that is identical to the bottom layer in relative position. Assume radius of enery sphere to be r.
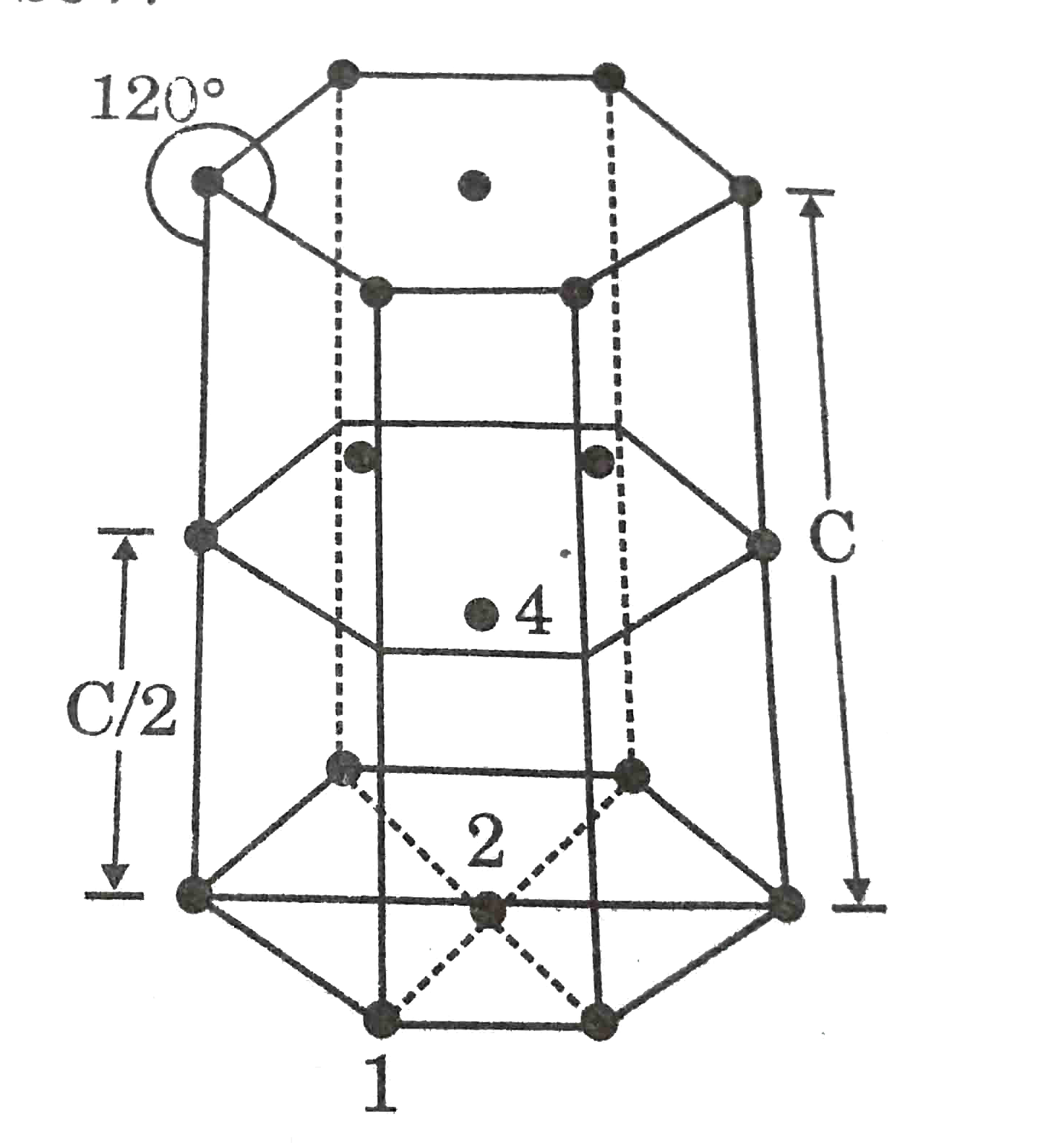
The number of atoms in the HPC unit cell is :
View SolutionIn hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space -filling model of this structure, called hexagonal close-packed (HCP), is consituted of a sphere on a flat surface surrouneded in the same plane by six identical spheres as closely possible. Three sphere are then palces over the first layer so that they touch each other and represent second layer is covered with third layer that is identical to the bottom layer in relative position. Assume radius of enery sphere to be r.

The number of atoms in the HPC unit cell is :
View Solution
Knowledge Check
In hexogonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexongonas and three atoms are sandwiched inbetween them. A space filling model of this structure called haxagonal closed packed (HCP) is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible . Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'.
The empty space in this HCP unit cell isA0.26B6C12D17In a hexaonal system system of cycstals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are refular hexagons, and three atoms are sandwiched in between them. A space-cilling model of this structure, called hexagonal close-paked is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spherres are then placed overt the first layer so that they toych each other and represent the second layer so that they toych each other and present the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is convered with a third layer identical to the bottom layer in relative position. Assume the radius of every sphere to be r.
The voume of this hcp unit cell isA24√2r3B16√2r3C12√2r3D643√3r3In hexogonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexongonas and three atoms are sandwiched inbetween them. A space filling model of this structure called haxagonal closed packed (HCP) is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible . Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'.
The empty space in this HCP unit cell isA74%B47.6%C32%D26%
In a hexaonal system system of cycstals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are refular hexagons, and three atoms are sandwiched in between them. A space-cilling model of this structure, called hexagonal close-paked is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spherres are then placed overt the first layer so that they toych each other and represent the second layer so that they toych each other and present the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is convered with a third layer identical to the bottom layer in relative position. Assume the radius of every sphere to be r.
The voume of this hcp unit cell is
View SolutionIn hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The volume of this HCP unit cell is
View SolutionIn hexagonal systems of crystals, a frequently ecounted arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are reqular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. There spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three sphere touches three spheres of the bottom layer. Finally the second layer is covered with third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'

The volume of this HCP unit cell is
View SolutionIn hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The empty space in this HCP unit cell is
View SolutionIn hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The number of atoms in this HCP unit cell is
View Solution
