In hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The number of atoms in this HCP unit cell is
4
6
12
17
The correct Answer is:B
The effective number of atoms in the HCP is
=12×16+2×12+3=6
Topper's Solved these Questions
- STATES OF MATTER (SOLID STATE CHEMISTRY)BOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER (SOLID STATE CHEMISTRY)EXERCISE - MATRIX MATCH TYPE MCQs
4 Videos
- STATES OF MATTER (SOLID STATE CHEMISTRY)BOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER (SOLID STATE CHEMISTRY)EXERCISE - INTEGER TYPE QUESTIONS
1 Videos
- STATES OF MATTER (SOLID STATE CHEMISTRY)BOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER (SOLID STATE CHEMISTRY)EXERCISE - SELECTED STRAIGHT OBJECTIVE TYPE MCQs
23 Videos
- STATE OF MATTER (GASEOUS AND LIQUID STATE)BOOK - DINESH PUBLICATIONCHAPTER - STATE OF MATTER (GASEOUS AND LIQUID STATE)EXERCISE - Ulitmate Preparatory package
20 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - DINESH PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - Statement Type Question
5 Videos
Similar Questions
In hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure, called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be ‘r’.
The empty space in this HCP unit cell is :
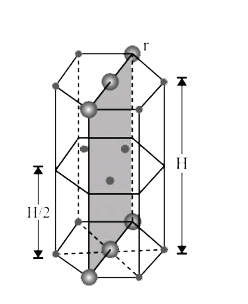
View SolutionIn hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure, called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be ‘r’.
The volume of this HCP unit cell is
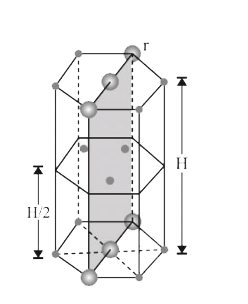
View Solution
Knowledge Check
In hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The volume of this HCP unit cell isA24√2r3B16√2r3C12√2r3D643√3r3In hexagonal system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagon and three atoms are sandwiched in between them. A space filling model of this structure, called hexagonal close packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical sphere as closely as possible. Three spheres are then placed over the first layer so that they touch other and represent the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be same
The empty space in this HCP unit cell isA0.74B0.476C0.32D0.26In hexagonal systems of crystals, a frequently ecounted arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are reqular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. There spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three sphere touches three spheres of the bottom layer. Finally the second layer is covered with third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'

The number of atoms in the HCP unit cell isA4B6C12D17
In a hexagonal system system of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons, and three atoms are sandwiched in between them. A space-cilling model of this structure, called hexagonal close-packed is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spheres are then placed overt the first layer so that they touch each other and represent the second layer so that they touch each other and present the second layer. Each one of the three spheres touches three spheres of the bottom layer. Finally, the second layer is converted with a third layer identical to the bottom layer in relative position. Assume the radius of every sphere to be r.
The number of atom in this hcp unit cell is
(a)4
(b)6
(c)12
(d)17
View SolutionIn hexagonal systems of crystals, a frequently ecounted arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are reqular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. There spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three sphere touches three spheres of the bottom layer. Finally the second layer is covered with third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'

The volume of this HCP unit cell is
View SolutionIn hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism.Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them.A space-fitting model of this structure, called hexagonal closed-packed (HCP), is constitution of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible Three spheres are then placed over the first layer so that they touch each other and represent the second layer.Each one of these three spheres touches three spheres of the bottom layer.Finally, the second layer is covered with third layer that is identical to the bottom layer in relative position.Assume radius of even sphere to be 'r'.
The volume of this HCP unit cell is :
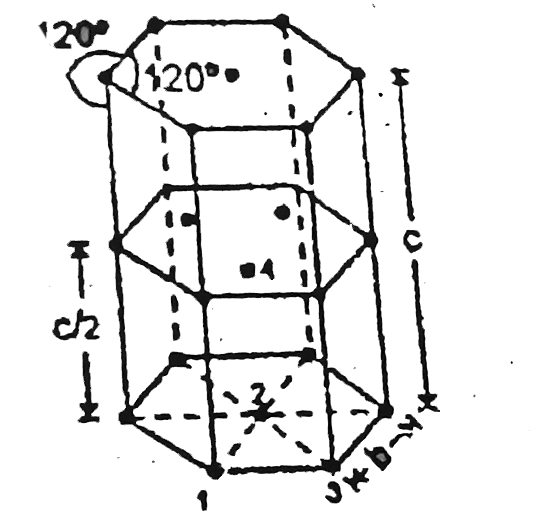
View SolutionIn hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure, called hexagonal close-packed (hcp), is constitued of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three spheres touched three spheres of the bottom layer. Finally, the second layer is convered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be 'r'.
The volume of this hcp unit cell is
View SolutionIn hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism.Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them.A space-fitting model of this structure, called hexagonal closed-packed (HCP), is constitution of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible Three spheres are then placed over the first layer so that they touch each other and represent the second layer.Each one of these three spheres touches three spheres of the bottom layer.Finally, the second layer is covered with third layer that is identical to the bottom layer in relative position.Assume radius of even sphere to be 'r'.
In hcp lattice, if a plane is drawn parallel to layers A and B at a distance c8 above the layer B, it passes through centres of x tetrahedral and y octahedral voids.A plane parallel to layer A at a distance c8 just below it passes through centres of p tetrahedral and q octahedral voids.Find (x+y+p+q) c is the cell parameter of hcp lattice, the vertical edge length.

View Solution
