Intitial rate method: Use the following initial rate data determine the form of the rate law expressoin for the reaction 3X+2Y→2U+V
![Intitial rate method: Use the following initial rate data determine the form of the rate law expressoin for the reaction 3X+2Yrarr2U+V Strategy: The rate law is of the form Rate = k[X]^(a)[Y]^(b) No two experiments have the same in initial [Y], thus, use the alternative method discussed earlier to evalaute a and b.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/RS_P2_CHM_C04_S01_010_Q01.png)
Strategy: The rate law is of the form
Rate=k[X]a[Y]b
No two experiments have the same in initial [Y], thus, use the alternative method discussed earlier to evalaute a and b.
The initial concentration of X is the same in expermental 1 and 3, thus we divide the third rate law expression by the corresponding terms in the first one:
Rate3Rate1=k[X]a3[Y]b3k[X]a1[Y]b1
The value k cancels, and so do the concentrations of X because they are equal. Consequently, the expression simplifies to
Rate3Rate1=([Y]3[Y]1)b
Subsituting known values of rates and [Y],
1.20×10−2Ms−16.00×10−3Ms−1=(2.00×10−2M1.00×10−2M)b
2.0=(2.0)b
or b=1
Thus, the reaction is first order in Y. No two of the expermental runs have the same concentrations of Y, so we must proceed somewhat differntly. Let us compare expermints 1 and 2. The observed change in rate must be due to the ombination of the change in [X] and [Y]. We can divide the second rate law expression by the correponding terms in the first one. Canceling the equal k values and collecting terms, we get
Rate2Rate1=k[X]a2[Y]b2k[X]a1[Y]b1=([X]2[X]1)a([Y]2[Y]1)b
Inserting the known the known values for rates and concentratoins and the known [Y] exponent of 1, we have.
1.44×10−1Ms−16.00×10−3Ms−1=(2.00×10(−2)M1.00×10−2M)a(3.00×10−2M1.00×10−2M)1
24.0=(2.00)a(3.00)
800=(2.00)a
(2.00)3=(2.00)
Thus, a=3
and the reaction is third order in X. The complete rate law expression has the from
Rate=k[X]3[Y]
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - R SHARMACHAPTER - CHEMICAL KINETICSEXERCISE - Follow-up 1
8 Videos
- CHEMICAL KINETICSBOOK - R SHARMACHAPTER - CHEMICAL KINETICSEXERCISE - Follow-up 2
10 Videos
- BIOMOLECULESBOOK - R SHARMACHAPTER - BIOMOLECULESEXERCISE - Archives
47 Videos
- CHEMISTRY IN EVERYDAY LIFEBOOK - R SHARMACHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - Archives
11 Videos
Similar Questions
Method of intial rates: Given the following data, determine the rate law expression and the value of the rate constant for the reaction. 2X+Y+Z→U+V
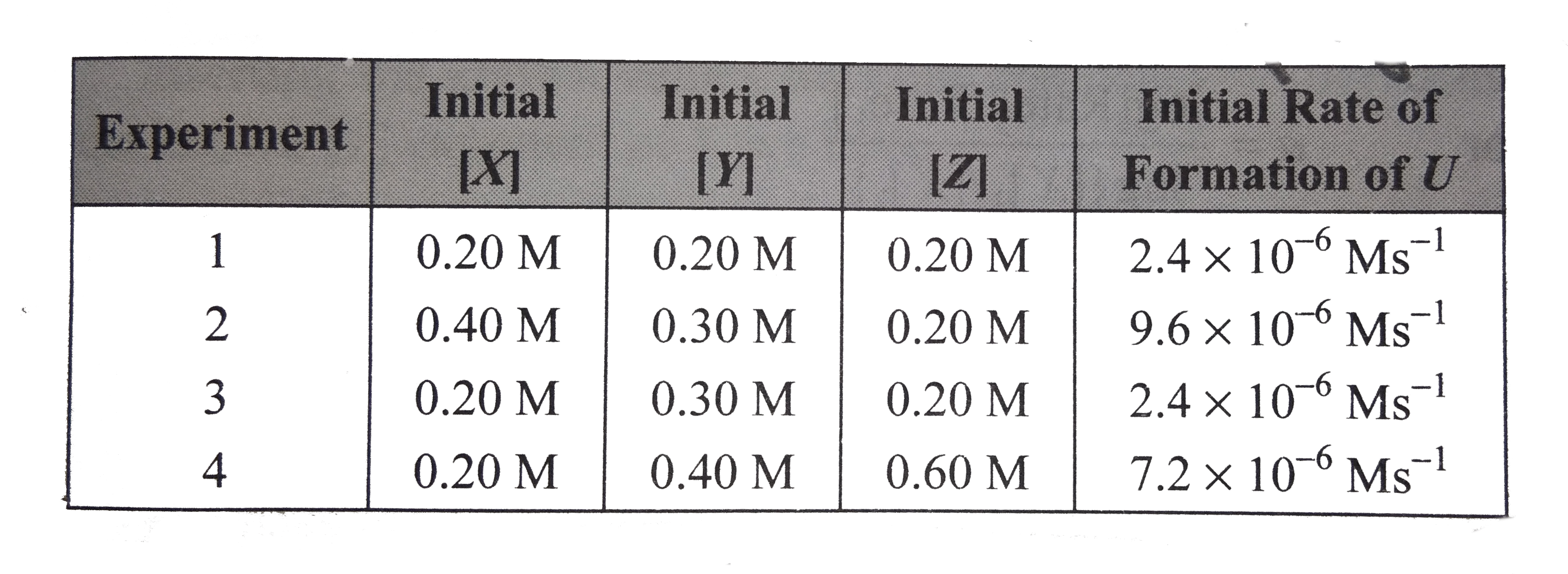
Strategy: The rate law is of the from
Rate=k[X]a[Y]b[Z]c
We must evaluate a,b,c, and k by using the reasoning outlined earlier. Thus, to determine order with respect to X, we must select those experiments in which concentration of X changes but the concentrations of Y and Z are kept onstant and so on. Note that the coefficient of U in the balenced equation is 1, so the rate of reaction is equal to the rate of formation of U.
View SolutionThe following initial rate data were obtained at 300K for the reactions:
2A+B→C+D

Deducethe rate law.
View Solution
Knowledge Check
Form the data given below for the reaction:A→B→ Products
the rate law expression is:ARate=k[A][B]BRate=k[A][B]2CRate=k[A]2[B]2DRate=k[A]2[B]The rate law for the single step reaction 2A+B→X given by
ARate =K[A][B]BRate =K[A]2[B]CRate =K[2A][B]DRate =K[A]2[B]0The rate law for the single step reaction 2A+B→X given by
ARate =K[A][B]BRate =K[A]2[B]CRate =K[2A][B]DRate =K[A]2[B]0
The rate law for a reaction is Rate = K[A][B]3/2
Can the reaction be an elementary process ? Explain.
View SolutionThe following initial rate data were obtianed for the reaction
2NOg+Br(g)→2NOBr(g)
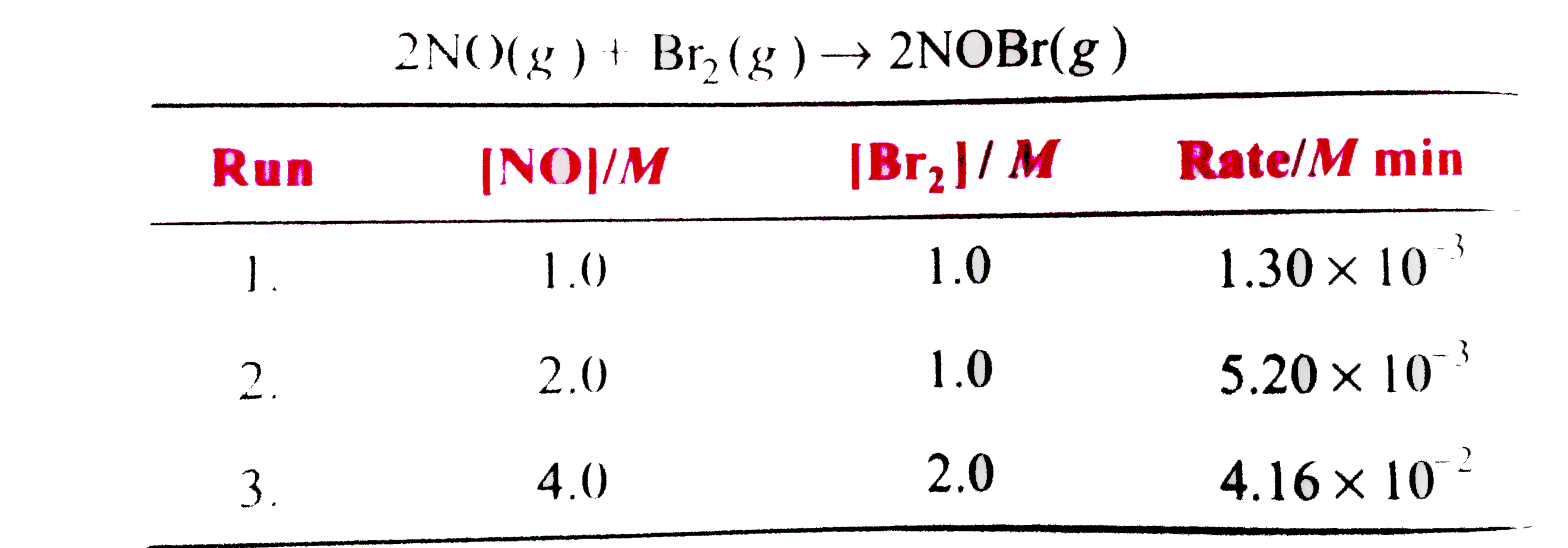
Determine the reaction rat law and the value of te rate constant.
View SolutionFor a hypothetical reaction aA+bB→ Product, the rate law is: rate =K[A]x[B]y, then
View SolutionThe rate law for the reaction
2X+Y→Z is Rate =k[X][Y]
The correct statement with regard to this relation is
View SolutionFor the reaction X+3Y→Z, which form of differential rate law is incorrect?
View Solution
