The energy of a I,II and III energy levels of a certain atom are E,4E3 and 2E respectively. A photon of wavelength λ is emitted during a transition from III to I. what will be the wavelength of emission for II to I?
λ2
λ
2λ
3λ
The correct Answer is:D
To solve the problem, we need to find the wavelength of the photon emitted during the transition from the second energy level (E2) to the first energy level (E1) of a certain atom, given the energy levels and the wavelength of a photon emitted during the transition from the third energy level (E3) to the first energy level (E1).
1. Identify the Energy Levels:
- E1 = E (Energy of the first level)
- E2 = (4/3)E (Energy of the second level)
- E3 = 2E (Energy of the third level)
2. Calculate the Energy Difference for the Transition from E3 to E1:
- The energy difference (ΔE) when a transition occurs from E3 to E1 is:
ΔE3→1=E3−E1=2E−E=E
3. Relate Energy to Wavelength:
- The energy of the emitted photon is related to its wavelength (λ) by the equation:
E=hcλ
- From the transition E3 to E1, we have:
E=hcλ
- This gives us our first equation:
E=hcλ(Equation 1)
4. Calculate the Energy Difference for the Transition from E2 to E1:
- Now, we calculate the energy difference for the transition from E2 to E1:
ΔE2→1=E2−E1=4E3−E=4E3−3E3=E3
5. Relate Energy Difference to Wavelength for the Transition from E2 to E1:
- Using the same energy-wavelength relationship:
ΔE2→1=hcλ′
- Substituting the energy difference we found:
E3=hcλ′
6. Substituting Equation 1 into the New Equation:
- From Equation 1, we know that E=hcλ. Substituting this into the equation gives:
hcλ3=hcλ′
7. Canceling hc and Rearranging:
- Cancel hc from both sides:
13λ=1λ′
- Rearranging gives:
λ′=3λ
Final Answer:
The wavelength of emission for the transition from the second energy level to the first energy level is:
λ′=3λ
- E1 = E (Energy of the first level)
- E2 = (4/3)E (Energy of the second level)
- E3 = 2E (Energy of the third level)
- The energy difference (ΔE) when a transition occurs from E3 to E1 is:
ΔE3→1=E3−E1=2E−E=E
- The energy of the emitted photon is related to its wavelength (λ) by the equation:
E=hcλ
- From the transition E3 to E1, we have:
E=hcλ
- This gives us our first equation:
E=hcλ(Equation 1)
- Now, we calculate the energy difference for the transition from E2 to E1:
ΔE2→1=E2−E1=4E3−E=4E3−3E3=E3
- Using the same energy-wavelength relationship:
ΔE2→1=hcλ′
- Substituting the energy difference we found:
E3=hcλ′
- From Equation 1, we know that E=hcλ. Substituting this into the equation gives:
hcλ3=hcλ′
- Cancel hc from both sides:
13λ=1λ′
- Rearranging gives:
λ′=3λ
The wavelength of emission for the transition from the second energy level to the first energy level is:
λ′=3λ
Topper's Solved these Questions
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - Level 3 (Passage 1)
3 Videos
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - Level 3 (Passage 2)
4 Videos
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - Subjective problems
1 Videos
- CHEMICAL EQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Match the column
1 Videos
Similar Questions
The energies of three conservative energy levels L3,L2 and L1 of hydrogen atom are E0. 4E09 and E04 respectively. A photon of wavelength λ is emitted for a transition L3 to L1 .What will be the wavelength of emission for transition L2 to L1 ?
View Solutionwhat are the frequency and wavelength of a photon emitted during a transition from n = 5 state to the n =2 state in the hydrogen atom?
View Solutionwhat are the frequency and wavelength of a photon emitted during a transition from n = 5 state to the n =2 state in the hydrogen atom?
View SolutionThe following diagram indicates the energy levels of a certain atom when the system moves from 4E level to E. A photon of wavelength λ1 is emitted. The wavelength of photon produced during its transition from 73E level to E is λ2. the ratio λ1λ2 will be
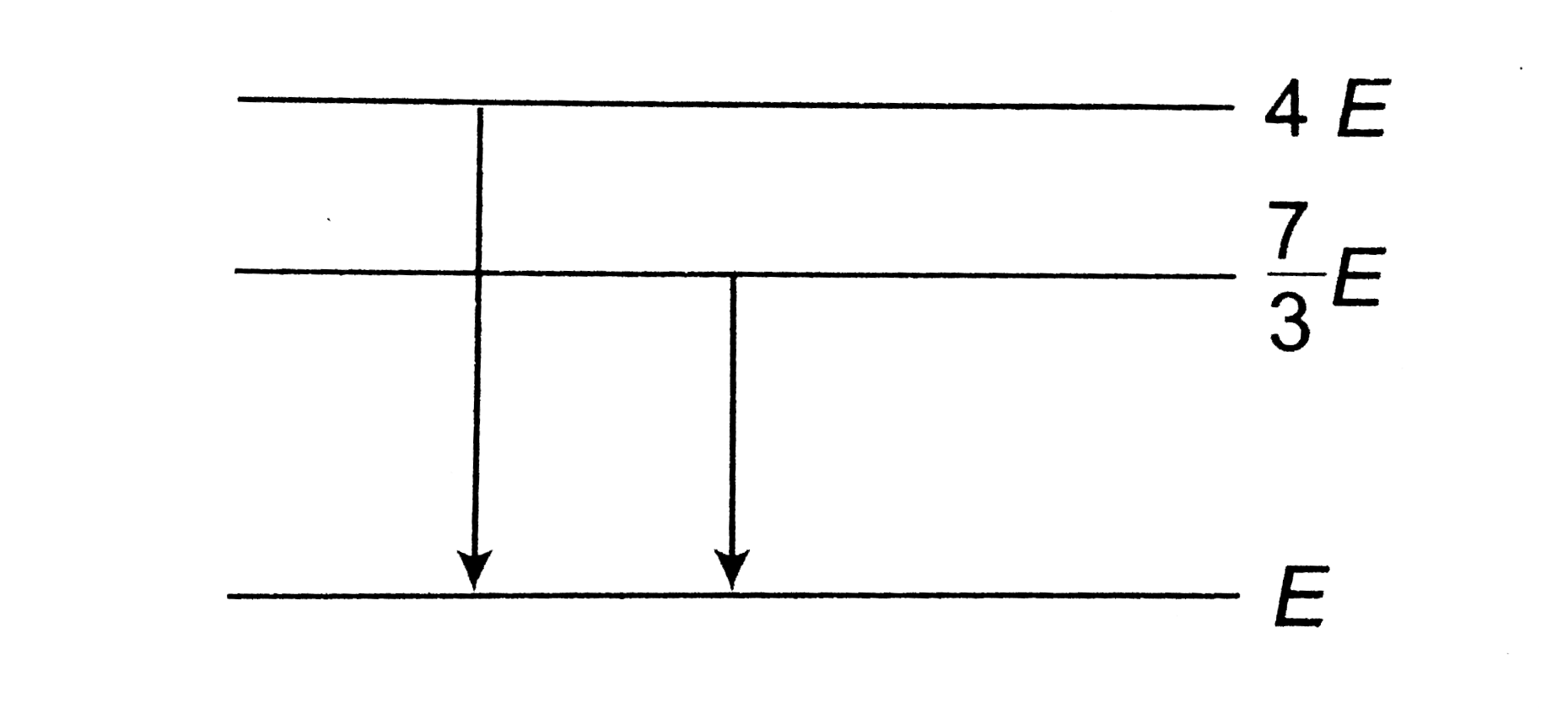
View SolutionThe following diagram indicates the energy levels of a certain atom when the system moves from 4E level to E. A photon of wavelength λ1 is emitted. The wavelength of photon produced during its transition from 73E level to E is λ2. the ratio λ1λ2 will be

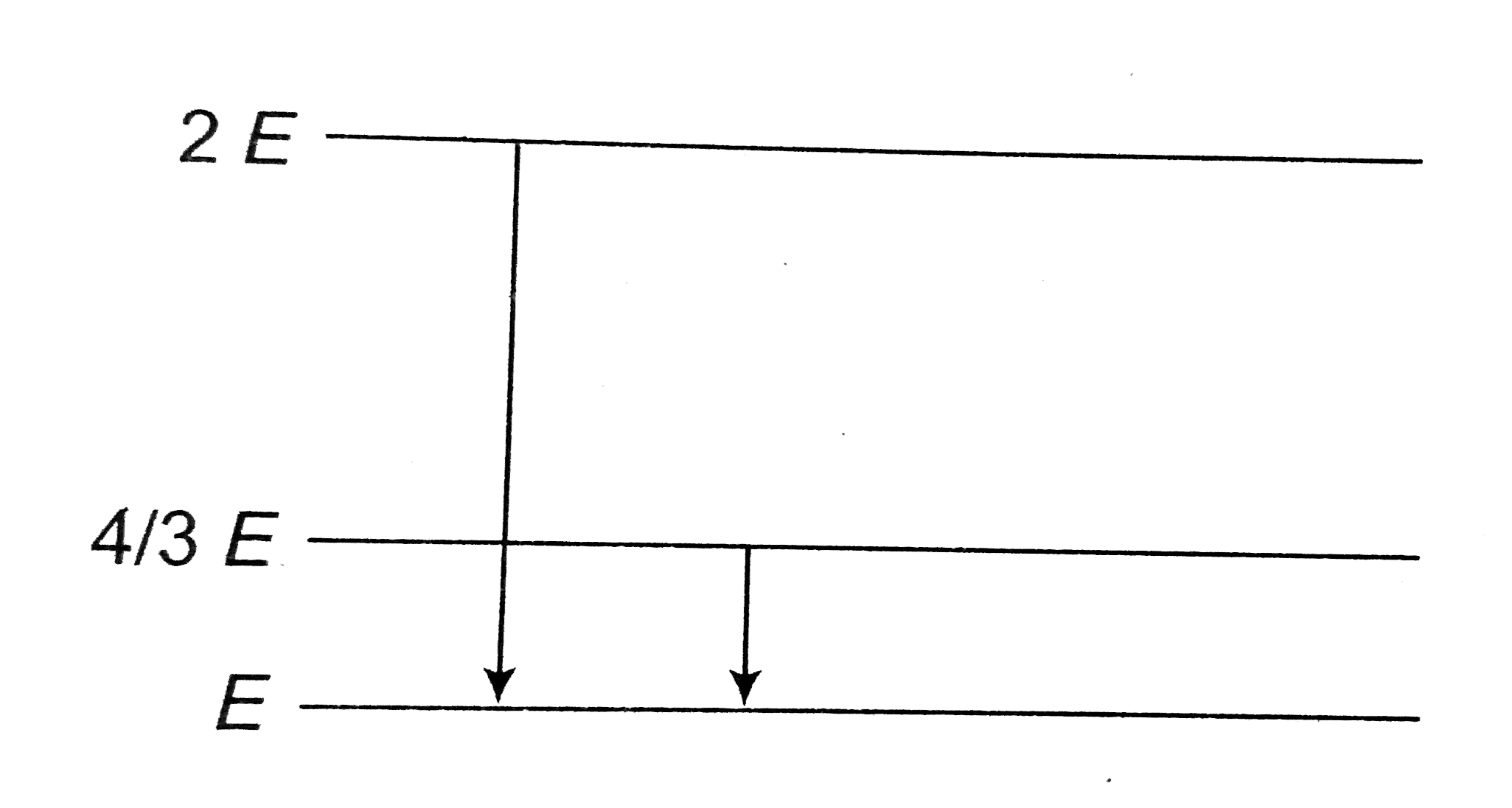
View SolutionThe follwing diagram indicates the energy levels of a certain atom when the system moves from 2E level to E, a photon of wavelength λ is emitted. The wavelength of photon produced during its transition from 4E3 level to E is
View SolutionThe energy levels of a cartain atom are shown in figure. If a photon of
frequency f is emitted when there is an electron transition from 5E to E
what frequencies of photons could be produced by other energy level transitions?

View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectroic effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejercted electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Aborbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−01λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
The folloeing figure indicates the energy livels of a certain atom .When the system moves from 2E level to E lvel a photon of wavelength λ is emitted .The wavelength of the photon produced during the transition from level 4E/3 to level E is

View SolutionWhat will be the kinetic energy and total energy of an electron in H atom if the atom emit a photon of wavelength 4860Å ?
View SolutionExcited atoms emits radiations consisting of only certain discrete frequencies or wavelengths. In spectroscopy it is often more convenient to use frequencies or wave numbers than are proportional to energy and spectroscopy involves transitions between energy levels. The line spectrum shown by and mono electronic excited atom (a finger pring of an atom) can be given as
1λ=¯v=RAZ2[1n21−1n22]
When Z is atomic number of mono electronic atom and n1,n2 are integers and if n2>n1, then emission spectrum is noticed and if n2<n1, then absorptioni spectrum is noticed. Every line in spectrum can be represented as differences of two terms RAZ2n21 and RAZ2n22
The given diagrma indicates the energ levels of certain atom. Where an electron moves from 2E.
level to E level, a photon of wavelength of photon emitted during its transition from 4E3 level to E level.
View Solution
