The molar conductivity of KCl solutions at different concentration at 298K are given below :
C/mol L−1∧m/Scm2mol−10.000198148.610.000309148.290.000521147.810.000987143.09
Show that a plot between ∧mandC1/2 is a straight line. Determine the values of ∧0mand∧ for KCl.
To solve the problem, we need to show that a plot of molar conductivity (Λ_m) against the square root of concentration (C^(1/2)) is a straight line. We will also determine the values of Λ_m^0 (the limiting molar conductivity) and the slope (a) for KCl.
1. List the Given Data:
The molar conductivity (Λ_m) values at different concentrations (C) are:
- C = 0.000198 mol/L, Λ_m = 148.61 S cm²/mol
- C = 0.000309 mol/L, Λ_m = 148.29 S cm²/mol
- C = 0.000521 mol/L, Λ_m = 147.81 S cm²/mol
- C = 0.000987 mol/L, Λ_m = 143.09 S cm²/mol
2. Calculate C^(1/2):
We will calculate the square root of each concentration:
- For C = 0.000198: C^(1/2) = √0.000198 = 0.01407
- For C = 0.000309: C^(1/2) = √0.000309 = 0.01758
- For C = 0.000521: C^(1/2) = √0.000521 = 0.02283
- For C = 0.000987: C^(1/2) = √0.000987 = 0.03145
3. Create a Table of Values:
Now we can create a table with the calculated values:
| C (mol/L) | C^(1/2) (mol^(1/2)/L^(1/2)) | Λ_m (S cm²/mol) |
|-----------|------------------------------|------------------|
| 0.000198 | 0.01407 | 148.61 |
| 0.000309 | 0.01758 | 148.29 |
| 0.000521 | 0.02283 | 147.81 |
| 0.000987 | 0.03145 | 143.09 |
4. Plotting the Graph:
- On the x-axis, plot C^(1/2) values.
- On the y-axis, plot the corresponding Λ_m values.
- From the data, we can observe that as C^(1/2) increases, Λ_m decreases, suggesting a linear relationship.
5. Determine the Equation of the Line:
The relationship can be expressed as:
Λm=Λ0m−a⋅C1/2
where:
- Λ0m is the intercept (limiting molar conductivity).
- a is the slope of the line.
6. Calculate the Intercept (Λ_m^0):
From the graph, when C^(1/2) = 0, the value of Λ_m can be estimated. By observing the trend, we find:
Λ0m≈150S cm2/mol
7. Calculate the Slope (a):
To find the slope (a), we can use two points from the graph:
- Let’s take the points (0.01407, 148.61) and (0.03145, 143.09):
a=Λm2−Λm1C1/22−C1/21=143.09−148.610.03145−0.01407
a=−5.520.01738≈−317.3S cm2/mol1/2
Final Results:
- Λ0m≈150S cm2/mol
- a≈317.3S cm2/mol1/2
The molar conductivity (Λ_m) values at different concentrations (C) are:
- C = 0.000198 mol/L, Λ_m = 148.61 S cm²/mol
- C = 0.000309 mol/L, Λ_m = 148.29 S cm²/mol
- C = 0.000521 mol/L, Λ_m = 147.81 S cm²/mol
- C = 0.000987 mol/L, Λ_m = 143.09 S cm²/mol
We will calculate the square root of each concentration:
- For C = 0.000198: C^(1/2) = √0.000198 = 0.01407
- For C = 0.000309: C^(1/2) = √0.000309 = 0.01758
- For C = 0.000521: C^(1/2) = √0.000521 = 0.02283
- For C = 0.000987: C^(1/2) = √0.000987 = 0.03145
Now we can create a table with the calculated values:
|-----------|------------------------------|------------------|
| 0.000198 | 0.01407 | 148.61 |
| 0.000309 | 0.01758 | 148.29 |
| 0.000521 | 0.02283 | 147.81 |
| 0.000987 | 0.03145 | 143.09 |
- On the x-axis, plot C^(1/2) values.
- On the y-axis, plot the corresponding Λ_m values.
- From the data, we can observe that as C^(1/2) increases, Λ_m decreases, suggesting a linear relationship.
The relationship can be expressed as:
Λm=Λ0m−a⋅C1/2
where:
- Λ0m is the intercept (limiting molar conductivity).
- a is the slope of the line.
From the graph, when C^(1/2) = 0, the value of Λ_m can be estimated. By observing the trend, we find:
Λ0m≈150S cm2/mol
To find the slope (a), we can use two points from the graph:
- Let’s take the points (0.01407, 148.61) and (0.03145, 143.09):
a=Λm2−Λm1C1/22−C1/21=143.09−148.610.03145−0.01407
a=−5.520.01738≈−317.3S cm2/mol1/2
- Λ0m≈150S cm2/mol
- a≈317.3S cm2/mol1/2
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE (PART-I Objective Questions)
28 Videos
- ELECTROCHEMISTRYBOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - EXERCISE (PART-I Objective Questions) (Choose the correct alternative)
25 Videos
- ELECTROCHEMISTRYBOOK - ICSECHAPTER - ELECTROCHEMISTRYEXERCISE - ISC EXAMINATION QUESTIONS (PART-II Numerical Problems)
19 Videos
- DISTINCTION BETWEEN PAIRS OF COMPOUNDS BOOK - ICSECHAPTER - DISTINCTION BETWEEN PAIRS OF COMPOUNDS EXERCISE - QUESTIONS
155 Videos
- GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS BOOK - ICSECHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTS EXERCISE - EXERCISE (PART-I ( OBJECTIVE QUESTIONS )) (Fill in the blanks)
60 Videos
Similar Questions
The molar conductivity of KCl solution at different concentrations at 298K is given below :
corM(molL−1)∧m(Scm2mol−1)0.000198148.610.000309148.290.000521147.810.000989147.09
Show that a plot between ∧m and √c is a straight line. Determine the value of ∧∘m and A for KCl .
View SolutionThe pressure-volume of varies thermodynamic process is shown in graphs:
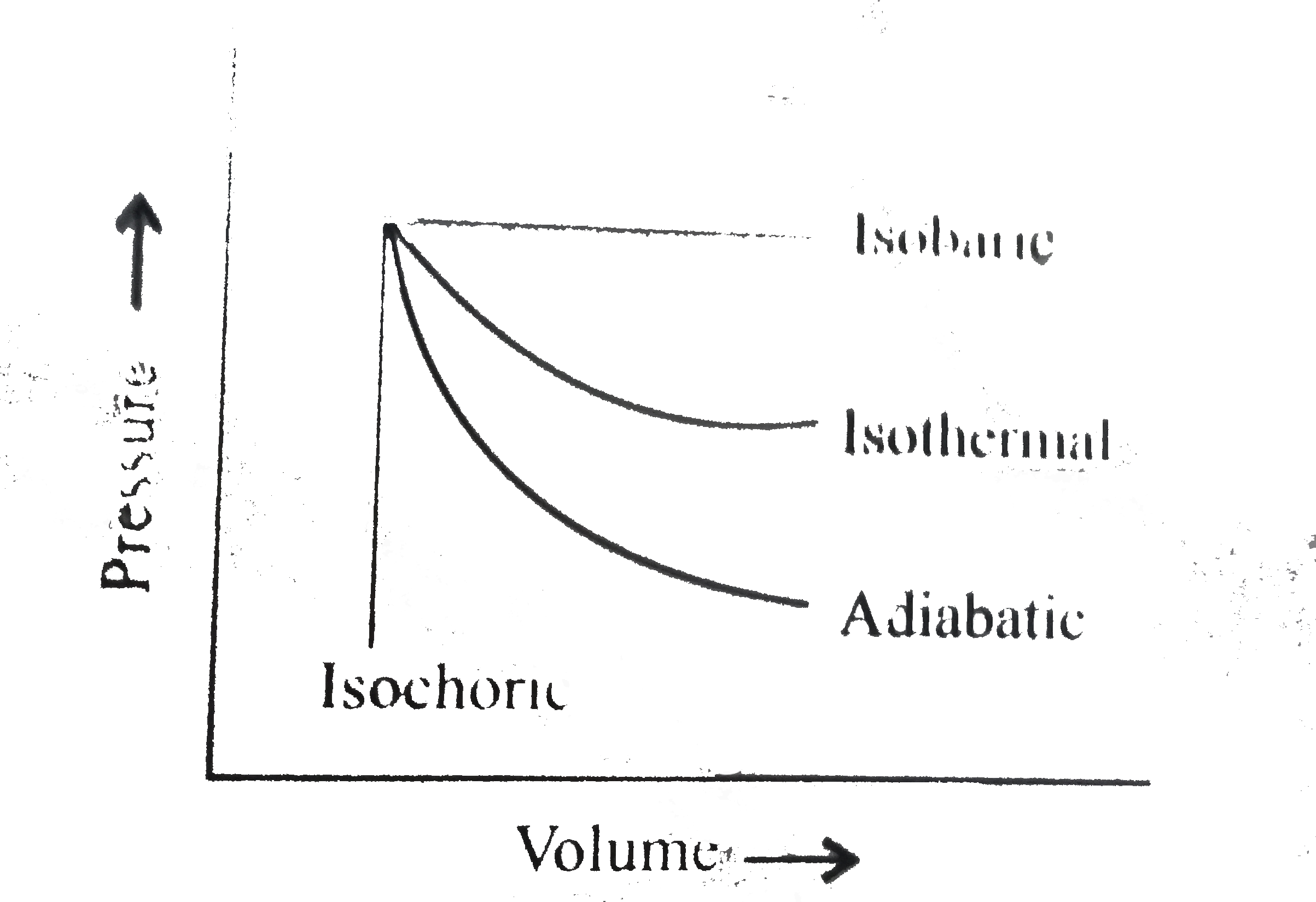
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=−2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
Calculate work done when 1 mole of an ideal gas is expanded reversibly from 30L to 60L at a constant temperature of 300K
View Solution
Knowledge Check
Molar conductivity of 0.15 M solution of KCI at 298 K, if its conductivity is 0.0152 S cm−1 will be
A124Ω−1cm2mol−1B204Ω−1cm2mol−1C101Ω−1cm2mol−1D300Ω−1cm2mol−1
The boiling point of 0.1MKCl solution is ………..than 100∘C.
View SolutionThe conductivity of 0.10 M solution of KCl at 298 K is 0.025 S cm Calculate its molar conductivity.
View SolutionThe conductivity of 0.20 M solution of KCl at 298 K is 0.0248 S cm−1 . Calculate its molar conductivity.
View SolutionThe conductivity of 0.20 M solution of KCl at 298 K is 0.0248 S cm−1 . Calculate its molar conductivity.
View SolutionThe conductivity of a 0.01 M solution of acetic acid at 298 k is 1.65×10−4Scm−1. Calculate molar conductivity (∧m) of the solution.
View SolutionThe conductivity of a 0.01 M solution of acetic acid at 298 k is 1.65×10−4Scm−1. Calculate molar conductivity (∧m)of the solution.
View SolutionThe conductivity of 0.20 M solution of KCl at 298 K is 0.025 S cm−1. Calculate its molar conductivity.
View Solution
