Which one among the following is the correct option for right relationship between Cp and Cv for one mole of ideal gas?
Cp=RCv
Cv=RCp
Cp+Cv=R
Cp−Cv=R
To solve the problem regarding the relationship between Cp (molar heat capacity at constant pressure) and Cv (molar heat capacity at constant volume) for one mole of an ideal gas, we can follow these steps:
1. Understand the Definitions:
- Cv is the molar heat capacity at constant volume, which is the amount of heat required to raise the temperature of one mole of a gas by one degree Celsius while keeping the volume constant.
- Cp is the molar heat capacity at constant pressure, which is the amount of heat required to raise the temperature of one mole of a gas by one degree Celsius while keeping the pressure constant.
2. Apply the First Law of Thermodynamics:
- The first law of thermodynamics states that:
ΔU=Q−W
- Here, ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
3. Express Heat and Work:
- For an ideal gas at constant volume:
Q=nCvΔT
- For an ideal gas at constant pressure:
Q=nCpΔT
4. Relate Internal Energy Change:
- The change in internal energy for one mole of an ideal gas can be expressed as:
ΔU=nCvΔT
5. Identify Work Done:
- At constant pressure, the work done by the gas is given by:
W=PΔV
- For an ideal gas, using the ideal gas law PV=nRT, we can express W in terms of n and ΔT:
W=nRΔT
6. Substitute into the First Law:
- Substituting Q and W into the first law gives:
nCvΔT=nCpΔT−nRΔT
7. Simplify the Equation:
- Dividing through by nΔT (assuming ΔT≠0):
Cv=Cp−R
8. Rearranging the Equation:
- Rearranging gives us the desired relationship:
Cp−Cv=R
Conclusion:
The correct relationship between Cp and Cv for one mole of an ideal gas is:
Cp−Cv=R
Final Answer:
The correct option is Cp−Cv=R (Option 4).
- Cv is the molar heat capacity at constant volume, which is the amount of heat required to raise the temperature of one mole of a gas by one degree Celsius while keeping the volume constant.
- Cp is the molar heat capacity at constant pressure, which is the amount of heat required to raise the temperature of one mole of a gas by one degree Celsius while keeping the pressure constant.
- The first law of thermodynamics states that:
ΔU=Q−W
- Here, ΔU is the change in internal energy, Q is the heat added to the system, and W is the work done by the system.
- For an ideal gas at constant volume:
Q=nCvΔT
- For an ideal gas at constant pressure:
Q=nCpΔT
- The change in internal energy for one mole of an ideal gas can be expressed as:
ΔU=nCvΔT
- At constant pressure, the work done by the gas is given by:
W=PΔV
- For an ideal gas, using the ideal gas law PV=nRT, we can express W in terms of n and ΔT:
W=nRΔT
- Substituting Q and W into the first law gives:
nCvΔT=nCpΔT−nRΔT
- Dividing through by nΔT (assuming ΔT≠0):
Cv=Cp−R
- Rearranging gives us the desired relationship:
Cp−Cv=R
The correct relationship between Cp and Cv for one mole of an ideal gas is:
Cp−Cv=R
The correct option is Cp−Cv=R (Option 4).
Topper's Solved these Questions
Similar Questions
Knowledge Check
Which one of the following options correct explains their respective relationships?
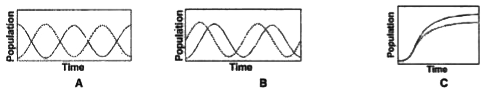 AA- Predation, B - Parasitism, C- Interspecific competition.BA-Parasitism, B - Predation, C- Interspecific competition.CA- Predation, B - Parasitism, C - Symbiosis.DA- Predation, B - Parasitism, C- Commensalism
AA- Predation, B - Parasitism, C- Interspecific competition.BA-Parasitism, B - Predation, C- Interspecific competition.CA- Predation, B - Parasitism, C - Symbiosis.DA- Predation, B - Parasitism, C- CommensalismThe K.E. of one mole of an ideal gas is
A0.5 RB0.1 RC1.5 RD2.5 RCalculate the difference between CpandCV for 10 mole of an ideal gas.
A83.14 JB8.314 JC831.4 JD0.8414 J
