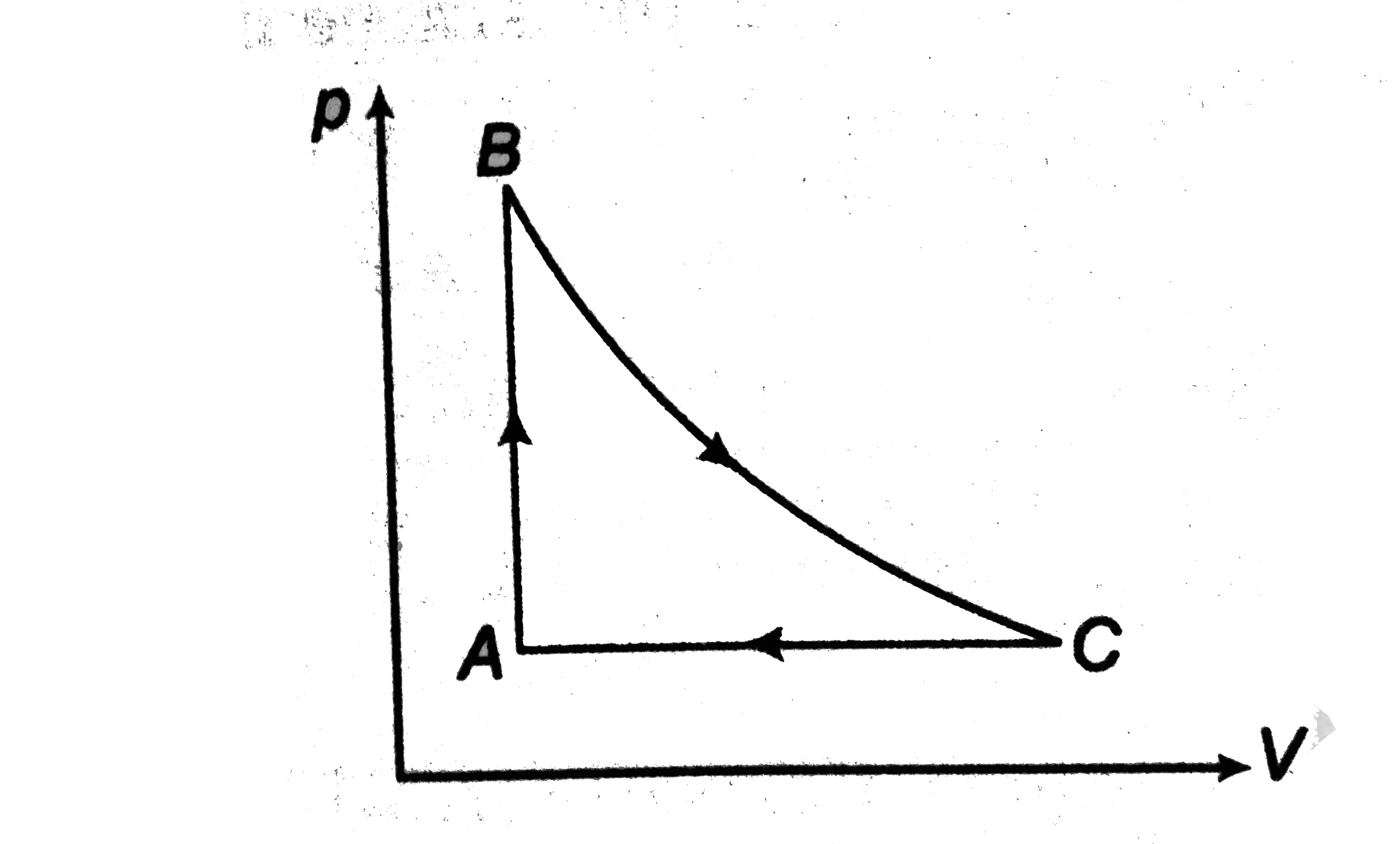
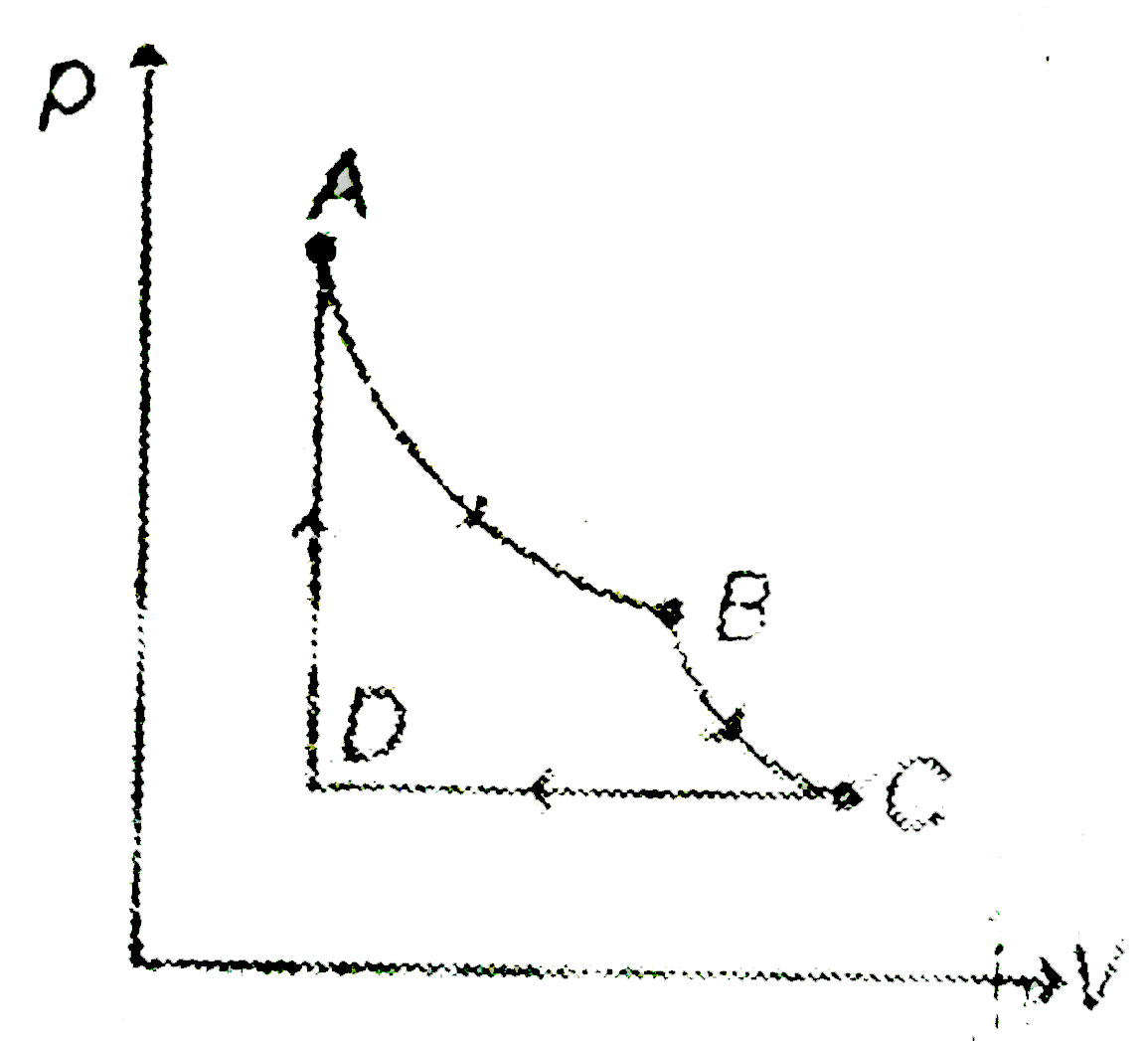
1.0 k-mol of a sample of helium gas is put through the cycle of operations shown in figure. BC is an isothermal process and pA=1.00atm, VA=22.4m3, pB=2.00atm. What are TA, TB and VC?
The correct Answer is:A, B, C, D
TA=pAVAnR=(1.013×105)(22.4)(103)(8.31)
=273K
Along path AB
V= constant
∴ T∝p
p is doubled. So, T is also doubled.
∴ TB=2TA=546K.
Further, TB=TC
∴ pBVB=pCVC
∴ VC=(pB)(VA)(pA)=(2)(22.4)1
=44.8m3
Topper's Solved these Questions
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Passage II
3 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- MAGNETIC EFFECT OF CURRENT AND MAGNETISMBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Integer type Questions
10 Videos
Similar Questions
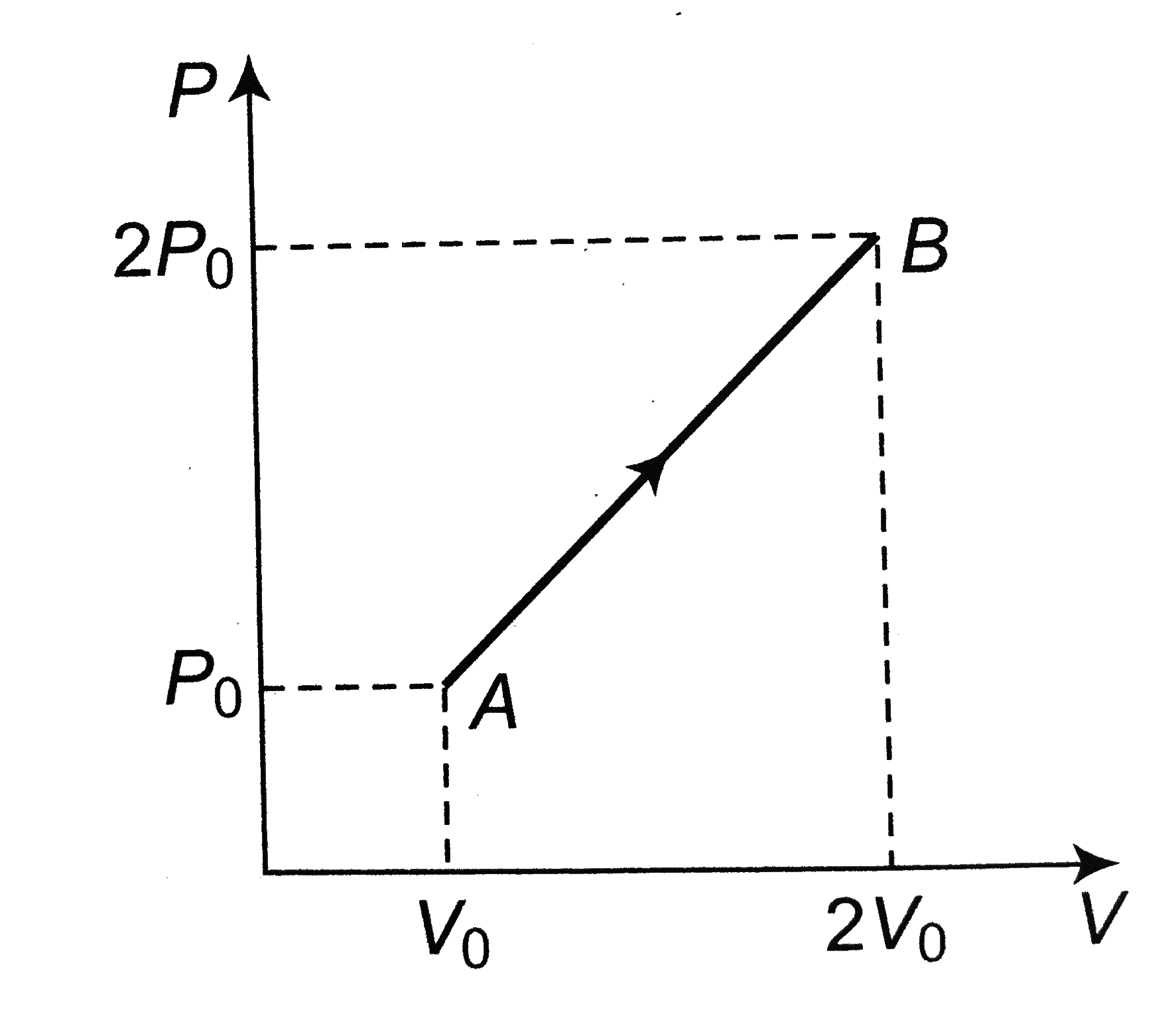
The P−V diagram of 2 gm of helium gas for a certain process A→B is shown in the figure. What is the heat given to the gas during the process A→B?
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle ABCDA as shown in the figure.
[Assume(23)0.4=0.85 and R=253JK−1mol−1]
The temperature at B is
TA=1000K and 2pA=3pB=6pC

View SolutionOne mole of a monoatomic ideal gas is taken through the cycle shown in figure The pressure and temperature at A, B etc are denoted by PA,PB−,TA,TB−, etc respectively
A → B adiabatic expansion
B → C cooling at constant volume
C→D adiabatic compression
D→A heating at constant volume
Given that : TA=1000 K, PB=23PA and PC=13PA
_E01_271_Q01.png)
the heat lost by the gas in process B→C
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle shown in figure The pressure and temperature at A, B etc are denoted by PA,PB,TA,TB−, etc respectively
A → B adiabatic expansion
B → C cooling at constant volume
C→D adiabatic compression
D→A heating at constant volume
Given that : TA=1000 K, PB=23PA and PC=13PA
_E01_271_Q01.png)
the work done by the gas in process A → B
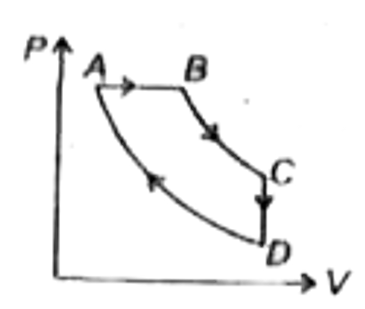
View SolutionFor P-V diagram of a thermodynamic cycle as shown in figure,process BC and DA are isothermal. Which of the corresponding graphs is correct?
View SolutionA reversible heat engine carries 1mol of an ideal monatomic gas around the cycle ABCA, as shown in the diagram. The process BC is adiabatic. Call the processes AB,BC and CA as 1,2 and 3 and the heat (ΔQ)r, change in internal energy (ΔU), and work done (ΔW)r,r=1,2,3 respectively. The temperature at A,B,C are T1=300K,T2=600K and T3=455K. Indicate the pressure and volume at A,B andC by Pr and Vr,r=1,2,3, respectively. Assume that intially pressure P1=1.00atm.
Which of the following represents the correct values of the quantities indicated ?
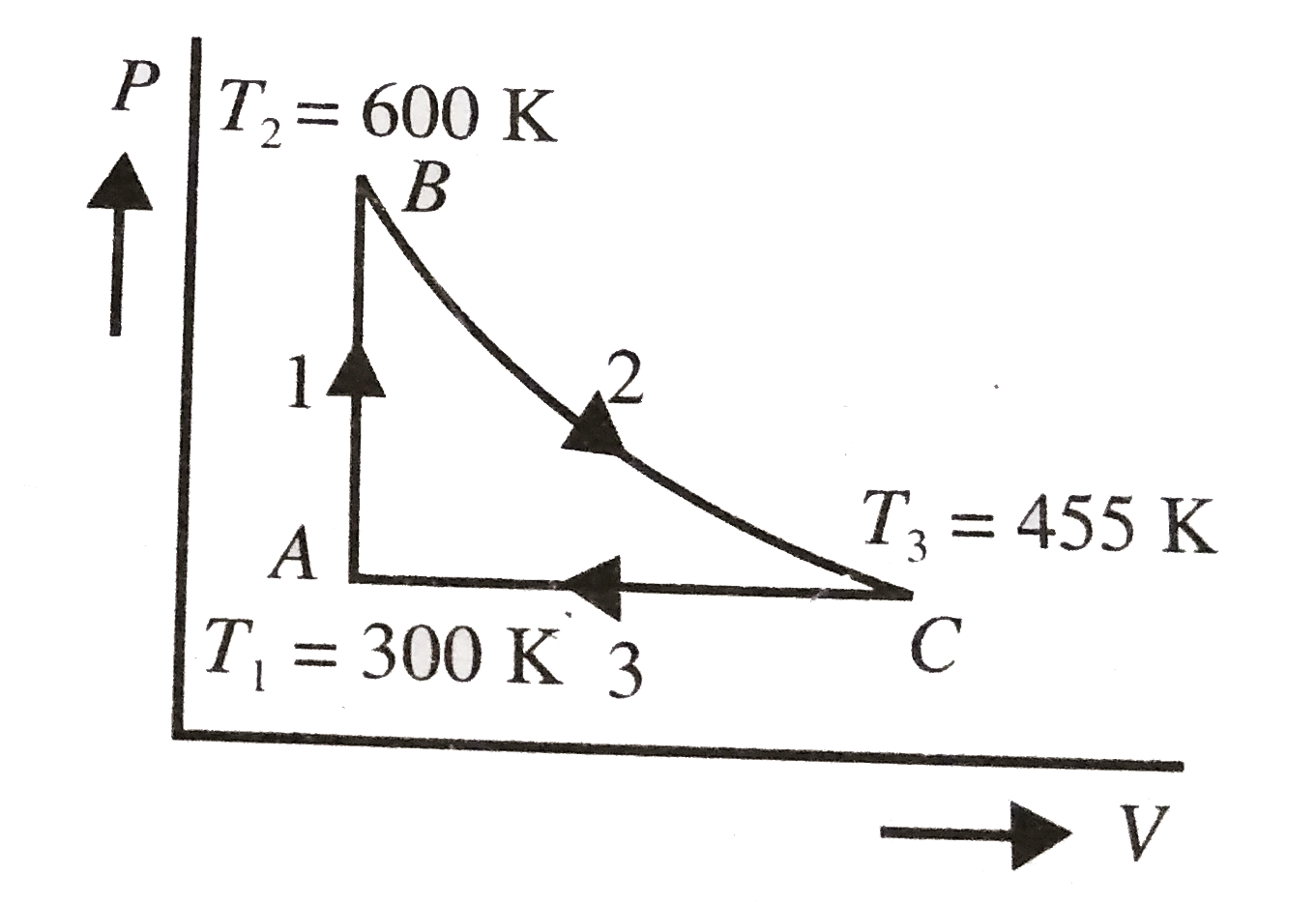
View SolutionA reversible heat engine carries 1mol of an ideal monatomic gas around the cycle ABCA, as shown in the diagram. The process BC is adiabatic. Call the processes AB,BC and CA as 1,2 and 3 and the heat (ΔQ)r, change in internal energy (ΔU), and work done (ΔW)r,r=1,2,3 respectively. The temperature at A,B,C are T1=300K,T2=600K and T3=455K. Indicate the pressure and volume at A,B andC by Pr and Vr,r=1,2,3, respectively. Assume that intially pressure P1=1.00atm.
Which of the following represents the correct values for the quantities indicated ?
View SolutionTwo moles of a diatomic gas are carried trhough the cycle ABCDA as shown in figure. The segment AB represents an isothermal expansion, the segment BC an adiabatic expansion. The pressure and temperature at A are 5 atm and 600K respectively. The volume at B is twice that at A. The pressure at D is 1 atm.

What is the pressure at B?
View SolutionTwo moles of a diatomic gas are carried trhough the cycle ABCDA as shown in figure. The segment AB represents an isothermal expansion, the segment BC an adiabatic expansion. The pressure and temperature at A are 5 atm and 600K respectively. The volume at B is twice that at A. The pressure at D is 1 atm.
What is the temperature at C?
View SolutionOne mole of a monoatomic ideal gas is taken through the cycle ABCDA as shown in the figure.
TA=1000K and 2pA=3pB=6pC

[Assume(23)0.4=0.85 and R=253JK−1mol−1]
The temperature at B is
View Solution
