24 identical cells, each of internal resistance 0.5Ω, are arranged in a parallel combination of n rows, each row containing m cells in series. The combination is connected across a resistor of 3Ω. In order to send maximum current through the resistor, we should have
m=12, n=2
m=8, n=3
m=2, n=2
m=3, n=8
The correct Answer is:A
N=24=mn
For current to be maximum
RInternal=Rexternal
⇒mrn=3⇒mn(0.5)=3⇒mn=6
m=6n, substituting the values, we get
24=6n2
⇒n=2⇒m=12
Topper's Solved these Questions
- BOOK - BITSAT GUIDECHAPTER - CURRENT ELECTRICITYEXERCISE - Bitsat Archives26videos
CURRENT ELECTRICITY
- BOOK - BITSAT GUIDECHAPTER - CATHODE RAY, PHOTOELECTRIC EFFECT AND X-RAYSEXERCISE - BITSAT Archives6videos
CATHODE RAY, PHOTOELECTRIC EFFECT AND X-RAYS
- BOOK - BITSAT GUIDECHAPTER - ELECTRIC CAPACITOREXERCISE - BITSAT archives6videos
ELECTRIC CAPACITOR
Similar Questions
12 cells, each of emf 1.5 V and internal resistance of 0.5Ω, are arranged in m rows each containing n cells connected in series, as shown. Calculate the values of n and m for which this combination would send maximum current through an external resistance of 1.5Ω.
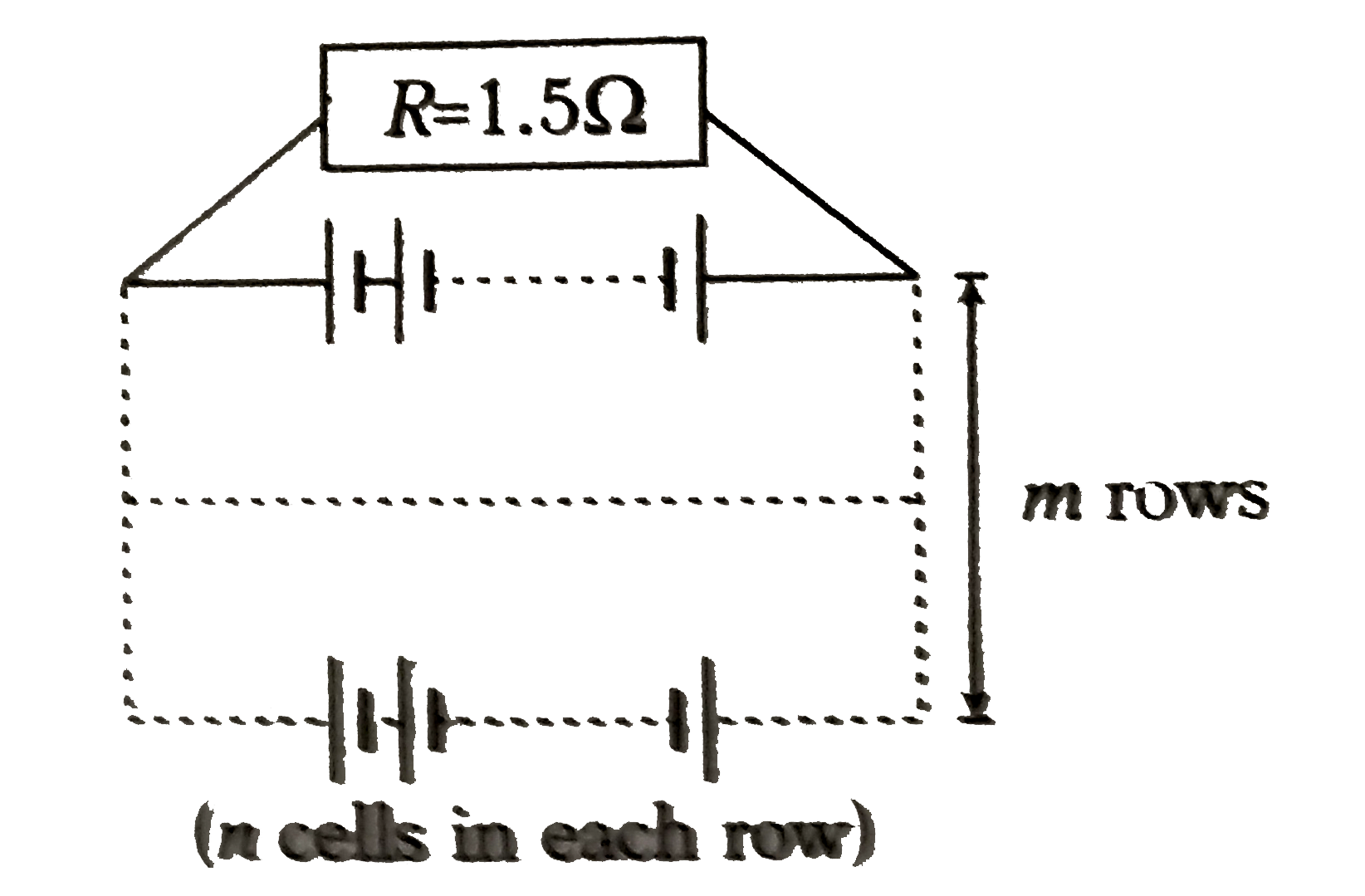 View Solution
View SolutionA battery of 6 V internal resistance 0.5Ω is joined in parallel with another of 10 V and internal resistnace 1Ω. The combination sends a current through an external resistance of 12Ω. Find the current through each battery.
View Solution
Knowledge Check
- Question 1 - Select One
A parallel combination of two resistors, of 1Ω each, is connected in series with a 1.5Ω resistor. The total combination is connected across a 10 V battery. The current flowing in the circuit is
A5AB20AC0.2AD0.4A - Question 2 - Select One
n identical cells each of e.m.f. E and internal resistance r are connected in series. An external resistance R is connected in series to this combination. The current through R is
AnER+nrBnEnR+rCER+nrDnER+r - Question 3 - Select One
4 cells each of emf 2 V and internal resistance of 1Ω are connected in parallel to a load resistor of 2Ω. Then the current through the load resistor is
A2AB1.5AC1AD0.888A
Three identical cells of emf 2 V and internal resistances 0.20Ω are connected in series. The combination is further connected to an external resistor of 6Ω . Calculate the current through 6Ω resistor.
View SolutionA battery of emf 6V internal resistance 5Ω is joined in parallel with another cell of emf of 10V and internal resistance 1Ω and the combination is used to send current through a resistor of 12Ω. Calculate the current through each battery.
View Solution100 cells each of emf 5V and internal resistance 1Ω are to be arranged to produce maximum current in a 25Ω resistance. Each row contains equal number of cells. Find the number of rows.
View SolutionFive identical cells each of internal resistance 1Ω and emf 5V are connected in series and in parallel with an external resistance 'R' . For what value of 'R', current in series and parallel combination will remain the same ?
View SolutionThree identical cells, each of emf 2 V and internal resistance 0.2Ω are connected in series to an external resistor of 7.4Ω. Calculate the current in the circuit.
View Solution
