A 50 g bullet moving with velocity 10 m / s strikes a block of mass 950 g at rest and gets embedded in it. The loss in kinetic energy will be
1
2.375
0.05
0.5
The correct Answer is:B
To solve the problem of finding the loss in kinetic energy when a bullet embeds itself in a block, we can follow these steps:
Step 1: Convert the masses into kilograms
The mass of the bullet is given as 50 g, and the mass of the block is 950 g. We need to convert these masses into kilograms for consistency in SI units.
- Mass of bullet, mb=50g=0.050kg
- Mass of block, mB=950g=0.950kg
Step 2: Calculate the initial kinetic energy of the bullet
The initial kinetic energy (KE) of the bullet can be calculated using the formula:
KE=12mv2
where m is the mass and v is the velocity.
- Velocity of bullet, vb=10m/s
Calculating the initial kinetic energy of the bullet:
KEinitial=12×0.050kg×(10m/s)2
KEinitial=12×0.050×100=2.5J
Step 3: Calculate the final velocity after the collision
Since the bullet embeds itself in the block, we can use the conservation of momentum to find the final velocity vf after the collision.
The initial momentum of the system (only the bullet is moving):
Initial Momentum=mb⋅vb+mB⋅0=0.050⋅10+0.950⋅0=0.5kg m/s
The total mass after the collision:
mtotal=mb+mB=0.050+0.950=1.000kg
Using conservation of momentum:
Initial Momentum=Final Momentum
0.5=1.000⋅vf
vf=0.5m/s
Step 4: Calculate the final kinetic energy of the combined system
Now we can calculate the final kinetic energy of the system (bullet + block) using the final velocity vf:
KEfinal=12mtotalv2f
KEfinal=12×1.000kg×(0.5m/s)2
KEfinal=12×1.000×0.25=0.125J
Step 5: Calculate the loss in kinetic energy
The loss in kinetic energy can be found by subtracting the final kinetic energy from the initial kinetic energy:
Loss in KE=KEinitial−KEfinal
Loss in KE=2.5J−0.125J=2.375J
Final Answer
The loss in kinetic energy is 2.375J.
---
The mass of the bullet is given as 50 g, and the mass of the block is 950 g. We need to convert these masses into kilograms for consistency in SI units.
- Mass of block, mB=950g=0.950kg
The initial kinetic energy (KE) of the bullet can be calculated using the formula:
KE=12mv2
where m is the mass and v is the velocity.
KEinitial=12×0.050kg×(10m/s)2
KEinitial=12×0.050×100=2.5J
Since the bullet embeds itself in the block, we can use the conservation of momentum to find the final velocity vf after the collision.
Initial Momentum=mb⋅vb+mB⋅0=0.050⋅10+0.950⋅0=0.5kg m/s
mtotal=mb+mB=0.050+0.950=1.000kg
Initial Momentum=Final Momentum
0.5=1.000⋅vf
vf=0.5m/s
Now we can calculate the final kinetic energy of the system (bullet + block) using the final velocity vf:
KEfinal=12mtotalv2f
KEfinal=12×1.000kg×(0.5m/s)2
KEfinal=12×1.000×0.25=0.125J
The loss in kinetic energy can be found by subtracting the final kinetic energy from the initial kinetic energy:
Loss in KE=KEinitial−KEfinal
Loss in KE=2.5J−0.125J=2.375J
The loss in kinetic energy is 2.375J.
Topper's Solved these Questions
Similar Questions
A bullet of mass m moving with velocity u on smooth surface strikes a block of mass M kept at rest. The collision is completely inelastic. Find the common velocity and the fractional loss in kinetic energy.
View SolutionIn the given diagram a 0.1 kg bullet moving with speed 20 m/sec strikes 1.9kg mass and get embedded in it. find the kinetic energy of the mass with which it will strikes the ground is
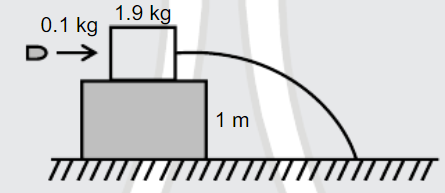
View Solution
Knowledge Check
A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
A12mv2×m(m+M)B12mv2×M(m+M)C12mv2×(M+m)mD12Mv2×m(m+M)A bullet of mass m moving with velocity v strikes a block of mass M at rest and gets embedded into it. The kinetic energy of the composite block will be
A12mv2×m(m+M)B12mv2×M(m+M)C12mv2×(M+m)MD12mv2(M+m)mA bullet of mass 'x' moves with a velocity y, hits a wooden block of mass z at rest and gets embedded in it. After collision, the wooden block and bullet moves with a velocity
Axx+zyBx+yxyCzx+yyDx+yzy
A bullet of mass 50 grams going at a speed of 200ms−1 strikes a wood block of mass 950gm and gets embedded in it. The velocity of the block after the impact is
View SolutionA bullet of mass m travelling with a speed v hits a block of mass M initially at rest and gets embedded in it. The combined system is free to move and there is no other force acting on the system. The heat generated in the process will be
View SolutionA bullet of mass in travelling with a speed v hits a block of mass M initially at rest and gets embedded in it. The combined system is free to move and there is no other force acting on the system. The heat generated in the process will be
View SolutionA bullet of mass m moving horizontally with a velocity v strikes a block of wood of mass M and gets embedded in the block. The block is suspended from the ceiling by a massless string. The height to which block rises is
View SolutionA bullet of mass m moving with a speed v strikes a wooden block of mass M & gets embedded into the block. The final speed is
View Solution
