A ball moving with velocity u strikes a smooth horizontal floor at an angle with the normal to the surface at the point of contact . After collision , ball moves with velocity v at an angle with the normal . Assume e as the coefficient of restitution .
Impulse applied by the surface on the ball is mu(1+e)cos
v=
The correct Answer is:A, B, C, D
To solve the problem, we will analyze the collision of the ball with the smooth horizontal floor, applying the principles of momentum and the coefficient of restitution.
1. Identify the Components of Velocity:
- The ball strikes the floor with an initial velocity u at an angle α with the normal.
- The components of the initial velocity u can be expressed as:
- ux=ucosα (component along the normal)
- uy=usinα (component parallel to the surface)
2. Post-Collision Velocity Components:
- After the collision, the ball moves with a velocity v at an angle β with the normal.
- The components of the final velocity v are:
- vx=vcosβ (component along the normal)
- vy=vsinβ (component parallel to the surface)
3. Apply the Coefficient of Restitution:
- The coefficient of restitution e is defined as the ratio of the relative speed after collision to the relative speed before collision along the normal.
- Therefore, we can write:
e=vxux=vcosβucosα
- Rearranging gives us:
vcosβ=eucosα(Equation 1)
4. Conservation of Momentum in the Horizontal Direction:
- Since no external forces act along the horizontal direction (the surface is smooth), the horizontal component of momentum is conserved:
uy=vy
- This gives us:
usinα=vsinβ(Equation 2)
5. Square and Add the Equations:
- Square both equations:
(usinα)2=(vsinβ)2(from Equation 2)
(vcosβ)2=(eucosα)2(from Equation 1)
- Adding these two equations:
u2sin2α+e2u2cos2α=v2sin2β+v2cos2β
- Since sin2β+cos2β=1, we can simplify:
u2(sin2α+e2cos2α)=v2
6. Solve for v:
- Rearranging gives us:
v2=u2(sin2α+e2cos2α)
- Taking the square root:
v=u√sin2α+e2cos2α
Final Result:
The final velocity v of the ball after the collision is given by:
v=u√sin2α+e2cos2α
- The ball strikes the floor with an initial velocity u at an angle α with the normal.
- The components of the initial velocity u can be expressed as:
- ux=ucosα (component along the normal)
- uy=usinα (component parallel to the surface)
- After the collision, the ball moves with a velocity v at an angle β with the normal.
- The components of the final velocity v are:
- vx=vcosβ (component along the normal)
- vy=vsinβ (component parallel to the surface)
- The coefficient of restitution e is defined as the ratio of the relative speed after collision to the relative speed before collision along the normal.
- Therefore, we can write:
e=vxux=vcosβucosα
- Rearranging gives us:
vcosβ=eucosα(Equation 1)
- Since no external forces act along the horizontal direction (the surface is smooth), the horizontal component of momentum is conserved:
uy=vy
- This gives us:
usinα=vsinβ(Equation 2)
- Square both equations:
(usinα)2=(vsinβ)2(from Equation 2)
(vcosβ)2=(eucosα)2(from Equation 1)
- Adding these two equations:
u2sin2α+e2u2cos2α=v2sin2β+v2cos2β
- Since sin2β+cos2β=1, we can simplify:
u2(sin2α+e2cos2α)=v2
- Rearranging gives us:
v2=u2(sin2α+e2cos2α)
- Taking the square root:
v=u√sin2α+e2cos2α
The final velocity v of the ball after the collision is given by:
v=u√sin2α+e2cos2α
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (D. Multiple Choice Questions)
10 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (Assertion Reason Type Questions)
13 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (JEE (Advanced) for IIT Entrance)
7 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A ball strikes a smooth horizontal floor at an angle α with the normal to the surface and after rebounding from the floor , ball moves at an angle β with the normal . Find the relation between α and β if coefficient of elasticity is e .
View SolutionA ball moving with speed v collides with a horizontal smooth surface at an angle θ with normal to surface as shown in figure. If coefficient of restitution of collision is e, then find v'
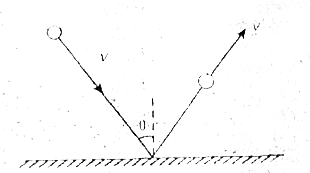
View Solution
Knowledge Check
A ball strikes a smooth horizontal ground at an angle of 45∘ with the vertical. What cannot be the possible angle of its velocity with the vertical after the collision. (Assume e ≤ 1).
A45°B30°C53°D60°A ball strikes a wall with a velocity →u at an angle θ with the normal to the wall surface. and rebounds from it at an angle β with the surface. Then
A(θ+β)<90∘ if the wall is smoothBif the wall is rough , coefficient of restitution =tanβ/cosθCif the wall is rough, coefficient of restition
<tanβ/cotθDnone of theseA ball strikes a smooth horizontal ground at an angle of 45∘ with the vertical. What cannot be the possible angle of its velocity with the vertical after the collisioin. (Assume e≤1).
A45∘B30∘C53∘D60∘
A small ball of mass m collides with as rough wall having coefficient of friction μ at an angle θ with the normal to the wall. If after collision the ball moves with angle α with the normal to the wall and the coefficient of restitution is e, then find the reflected velocity v of the ball just after collision.

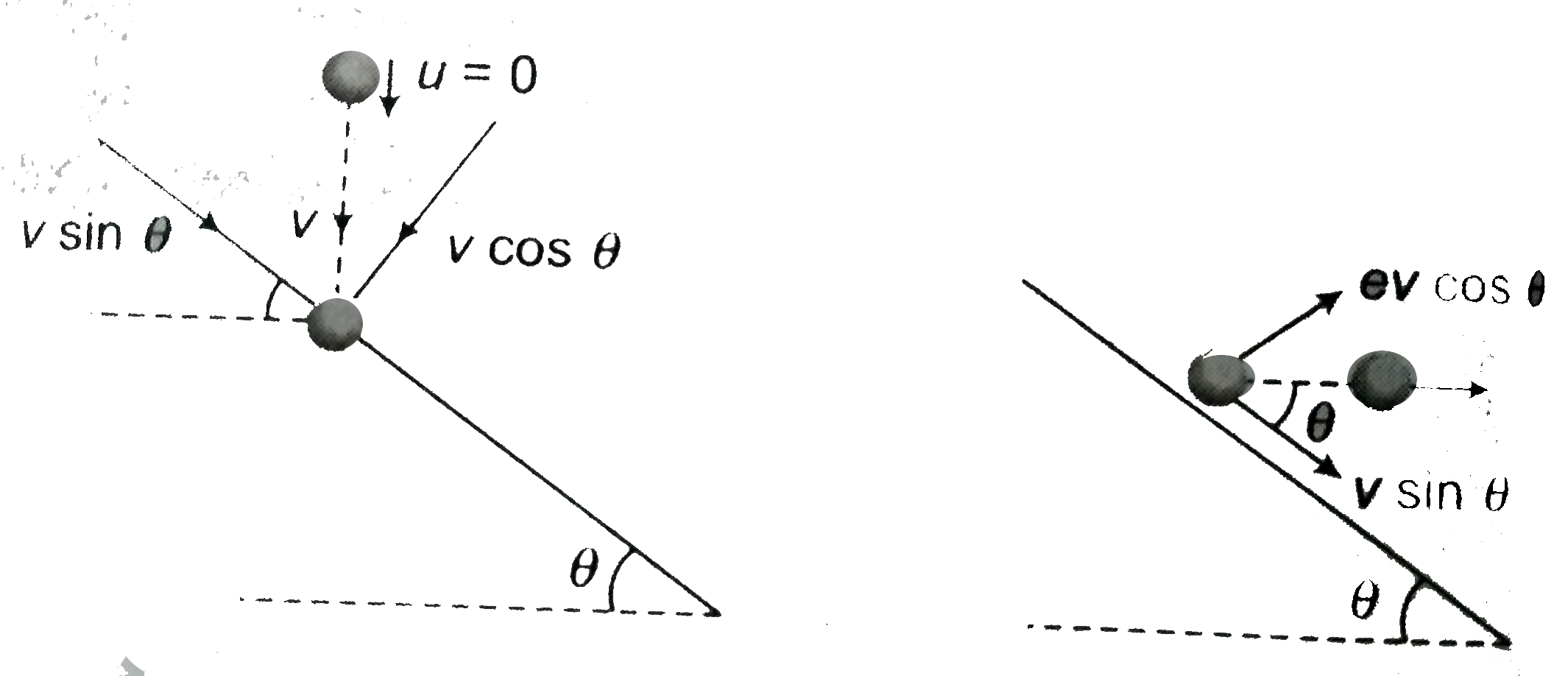
View SolutionA ball after falling a distance collides an inclined plane of inclination θ. If after the collision , the ball moves horizontally , find the coefficient of restitution.
View SolutionA particle of mass m strikes a horizontal smooth floor with velocity u making an angle θ with the floor and rebound with velocity v making an angle θ with the floor. The coefficient of restitution between the particle and the floor is e. Then
View SolutionA ball strickes a horizontal floor at an angle θ=45∘ with the normal to floor. The coefficient of restitution between the ball and the floor is e=1/2. The function of its kinetic energy lost in the collision is.
View SolutionA particle strikes a horizontal frictionless floor with a speed 'u' at an angle 'theta' with the vertical and rebounds with a speed 'v' at an angle 'alpha' with the vertical. Find the value of 'v' if 'e' is the coefficient of restitution.
View Solution
