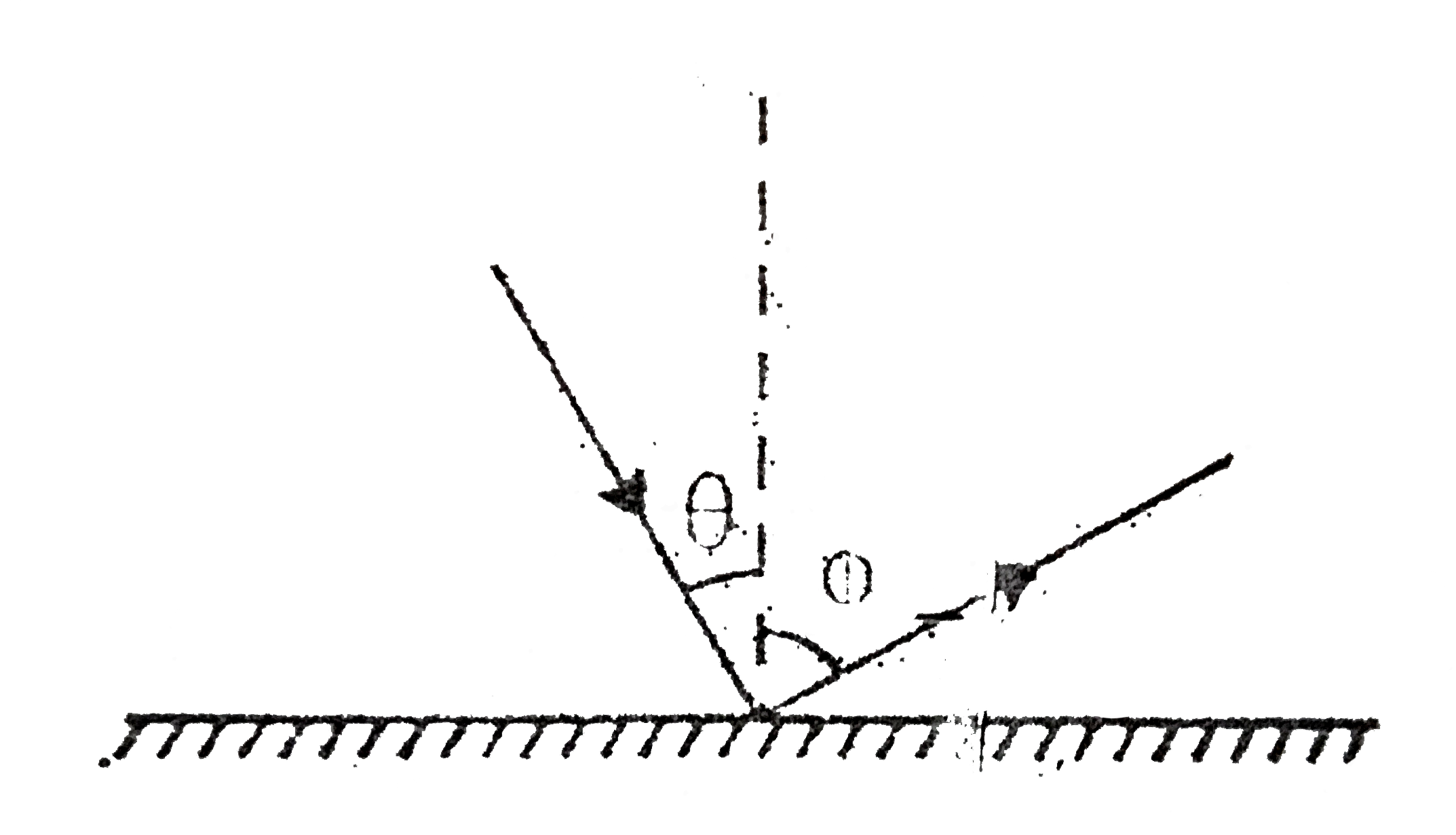
A ball strikes a smooth horizontal floor at an angle $α$ with the normal to the surface and after rebounding from the floor , ball moves at an angle $β$ with the normal . Find the relation between $α$ and $β$ if coefficient of elasticity is e .

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the relation between the angles of incidence (α) and reflection (β) of a ball rebounding off a smooth horizontal floor with a coefficient of restitution (e), we can follow these steps:
1. Understanding the Problem:
- A ball strikes the floor at an angle α with the normal (the line perpendicular to the surface).
- After rebounding, it moves at an angle β with the normal.
- The coefficient of restitution (e) relates the velocities before and after the collision.
2. Components of Velocity:
- Let the initial velocity of the ball be $u$ .
- The vertical component of the velocity before the impact is $u sin α$ .
- The horizontal component of the velocity before the impact is $u cos α$ .
- After rebounding, the vertical component of the velocity is $v sin β$ and the horizontal component is $v cos β$ .
3. Applying the Conservation of Momentum:
- Since the floor is smooth, the horizontal momentum is conserved. Therefore, the horizontal components of the velocities before and after the collision are equal:
$u cos α = v cos β$
- This gives us our first equation.
4. Using the Coefficient of Restitution:
- The coefficient of restitution (e) is defined as the ratio of the relative speed after the collision to the relative speed before the collision along the normal direction:
$e = \frac{Velocity of separation}{Velocity of approach} = \frac{v sin β}{u sin α}$
- Rearranging this gives:
$v sin β = e \cdot u sin α$
5. Combining the Equations:
- From the first equation, we can express $v$ in terms of $u$ :
$v = \frac{u cos α}{cos β}$
- Substitute this expression for $v$ into the equation for the coefficient of restitution:
$\frac{u cos α}{cos β} sin β = e \cdot u sin α$
- Cancel $u$ from both sides (assuming $u \neq 0$ ):
$\frac{cos α sin β}{cos β} = e sin α$
6. Rearranging to Find Relation:
- Rearranging gives:
$tan β = \frac{e sin α}{cos α}$
- This simplifies to:
$tan β = e tan α$
Final Relation:
Thus, the relation between the angles α and β is:
$tan β = e tan α$

Updated on:18/9/2024

A ball moving with velocity u strikes a smooth horizontal floor at an angle $α$ with the normal to the surface at the point of contact . After collision , ball moves with velocity v at an angle $β$ with the normal . Assume e as the coefficient of restitution .
A $tan α = e tan β$
BImpulse applied by the surface on the ball is mu(1+e)cos
C $v = u \sqrt{({sin}^{2} α + e^{2} {cos}^{2} α)}$
Dv= $tan α$
A ball strikes a smooth horizontal floor obliquely and rebounds inelastically.
AThe kinetic energy of the ball just after hitting the floor is equal to the potential energy of the ball at its maximum height alter rebound.
BTotal energy of the ball is not conserved.
CThe angle of rebound with the vertical is greater than the angle of incidence.
DNone of the above.
A ball strikes a wall with a velocity $\to u$ at an angle $θ$ with the normal to the wall surface. and rebounds from it at an angle $β$ with the surface. Then
A $(θ + β) < 90^{\circ}$ if the wall is smooth
Bif the wall is rough , coefficient of restitution $= tan β / cos θ$
Cif the wall is rough, coefficient of restition
$< tan β / cot θ$
Dnone of these