A ball of density d is dropped on to a horizontal solid surface. It bounces elastically from the surface and returns to its original position in a time $t_{1}$ . Next, the ball is released and it falls through the same height before striking the surface of a liquid of density of $d_{L}$
(a) If $d < d_{L}$ , obtain an expression (in terms of d, $t_{1}$ and $d_{L}$ ) for the time $t_{2}$ the ball takes to come back to the position from which it was released.
(b) Is the motion of the ball simple harmonic?
(c) If $d = d_{L}$ , how does the speed of the ball depend on its depth inside the liquid? Neglect all frictional and other dissipative forces. Assume the depth of the liquid to be large.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

Let the ball be dropped from a height h. During fall $V = u t + a t = 0 + \frac{>_{1}}{2} \Rightarrow t_{1} = \frac{2 v}{g}$
In the second case the ball is made to fall through the same height and then the ball strikes the surface of liquid of density $d_{L}$ . When the ball reaches inside the liquid, it is under the influence of two force (i) $V d g$ , the weight of ball in downward direction (ii) $V d_{L} g$ , the upthrust in upward direction.
The viscous forces are absent. (given)
Since, $d_{L} > d$
the upward force is greater and the ball starts retarding.
For motion B to C
$u = V$ , $v = 0$ , $t = t$ , $a = - a$
$v = u + a t = 0 = v + (- a) t$
$\Rightarrow t = \frac{v}{a}$
Now, $a = \frac{F_{n e t}}{m}$
$= \frac{V d_{L} g - V d g}{V d} = \frac{(d_{L} - d) g}{d}$
$\Rightarrow t = \frac{v d}{(d_{L} - d) g}$ ...(iii)
Therefore,
$t_{2} = t_{1} + 2 t = t_{1} + \frac{2 d v}{(d_{L} - d) g}$
$= t_{1} + \frac{2 d}{(d_{L} - d) g} \frac{t_{1} g}{2} = t_{1} [1 + \frac{d}{d_{L} - d}]$
$\Rightarrow t_{2} = \frac{d_{L} t_{1}}{d_{L} - d}$
(b) Since the retardation is not proportional to displacement, the motion of the ball is not simple harmonic.
(c) If $d = d_{L}$ then the retardation $a = 0$ . Since the ball strikes the water surface with some velocity, it will continue with same velocity in downward direction(until it is interrupted by some other force).

|

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

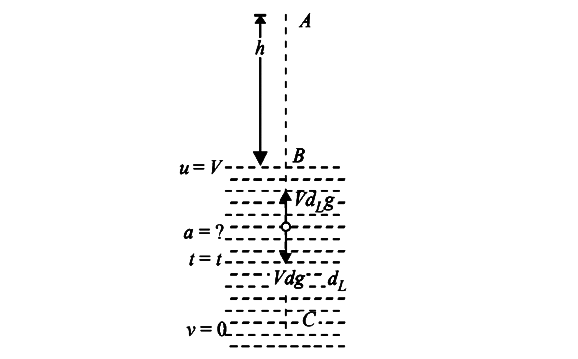
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A ball of density d is dropped on to a horizontal solid surface. It bounces elastically from the surface and returns to its original position in a time $t_{1}$ Next, the ball is released and its falls through the same height before striking the surface of a liquid of density $d_{L}$ . Neglect all frictional and other dissipative force. Assume the depth of the liquid to be large.
If $d < d_{L}$ obtain an expression (in terms of d, $t_{1} and d_{L}$ ) for the time $t_{2}$ the ball takes to come back to the position from which it was released.
A $2 t_{1}$
B $\frac{{d t}_{1}}{(d_{L} - d)}$
C $\frac{{d t}_{1}}{2 (d_{L} - d)}$
D $\frac{2 {d t}_{1}}{(d_{L} - d)}$
Question 2 - Select One
A ball is dropped from the certain height on the surface of glass. It is collide elastically the comes back to initial position. If this process it repeated then velocity time graph is:-
A
B
C
D
Question 3 - Select One
The fraction of a floating object of volume $V_{0}$ and density $d_{0}$ above the surface of a liquid of density d will be
A $\frac{d_{0}}{d}$
B $\frac{d d_{0}}{d + d_{0}}$
C $\frac{d - d_{0}}{c}$
D $\frac{d d_{0}}{d . d_{0}}$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-MECHANICAL PROPERTIES OF MATTER AND FLUIDS-Subjective Problems

Home
Ask Doubt
Profile