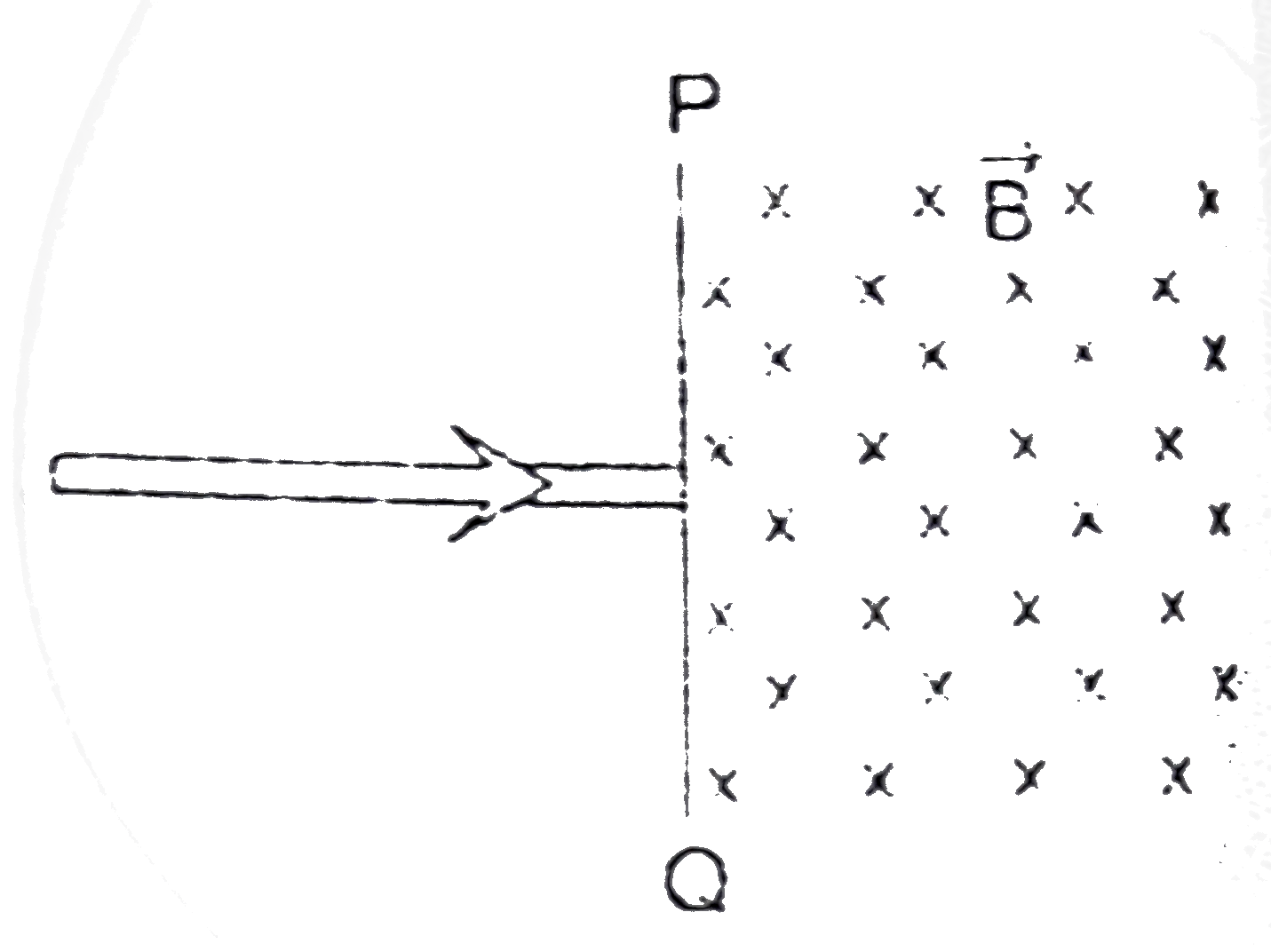
A beam of equally charged particles after being accelerated through a voltage V enters into a magnetic field B as shown in Fig. 1.31. It is found that all the particles hit the plate between C and D. Find the ratio between the masses of the heaviest and lightest particles of the beam.

qV=12mv2⇒v=√2qVm
r=mvqB=mqB√2qVm=√2mVqB2⇒m∝r2
mheavymlight=(1+dl)2
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.2
13 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise1.3
14 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Solved Example
25 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
A beam of mixture of α particle and protons are accelerted through same potential difference before entering into the magnetic field of strength B. if r1=5 cm then r2 is
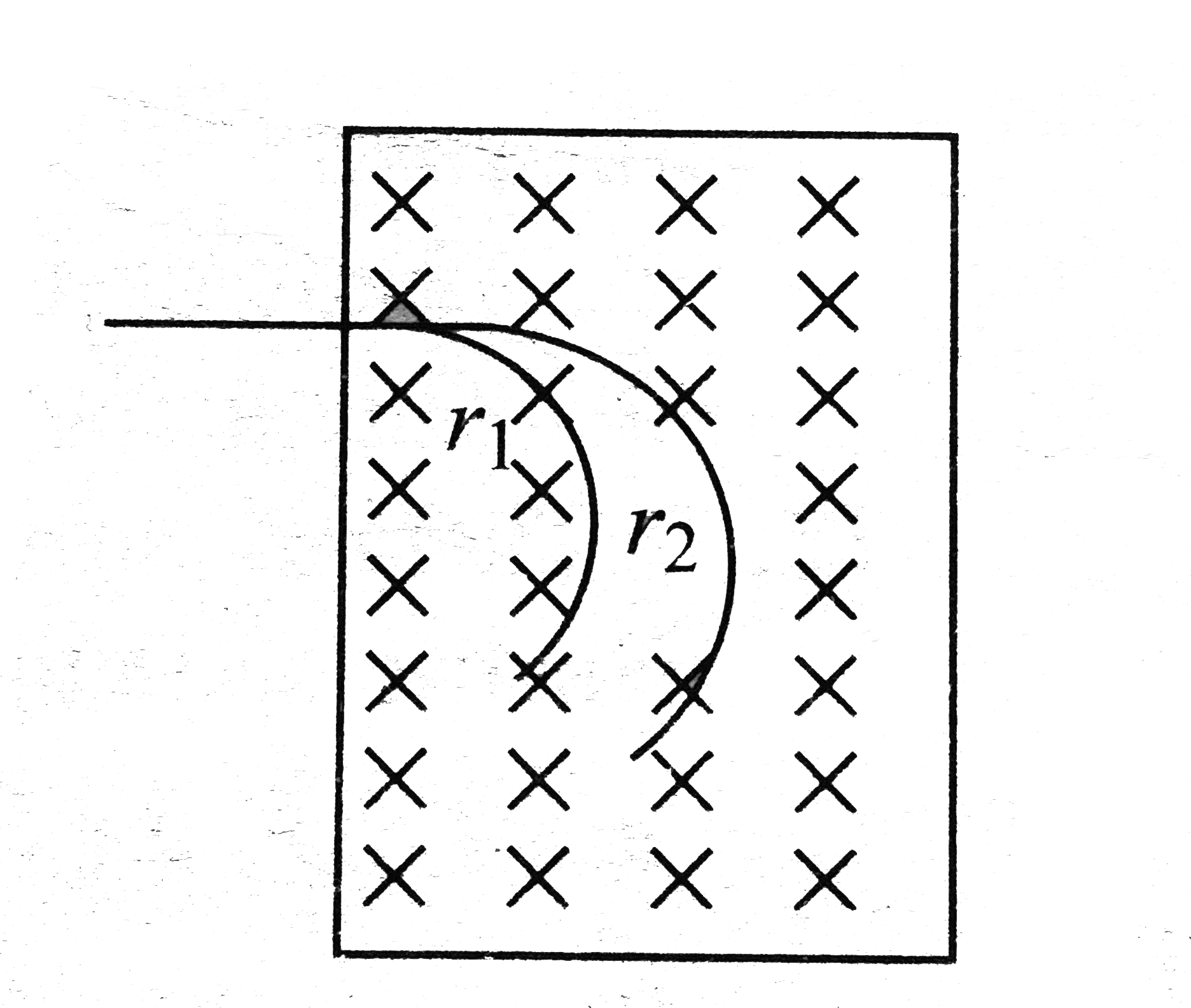
View SolutionA charged particle, having charge q1 accelerated through a potential difference V enters a perpendicular magnetic field in which it experiences a force F. It V is increased to 5V, the particle will experience a force
View Solution
Knowledge Check
Two particles X and Y with equal charges, after being accelerated throuhg the same potential difference, enter a region of uniform magnetic field and describe circular paths of radii R1 and R2 respectively. The ratio of the mass of X to that of Y is
AR1/R2B(R1/R2)2C(R2/R1)D(R2/R2)2
If a charged particle of charge to mass ratio (qm)=α centers in a magnetic field of strength B at a speed v=(2αd)(B), then

View SolutionA thin beam of charged particles in incident normally on the boundry of a region containing a uniform magnetic field as shown.The beam comprise of mono energetic α and β(−) particles, each possessing a kinetic energy T.Neglecting interaction between particles of the beam, find the separation between the points on line PQ where the α and β particles emerge out of the magnetic field.(express your answer in terms of T,B,α(2e,mα)&β−(−e,me),where all terms have their usual meanings.)
View SolutionTwo particle X and Y having equal charge, after being accelerated through the same potential difference enter a region of uniform magnetic field and describe circular paths of radii R1andR2 respectively. The ratio of the mass of X to that of Y is
View SolutionA proton, a deutron and an alpha particle, after being accelerated through the same potential difference enter a region of uniform magnetic field →B, in a direction perpendicular to →B. Find the ratio of their kinetic energies. If the radius of proton's circular path is 7cm, what will be the radii of the paths of deutron and alpha particle?
View SolutionTwo particles X and Y with equal charges, after being accelerated throuhg the same potential difference, enter a region of uniform magnetic field and describe circular paths of radii R1 and R2 respectively. The ratio of the mass of X to that of Y is
View SolutionTwo particles X and Y with equal charges, after being accelerated throuhg the same potential difference, enter a region of uniform magnetic field and describe circular paths of radii R1 and R2 respectively. The ratio of the mass of X to that of Y is
View SolutionTwo particles X and Y with equal charges, after being accelerated throuhg the same potential difference, enter a region of uniform magnetic field and describe circular paths of radii R1 and R2 respectively. The ratio of the mass of X to that of Y is
View Solution
