A biconvex lens of focal length 20cm, made of glass or refractive index 1.5, has water on one side and air on the other. An object is placed 15 cm form the lens on the side with water. Where is the image formed?
To solve the problem, we will follow these steps:
Step 1: Identify the given values
- Focal length of the lens (F) = 20 cm
- Refractive index of the lens (μ) = 1.5
- Refractive index of water (μ1) = 1.33
- Refractive index of air (μ2) = 1.0
- Object distance from the lens (U) = -15 cm (the object is on the same side as the water)
Step 2: Calculate the radius of curvature (R)
For a biconvex lens, the formula for the focal length (F) is given by:
1F=(μ−1)(1R1−1R2)
Since it is a biconvex lens, we can assume R1=R and R2=−R:
1F=(1.5−1)(1R+1R)
This simplifies to:
1F=0.5R
Given F=20 cm, we have:
120=0.5R
Thus,
R=10 cm
Step 3: Use the lens maker's formula for two media
The lens formula for two different media is given by:
μ2v−μ1u=μ2−μ1R
Substituting the values:
- μ2=1 (for air)
- μ1=1.33 (for water)
- u=−15 cm
- R=10 cm
The equation becomes:
1v−1.33−15=1−1.3310
This simplifies to:
1v+1.3315=−0.3310
Step 4: Solve for v
First, calculate 1.3315:
1.3315≈0.08867
Now substituting back into the equation:
1v+0.08867=−0.033
Rearranging gives:
1v=−0.033−0.08867≈−0.12167
Thus,
v≈−8.22 cm
Step 5: Interpret the result
The negative value of v indicates that the image is formed on the same side as the object, which means it is a virtual image.
Final Answer
The image is formed approximately 8.22 cm from the lens on the side of the water.
---
- Focal length of the lens (F) = 20 cm
- Refractive index of the lens (μ) = 1.5
- Refractive index of water (μ1) = 1.33
- Refractive index of air (μ2) = 1.0
- Object distance from the lens (U) = -15 cm (the object is on the same side as the water)
For a biconvex lens, the formula for the focal length (F) is given by:
1F=(μ−1)(1R1−1R2)
Since it is a biconvex lens, we can assume R1=R and R2=−R:
1F=(1.5−1)(1R+1R)
This simplifies to:
1F=0.5R
Given F=20 cm, we have:
120=0.5R
Thus,
R=10 cm
The lens formula for two different media is given by:
μ2v−μ1u=μ2−μ1R
Substituting the values:
- μ2=1 (for air)
- μ1=1.33 (for water)
- u=−15 cm
- R=10 cm
1v−1.33−15=1−1.3310
This simplifies to:
1v+1.3315=−0.3310
First, calculate 1.3315:
1.3315≈0.08867
Now substituting back into the equation:
1v+0.08867=−0.033
Rearranging gives:
1v=−0.033−0.08867≈−0.12167
Thus,
v≈−8.22 cm
The negative value of v indicates that the image is formed on the same side as the object, which means it is a virtual image.
The image is formed approximately 8.22 cm from the lens on the side of the water.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.7
21 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.5
22 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
A double convex lens made of glass of refractive index 1.56 has both radii of curvature of magnitude 20cm. If an object is placed at a distance of 10cm from this lens, find the position of image formed.
View SolutionA plane convex lens has a focal length 12cm and is made up of glass with refractive index 1.5. Find the radius of curvature of its curved side.
View SolutionA glass slab of thickness 2cm and refractive index 2 is placed in contact with a biconvex lens of focal length 20cm. The refractive index of the material of the lens 1.5 . The far side of the lens is silvered. Where should an object be placed in front of the slab so that it images on to itself?
View SolutionA biconvex lens has radii of curvature 20 cm and 40cm. The refractive index of the material of the lens is 1.5. An object is placed 40cm in front of the lens. Calculate the psition of the image.
View SolutionA biconvex lens of focal length f1=10cm is placed 40 cm in front of a concave mirror of focal length f2=7.50cm, as shown in the figure. An object, 2 cm high, is placed 20 cm to the left of the lens.
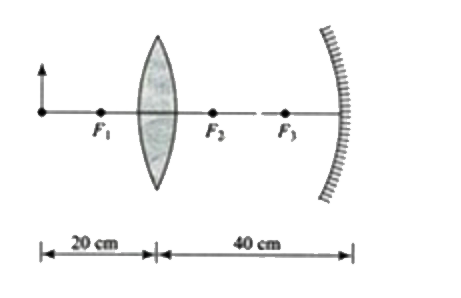
The image formed by the lens, as rays travel to the right, is
View SolutionA thin equiconvex lens has focal length 10 cm and refractive index 1.5 . One of its faces is now silvered and for an object placed at a distance u in front of it, the image coincides with the object. The value of u is
View SolutionA biconvex lens made of glass (refractive index 1.5) has two spherical surfaces having radii 20cm and 30cm. Calculate its focal length.
View SolutionA parallel beam of light is incident on a lens of focal length 10 cm. A parallel slab of refractive index 1.5 and thickness 3 cm is placed on the other side of the lens. Find the distance of the final image from the lens.
 .
.
View SolutionA plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?
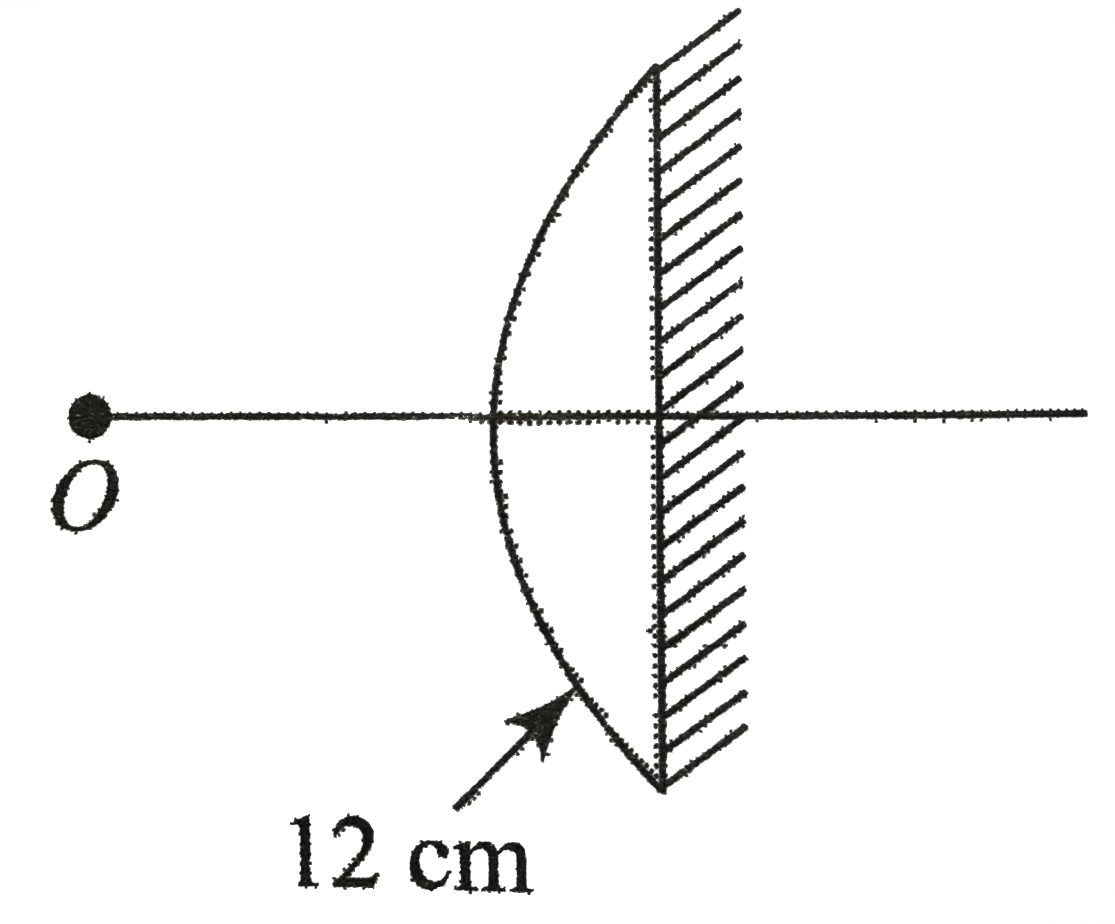
View SolutionA thin biconvex lens of focal length 6.25 cm is made of material of refractive index 1.5. It is cut into two identical pieces perpendicular to its principal axis. One of the pieces is placed in water of refractive index 43. The focal power of the piece immersed in water in diopter is
View Solution
