A bimetallic strip is composed of two identical strips, one of steel and other of brass. When the temperature of the strip is raised by ΔT , it bends to form arc of curvature R. If the coefficients of linear expansion of steel and brass are α1 and α2 , respectively, then R is
directly proportional to |α1−α2|
inversely proportional to |α1−α2|
directly proportional to ΔT
NONE OF THESE
The correct Answer is:B
To solve the problem of the bimetallic strip composed of steel and brass, we need to analyze how the two materials expand differently when the temperature is raised by ΔT. Here’s a step-by-step breakdown of the solution:
Step 1: Understanding the Expansion
When the temperature of the bimetallic strip is raised by ΔT, both materials (steel and brass) will expand. The amount of expansion is determined by their coefficients of linear expansion, denoted as α1 for steel and α2 for brass.
Step 2: Length Change Due to Expansion
The change in length (ΔL) for each material can be expressed as:
- For brass:
Lb=L0b(1+α2ΔT)
- For steel:
Ls=L0s(1+α1ΔT)
Where L0b and L0s are the initial lengths of brass and steel, respectively.
Step 3: Relating Lengths to Radius of Curvature
When the strip bends, the length of the arc formed by each material can be related to the radius of curvature R and the angle θ subtended by the arc:
- For brass:
Lb=Rθ
- For steel:
Ls=Rθ
Step 4: Setting Up the Equations
Since both materials have the same initial length, we can set up the equations for the lengths after expansion:
1. For brass:
Rθ=L0(1+α2ΔT)
2. For steel:
Rθ=L0(1+α1ΔT)
Step 5: Dividing the Equations
To find the relationship between R and the coefficients of linear expansion, we can divide the two equations:
1+α2ΔT1+α1ΔT=R+dR
Where d is the small difference in length due to the different expansions.
Step 6: Applying the Binomial Approximation
Assuming that the coefficients of linear expansion are small, we can use the binomial approximation:
1+α2ΔT1+α1ΔT≈1+(α2−α1)ΔT
Step 7: Finding the Radius of Curvature
From the previous steps, we can derive:
dR≈α2ΔT−α1ΔT
Thus, we can express R as:
R≈d(α2−α1)ΔT
Conclusion
From this derivation, we conclude that the radius of curvature R is inversely proportional to the difference in the coefficients of linear expansion |α1−α2| and also inversely proportional to ΔT.
Final Result
Thus, we can summarize that:
R∝1|α2−α1|ΔT
When the temperature of the bimetallic strip is raised by ΔT, both materials (steel and brass) will expand. The amount of expansion is determined by their coefficients of linear expansion, denoted as α1 for steel and α2 for brass.
The change in length (ΔL) for each material can be expressed as:
- For brass:
Lb=L0b(1+α2ΔT)
- For steel:
Ls=L0s(1+α1ΔT)
When the strip bends, the length of the arc formed by each material can be related to the radius of curvature R and the angle θ subtended by the arc:
- For brass:
Lb=Rθ
- For steel:
Ls=Rθ
Since both materials have the same initial length, we can set up the equations for the lengths after expansion:
1. For brass:
Rθ=L0(1+α2ΔT)
2. For steel:
Rθ=L0(1+α1ΔT)
To find the relationship between R and the coefficients of linear expansion, we can divide the two equations:
1+α2ΔT1+α1ΔT=R+dR
Where d is the small difference in length due to the different expansions.
Assuming that the coefficients of linear expansion are small, we can use the binomial approximation:
1+α2ΔT1+α1ΔT≈1+(α2−α1)ΔT
From the previous steps, we can derive:
dR≈α2ΔT−α1ΔT
Thus, we can express R as:
R≈d(α2−α1)ΔT
From this derivation, we conclude that the radius of curvature R is inversely proportional to the difference in the coefficients of linear expansion |α1−α2| and also inversely proportional to ΔT.
Thus, we can summarize that:
R∝1|α2−α1|ΔT
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTION ((D ) MULTIPLE CHOICE QUESTION BASED ON A GIVEN PASSAGE/COMPREHENSION )9videos
THERMAL PROPERTIES OF MATTER
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - COMPETITION FILE (ASSERTION REASON TYPE QUESTION )10videos
THERMAL PROPERTIES OF MATTER
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTION ((B) MULTIPLE CHOICE QUESTION FROM CPMPETITIVE EXAMINATION ) JEE ADVANCED FOR IIT ENTRANCE )9videos
THERMAL PROPERTIES OF MATTER
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)16videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - UNITS AND MEASUREMENTEXERCISE - CHAPTER PRACTICE TEST15videos
UNITS AND MEASUREMENT
Similar Questions
Knowledge Check
- Question 1 - Select One or More
A bimetallic strip is formed out of two identical strips one of copper and the other of brass. The coefficients of linear expansion of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
Aproportional to ΔTBinversely proportional to ΔTCproportional to |αB−αC|Dinversely proportional to |αB−αC| - Question 2 - Select One
In figure which strip brass or steel have higher coefficient of linear expansion.
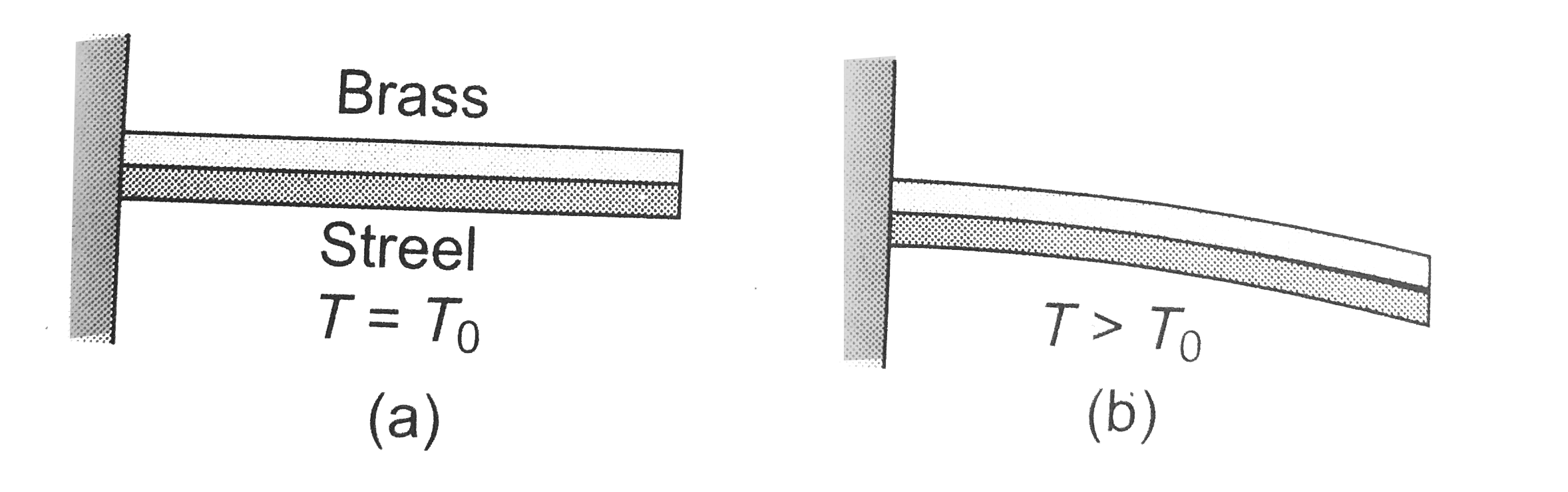 Abrass stripBsteel stripCboth strips has same coefficient of linear expansionDcannot be decided from given data
Abrass stripBsteel stripCboth strips has same coefficient of linear expansionDcannot be decided from given data - Question 3 - Select One
When the temperature of a rod increases from t to t+Δt, its moment of inertia increases from I to I+ΔI. If α is coefficient of linear expansion, the value of ΔI/I is
A2αΔtBαΔtCαΔt/2DΔt/α
Two same length rods of brass and steel of equal cross-sectional area are joined end to end as shown in figure and supported between two rigid vertical walls. Initially the rods are unstrained. If the temperature of system is raised by Δt. Find the displacement of the junction of two rods. Given that the coefficient of linear expansion and young's modulus of brass and steel are aphab.αs(αb>αs),Yb and Ys respectively.
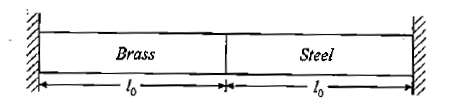 View Solution
View SolutionTwo steel rods and an aluminium rod of equal length l0 and equal cross section are joined rigidly at their ends as shown in the figure below. All the rods are in a state of zero tension at 00C. Find the length of the system when the temperature is raised to θ. Coefficient of linear expansion of aluminium and steel are αaandαs respectively. Young's modulus of aluminium is Ya and of steel is Ys.
steel
Aluminium
Steel
 View Solution
View SolutionA bimetallic strip is formed out of two identical strips one of copper and the other of brass. The co-efficients of linear expansion of the two metals are αC and αB. On heating, the the strip bends to form an are of radius of curvature R. Then R is
View SolutionThe moment of inertia of a rod about its perpendicular bisector is I . When the temperature of the rod is increased by ΔT , the increase in the moment of inertia of the rod about the same axis is (Here , α is the coefficient of linear expansion of the rod )
View SolutionTwo identical thin metal strips one of aluminium and the other of iron are riveted together to form a bimetallic strip. The temperature is raised by 50∘C. If the central planes of the two strips are separated by 2nm and the coefficients of thermal expansion of aluminium and iron are respectively 30×10−6/∘C and 10×10−6/∘C the average radius of curvature of the bimetallic strip is about
View Solution


