Two same length rods of brass and steel of equal cross-sectional area are joined end to end as shown in figure and supported between two rigid vertical walls. Initially the rods are unstrained. If the temperature of system is raised by Δt. Find the displacement of the junction of two rods. Given that the coefficient of linear expansion and young's modulus of brass and steel are aphab.αs(αb>αs),Yb and Ys respectively.
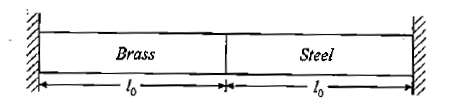
Here the initial lengths of the two rods are l0 and as αb>αs , we can directly state that the junction of the two rods is displacedf toward right. Due to this brass rod is some what expanded but less as compared to free expansion and steel is overall compressed due to the stress developed between the two rods.
Figure shows the final situation of rods at higher temperature Δt. in figure A is the initial position of junction and B is its final position.
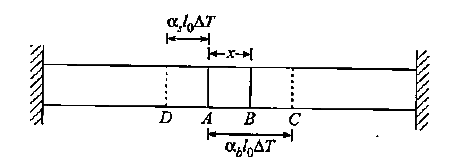
If only brass rod is present, expansion in it due to increase in temperature by ΔT is
Δlb=αbl0ΔT
Which is equal to AC in figure. But finally the rod is expanded by x, thus the elastic compression in the rod due to stress between the two rods is BC, which is given as
BC=αbl0ΔT−x
Thus elastic strain in brass rod is
(Strain)brass=αbl0Δt−xl0[ As αbl0ΔT is small]
Similary if steel rod alone is there, it would have been expanded by
Δls=αsl0ΔT
Which is equal to AD in figure . But finally steel rod is compressed by x, thus the elastic compression in steel rod due to stress between the two rods is BD, which is given as
BD=αs=l0ΔT+x
Thus elastic strain in steel rod is
(Strain)_(steel)=αsl0ΔT−xl0 [As αsl0ΔT is small]
As thetwo rods are in contact, stress develoed in the two rod must be equal thus we have
(Stress)brass=(Stress)steel
or Yb×(Strain)brass=YS×(Strain)steel
or Yb[αblbΔT−xl∘0=Ys[αsl∘ΔT+xl0]
or x=(Ybαb−Ysαs)l∘ΔT(Yb+Ys)
Topper's Solved these Questions
- HEAT AND THERMAL EXPANSIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT AND THERMAL EXPANSIONEXERCISE - PRACTICE EXERCISE
40 Videos
- HEAT AND THERMAL EXPANSIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT AND THERMAL EXPANSIONEXERCISE - DISCUSSION QUESTION
24 Videos
- GEOMETRICAL OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GEOMETRICAL OPTICSEXERCISE - Unsolved Numerical Problems
107 Videos
- HEAT TRANSFER BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - HEAT TRANSFER EXERCISE - Unsolved Numerical Problems for Preparation of NSEP,INPhO&IPhO
66 Videos
Similar Questions
Two rods of material X sandwich another rod of material Y as shown in figure. At temperature t, the three rods are in a state of zero strailn and of length L and riveted to each other in this state. If the temperature of the system increases to T+ΔT, find the final length of the system of the three rods. Given that the coefficients of linear expansions of the roods and their Young's modulus for material X and Y are alpnax,αy,Yx and Yy respectively. Consider αy>αx.

View SolutionTwo rods of different materials but having same length L and cross-sectioinal areas A are arranged end-to-end between fixed supports. The temperature is T and there is no initial stress. The rods are heated so that their temperature increases by ΔT. Find : (a) the displacement of the interface (b) the stress at the interface. The coefficinets of linear expansioin of the materials are α1 and α2 and their Young's modulii are E1 and E2 respectively.
View Solution
Knowledge Check
Two rods of equal cross sections area are joined end the end as shown in figure. These are supported between two rigid vertical walls. Initially the rods are unstrained .
_E01_227_Q01.png)
If temperature of system is increased by ΔT then junction will not shift if -AY1α1=Y2α2BY1α1l1=Y2α2l2Cα1=α2DY2α1l2=Y1α2l2Two rods of equal cross sections area are joined end the end as shown in figure. These are supported between two rigid vertical walls. Initially the rods are unstrained .
_E01_228_Q01.png)
If temperature of system is increased by ΔT then thermal stress developed in first rod-Ais equal to thermal stress developed in second rodBis greater than thermal stress developed in second rodCis less than thermal stress developed in second rodDNone of theseTwo rods of equal cross sections area are joined end the end as shown in figure. These are supported between two rigid vertical walls. Initially the rods are unstrained .
_E01_229_Q01.png)
If temperature of system is increased by ΔT then shifting in junction if junction if Y1α1>Y2α2 is given by -Al1l2(Y1α2−Y2α1)Y1l1+Y2l2Bl1l2(Y1α1−Y2α2)Y1l2+Y2l1Cl1l2(Y1α1−Y2α2)Y1l1+Y2l2DNone of these
Two Aluminium rods and a steel rod of equal cross-sectional area and equal length l0 are joined rigidly side by side as shown in figure. Initially the rods are at 0∘C. Find the length of the rod at the temperature θ if young's modulus of elasticity of the aluminium and steel are Ya and Ys respectively and coefficient of linear expansion of aluminum and steel are αa and αs respectively.
∣∣ ∣∣AluminiumSteelAluminium∣∣ ∣∣
View SolutionFigure shows a steel rod of cross sectional ara 2×10−6m2 is fixed between two vertical walls. Initially at 20∘C there is no force between the ends of the rod and the walls. Find the force which the rod will exert on walls at 100∘C. Given that the coefficient of linear expansion of steel is 1.2×10−5.∘C−1 and Young's modulus is 2×1011N/m2

View SolutionTwo rods of different materials and identical cross sectional area, are joined face to face at one end their free ends are fixed to the rigid walls. If the temperature of the surroundings is increased by 30∘C, the magnitude of the displacement of the joint of the rod is (length of rods l1=l2=1unit, ratio of their young's moduli, Y1/Y2=2, coefficients of linear expansion are α1 and α2)
View SolutionTwo rods of copper and brass having the same length and cross - section are joined end to end. The free end of the copper is at 0∘C and of brass is at 80∘C in steady-state. If thermal conductivity of copper is 4 times of that of brass find the temp. at junction of two rods
View SolutionThree rods A,B and C of the same length and cross- sectional area are joined in series as shown. The temperature at the junction of A and B, in equilibriu, is
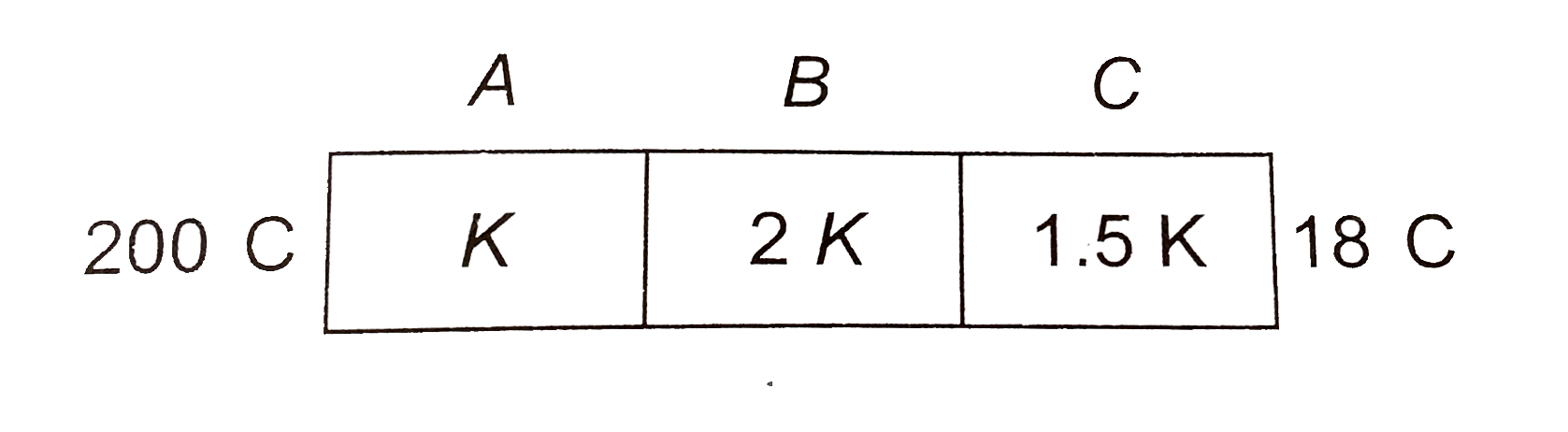
View Solution
