A block is constrained to move along x-axis under a force F=−2x. Here, F is in newton and x in metre. Find the work done by this force when the block is displaced fromx=2m→x=-4 m.
The correct Answer is:A, B
To find the work done by the force F=−2x when the block is displaced from x=2m to x=−4m, we can follow these steps:
Step 1: Understand the Work Done Formula
The work done W by a force is given by the integral of the force over the displacement:
W=∫Fdx
Step 2: Substitute the Force into the Integral
Given the force F=−2x, we can substitute this into the work done formula:
W=∫−42(−2x)dx
Step 3: Set Up the Integral
Now we need to evaluate the integral:
W=−2∫−42xdx
Step 4: Calculate the Integral
The integral of x is:
∫xdx=x22
Thus, we can evaluate the definite integral:
W=−2(x22)−42
Step 5: Evaluate the Limits
Now we need to evaluate this from x=2 to x=−4:
W=−2((−4)22−(2)22)
Calculating the squares:
W=−2(162−42)
This simplifies to:
W=−2(8−2)=−2×6=−12J
Final Answer
The work done by the force when the block is displaced from x=2m to x=−4m is:
W=−12J
---
The work done W by a force is given by the integral of the force over the displacement:
W=∫Fdx
Given the force F=−2x, we can substitute this into the work done formula:
W=∫−42(−2x)dx
Now we need to evaluate the integral:
W=−2∫−42xdx
The integral of x is:
∫xdx=x22
Thus, we can evaluate the definite integral:
W=−2(x22)−42
Now we need to evaluate this from x=2 to x=−4:
W=−2((−4)22−(2)22)
Calculating the squares:
W=−2(162−42)
This simplifies to:
W=−2(8−2)=−2×6=−12J
The work done by the force when the block is displaced from x=2m to x=−4m is:
W=−12J
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.2
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.3
2 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Miscellaneous Example
6 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A block is constrained to move along x-axis under a forc F=4x2(x≠0). Here, F is in newton and x in metre. Find the work done by this force when the block is displaced from x=4 m to x=2m.
View SolutionA force F=(5+2x) acts on a particle in x direction where F is in newtown and x is meter. Find the work done by this force during displacement from x=0 to x=1m.
View SolutionA force F=(2+x) acts on a particle in x-direction where F is in newton and x in metre. Find the work done by this force during a displacement form 1. 0 m to x = 2.0 m.
View SolutionA force F=(2+x) acts on a particle in x-direction where F is in newton and x in meter. Find the work done by this force during a displacement from 1. 0 m to x = 2.0 m.
View SolutionA force F=(5+2x) acts on a particle in x direction where F is in Newtown and x is in meter. Find the work done by this force during displacement from x=0 to x=1m.
View SolutionForce acting on a particle constrained to move along x-axis is F=(x−4). Here, F is in newton and x in metre. Find the equilibrium position and state whether it is stable or unstable equilibrium.
View SolutionA force F=(10+0.50x) acts on a particle in the x direction, where F is in newton and x in meter. Find the work done by this force during a displacement form x=0 to x=2.0m
View SolutionA force F=(10+0.50x) acts on a particle in the x direction, where F is in newton and x in meter./find the work done by this force during a displacement form x=0 tox=2.0m
View SolutionA Force F acting on an object varies with distance x as shown in the here . The force is in newton and x in metre. The work done by the force in moving the object from x=0 to x=6m is
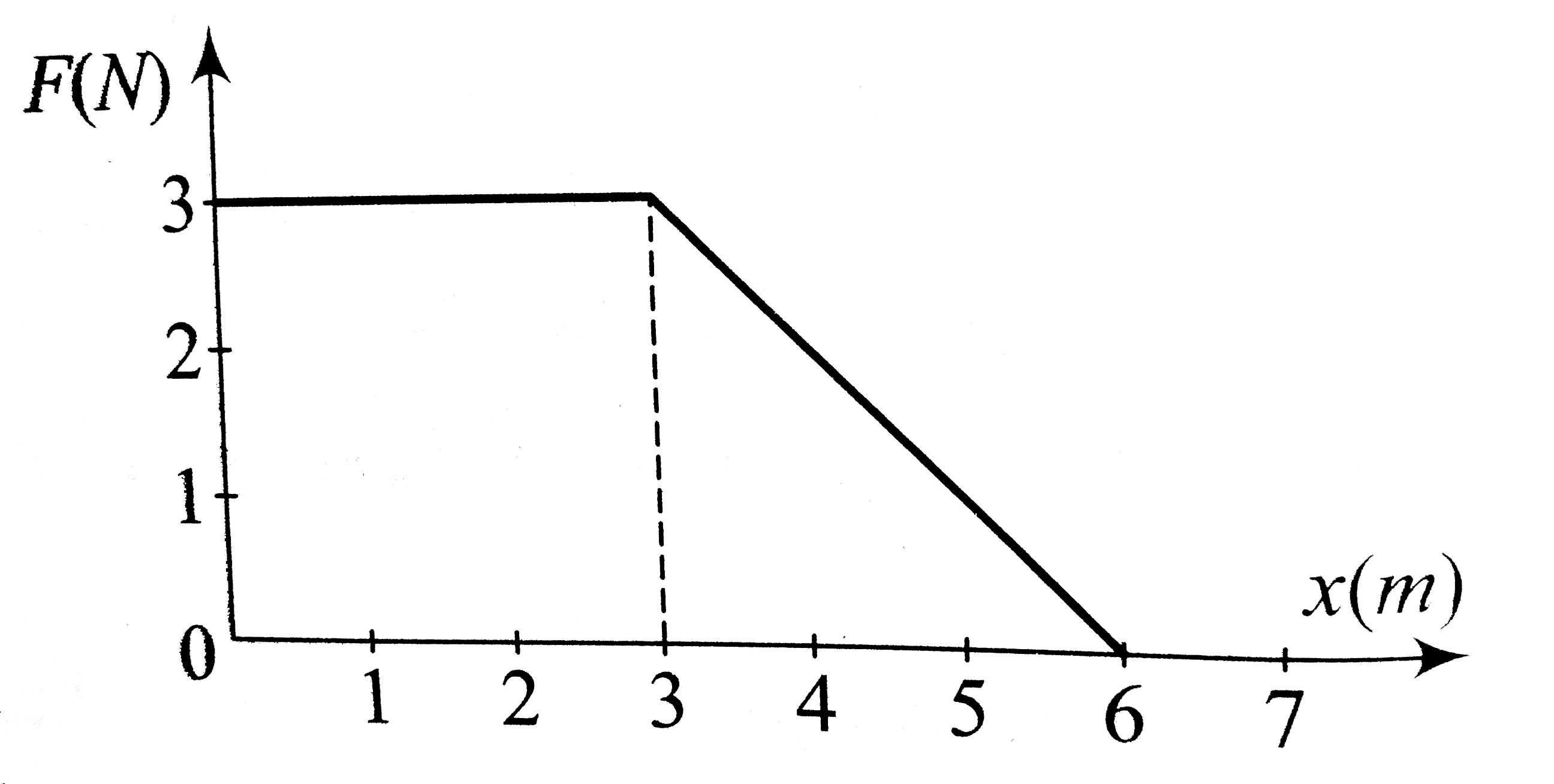
View SolutionA Force F acting on an object varies with distance x as shown in the figure . The force is in newton and x in metre. The work done by the force in moving the object from x=0 to x=6m is

(a) 4.5 J (b) 13.5 J (c) 9.0 J (d) 18.0 J
View Solution
