A block is pulled a distance x along a rough horizontal table by a horizontal string. If the tension in the string is T, the weight of the block is W, the reaction is N and frictonal force is F. Write down expressions for the work done by each of these forces.
To solve the problem of finding the work done by each force acting on a block being pulled along a rough horizontal table, we will analyze the forces involved and apply the work formula.
1. Identify the Forces Acting on the Block:
- Tension in the string (T)
- Weight of the block (W)
- Normal reaction force (N)
- Frictional force (F)
2. Work Done by the Tension (T):
- The tension pulls the block in the direction of displacement.
- The formula for work done is given by:
W=F⋅s⋅cos(θ)
- Here, F is the tension T, s is the displacement x, and the angle θ between the tension and displacement is 0∘ (since they are in the same direction).
- Therefore, the work done by tension is:
WT=T⋅x⋅cos(0)=T⋅x⋅1=T⋅x
3. Work Done by the Normal Force (N):
- The normal force acts perpendicular to the displacement.
- The angle θ between the normal force and the displacement is 90∘.
- Thus, the work done by the normal force is:
WN=N⋅x⋅cos(90)=N⋅x⋅0=0
4. Work Done by the Weight (W):
- The weight of the block also acts vertically downward, which is perpendicular to the displacement.
- The angle θ between the weight and the displacement is 90∘.
- Therefore, the work done by the weight is:
WW=W⋅x⋅cos(90)=W⋅x⋅0=0
5. Work Done by the Frictional Force (F):
- The frictional force acts opposite to the direction of displacement.
- The angle θ between the frictional force and the displacement is 180∘.
- Hence, the work done by the frictional force is:
WF=F⋅x⋅cos(180)=F⋅x⋅(−1)=−F⋅x
Summary of Work Done by Each Force:
- Work done by tension: WT=T⋅x
- Work done by normal force: WN=0
- Work done by weight: WW=0
- Work done by frictional force: WF=−F⋅x
- Tension in the string (T)
- Weight of the block (W)
- Normal reaction force (N)
- Frictional force (F)
- The tension pulls the block in the direction of displacement.
- The formula for work done is given by:
W=F⋅s⋅cos(θ)
- Here, F is the tension T, s is the displacement x, and the angle θ between the tension and displacement is 0∘ (since they are in the same direction).
- Therefore, the work done by tension is:
WT=T⋅x⋅cos(0)=T⋅x⋅1=T⋅x
- The normal force acts perpendicular to the displacement.
- The angle θ between the normal force and the displacement is 90∘.
- Thus, the work done by the normal force is:
WN=N⋅x⋅cos(90)=N⋅x⋅0=0
- The weight of the block also acts vertically downward, which is perpendicular to the displacement.
- The angle θ between the weight and the displacement is 90∘.
- Therefore, the work done by the weight is:
WW=W⋅x⋅cos(90)=W⋅x⋅0=0
- The frictional force acts opposite to the direction of displacement.
- The angle θ between the frictional force and the displacement is 180∘.
- Hence, the work done by the frictional force is:
WF=F⋅x⋅cos(180)=F⋅x⋅(−1)=−F⋅x
- Work done by tension: WT=T⋅x
- Work done by normal force: WN=0
- Work done by weight: WW=0
- Work done by frictional force: WF=−F⋅x
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.29videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.32videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Miscellaneous Example6videos
WORK, ENERGY & POWER
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question11videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY33videos
WORK, ENERGY AND POWER
Similar Questions
A particle is pulled a distance l up a rough plane inclined at an angle α to the horizontal by a string inclined at an angle β to the plane (β+α<90∘). If the tension the string is T, the normal reaction between the particle and plane is N, the frictional force is F, and the weight of the particle is w. write down expression for the work done by each of forces.
View SolutionA block placed on a rough horizontal surface is pulled by a horizontal force F Let f be the force applied by the rough surface on the block . Plot a graph of f versus F .
View SolutionA block of 10kg is pulled at a constant speed on a rough horizontal plane by a force of 20N. Calculate the coefficient of friction ?
View SolutionAs shown in figure the tension in the horizontal cord is 30N. The weight W and tension in the string OA in Newton are
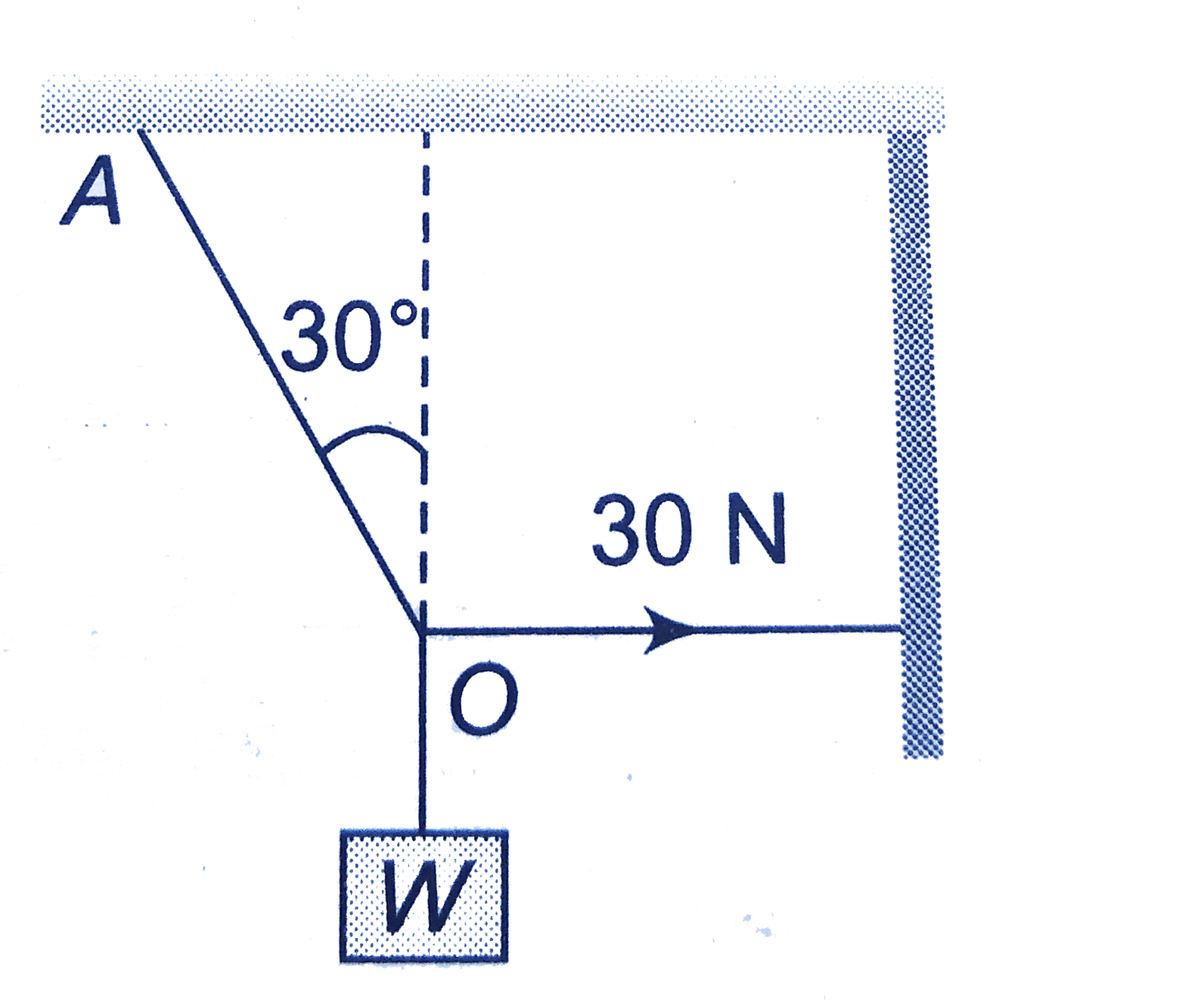 View Solution
View SolutionAs shown in figure the tension in the horizontal cord is 30N. The weight W and tension in the string OA in Newton are
 View Solution
View SolutionA block is placed on a rough horizontal plane. A time dependent horizontal force F=K t acts on the block K is a postive constant. Accelration-time graph of the block is
View SolutionA block of mass 1 kg is placed on a horizontal surface . The reaction force to the weight of the block is
View SolutionA block of mass m is placed on a rough horizontal floor and it is pulled by a ideal string by a constant force F as shown. As the block moves towards right the friction force on block
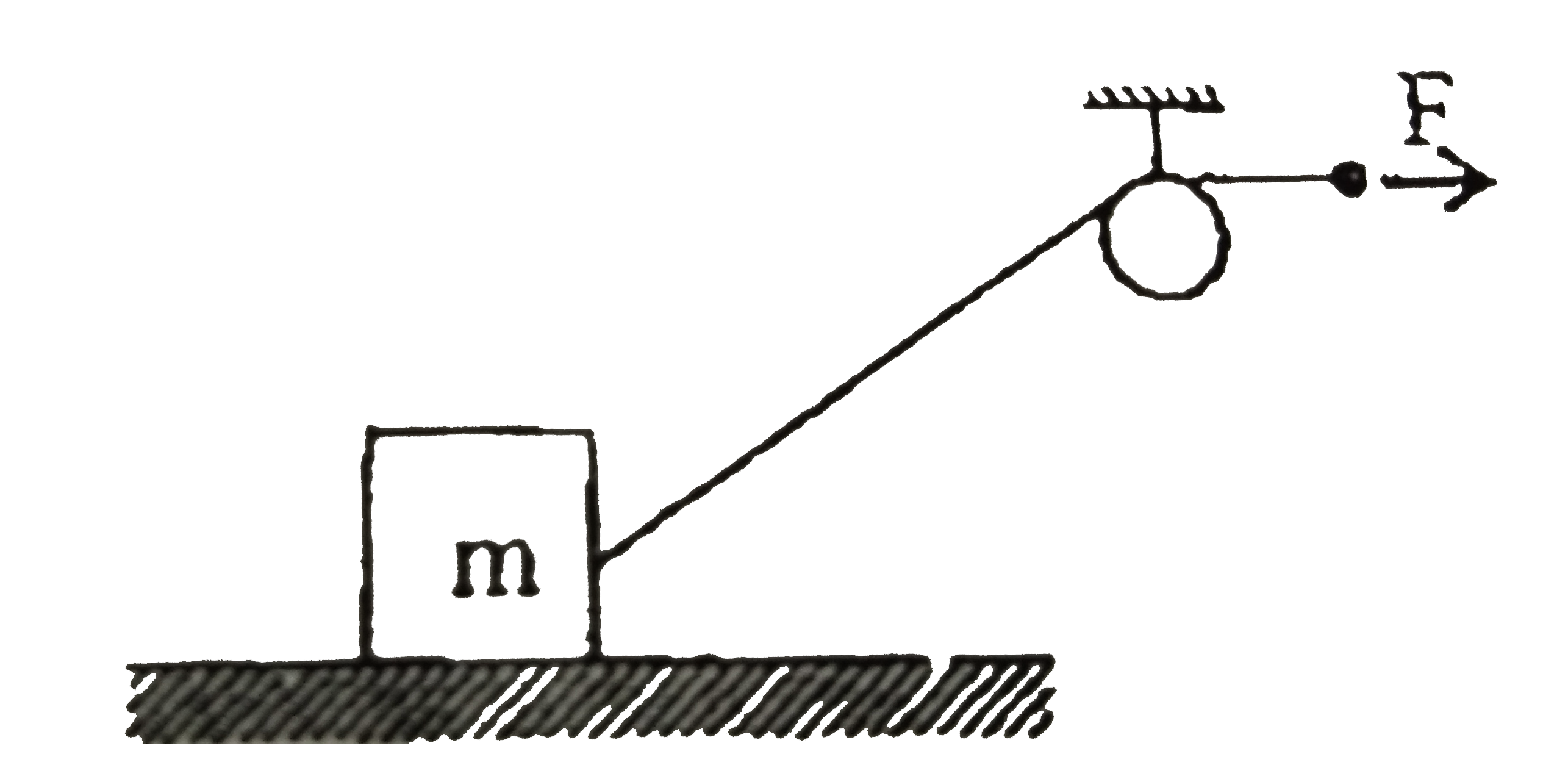 View Solution
View SolutionA block of unknown mass is at rest on a rough, horizontal surface. A horizontal force F is applied to the block. The graph in the figure shows the acceleration of the block with respect to the applied force. The mass of the block is
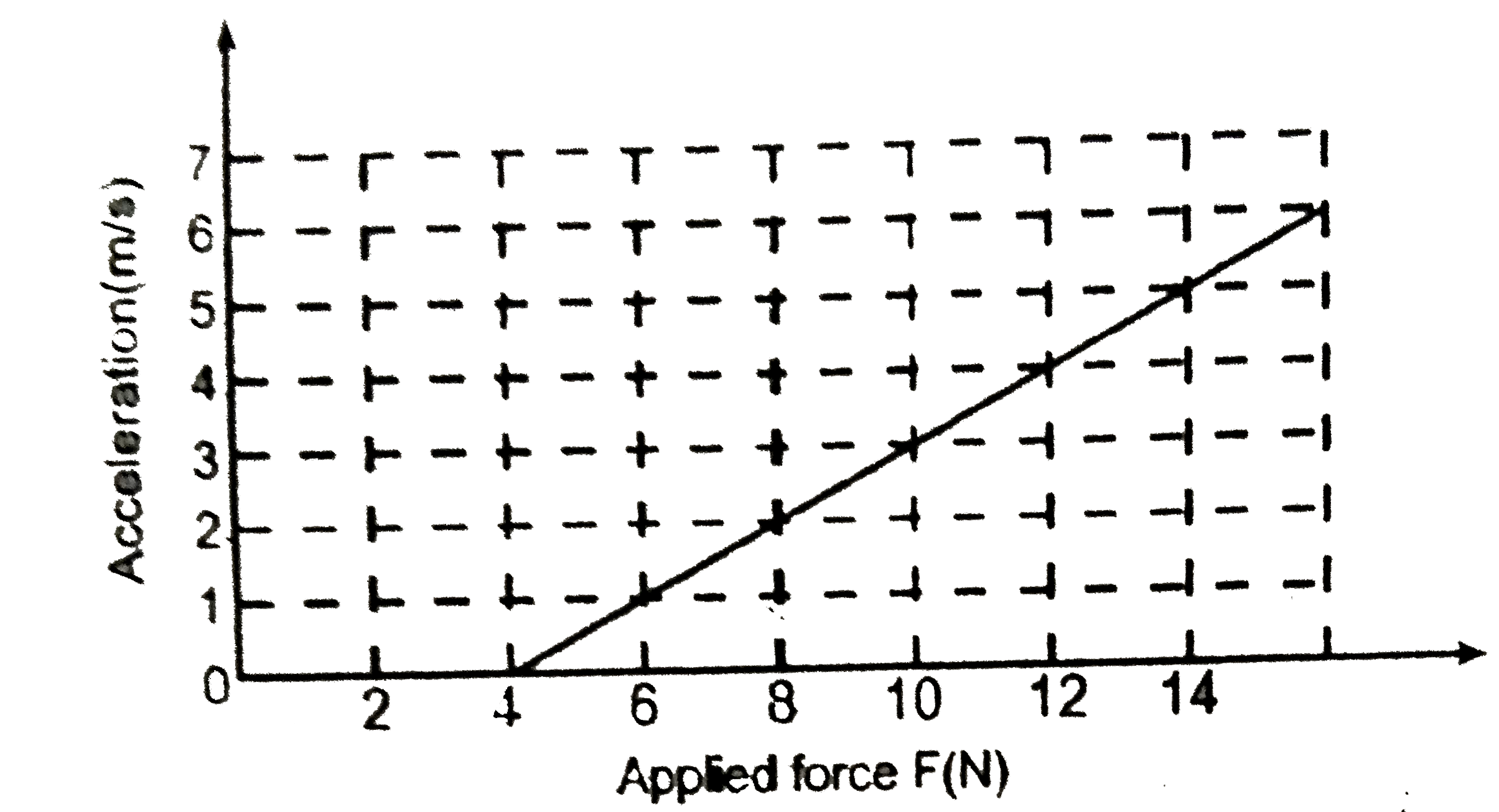 View Solution
View SolutionA block of mass 20kg is placed on a rough horizontal plane and a horizontal force of 12N is applied. If coefficient of friction is 0.1 the frictional force acting on it is,
View Solution

