A block of density 2000kg/m3 and mass 10kg is suspended by a spring stiffness 100N/m. The other end of the spring is attached to a fixed support. The block is completely submerged in a liquid of density 1000kg/m3. If the block is in equilibrium position.
the elongation of the spring is 1 cm
the magnitude of buoyant force acting on the block is 50 N
the spring potential energy is 12.5 J
magnitude of spring force on the block is greater than the weight of the block
The correct Answer is:B, C
To solve the problem step by step, we will analyze the forces acting on the block and apply the principles of equilibrium.
Step 1: Identify the forces acting on the block
When the block is submerged in the liquid and in equilibrium, three forces act on it:
1. The weight of the block (downward).
2. The buoyant force acting on the block (upward).
3. The force exerted by the spring (upward).
Step 2: Calculate the weight of the block
The weight of the block can be calculated using the formula:
W=m⋅g
Where:
- m=10kg (mass of the block)
- g=10m/s2 (acceleration due to gravity)
Calculating the weight:
W=10kg⋅10m/s2=100N
Step 3: Calculate the buoyant force
The buoyant force can be calculated using Archimedes' principle:
Fb=Vb⋅ρl⋅g
Where:
- Vb is the volume of the block.
- ρl=1000kg/m3 (density of the liquid).
To find the volume of the block, we can use its density:
Vb=mρb=10kg2000kg/m3=0.005m3
Now, substituting in the buoyant force formula:
Fb=0.005m3⋅1000kg/m3⋅10m/s2=50N
Step 4: Calculate the force exerted by the spring
The force exerted by the spring can be expressed as:
Fs=k⋅x
Where:
- k=100N/m (spring constant)
- x is the extension of the spring.
Step 5: Apply equilibrium condition
In equilibrium, the sum of upward forces equals the sum of downward forces:
Fb+Fs=W
Substituting the known values:
50N+(100N/m⋅x)=100N
Step 6: Solve for x
Rearranging the equation:
100N/m⋅x=100N−50N
100N/m⋅x=50N
x=50N100N/m=0.5m
Step 7: Calculate the potential energy stored in the spring
The potential energy stored in the spring can be calculated using:
PE=12kx2
Substituting the values:
PE=12⋅100N/m⋅(0.5m)2=12⋅100⋅0.25=12.5J
Final Results
1. The buoyant force acting on the block is 50N.
2. The potential energy stored in the spring is 12.5J.
When the block is submerged in the liquid and in equilibrium, three forces act on it:
1. The weight of the block (downward).
2. The buoyant force acting on the block (upward).
3. The force exerted by the spring (upward).
The weight of the block can be calculated using the formula:
W=m⋅g
Where:
- m=10kg (mass of the block)
- g=10m/s2 (acceleration due to gravity)
W=10kg⋅10m/s2=100N
The buoyant force can be calculated using Archimedes' principle:
Fb=Vb⋅ρl⋅g
Where:
- Vb is the volume of the block.
- ρl=1000kg/m3 (density of the liquid).
Vb=mρb=10kg2000kg/m3=0.005m3
Fb=0.005m3⋅1000kg/m3⋅10m/s2=50N
The force exerted by the spring can be expressed as:
Fs=k⋅x
Where:
- k=100N/m (spring constant)
- x is the extension of the spring.
In equilibrium, the sum of upward forces equals the sum of downward forces:
Fb+Fs=W
Substituting the known values:
50N+(100N/m⋅x)=100N
Rearranging the equation:
100N/m⋅x=100N−50N
100N/m⋅x=50N
x=50N100N/m=0.5m
The potential energy stored in the spring can be calculated using:
PE=12kx2
Substituting the values:
PE=12⋅100N/m⋅(0.5m)2=12⋅100⋅0.25=12.5J
1. The buoyant force acting on the block is 50N.
2. The potential energy stored in the spring is 12.5J.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Comprehension
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Matrix Matching
12 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced
57 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
A block of mass 1 kg is attached to one end of a spring of force constant k = 20 N/m. The other end of the spring is attached to a fixed rigid support. This spring block system is made to oscillate on a rough horizontal surface (μ=0.04). The initial displacement of the block from the equilibrium position is a = 30 cm. How many times the block passes from the mean position before coming to rest ? (g=10m/s2)
View SolutionA block of 2 kg is suspended from the ceiling through a massless spring of spring constant k=100 N/m. What is the elongation of the spring? If another 1 kg is added to the block, what would be the further elongation?
View SolutionA block of mass 4 kg and volume 5×10−4m3 is suspended by a spring balance in a lift which is accelerating. The apparent weight shown by the spring balance is 3 kg. Now the block is immersed in water in kg shown by the spring balance is
View SolutionA block of mass m, lying on a smooth horizontal surface, is attached to a spring (of negligible mass) of spring constant k. The other end of the spring is fixed, as shown in the figure. The block is initally at rest in its equilibrium position. If now the block is pulled withe a constant force F, the maximum speed of the block is :

View SolutionA rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m3) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.
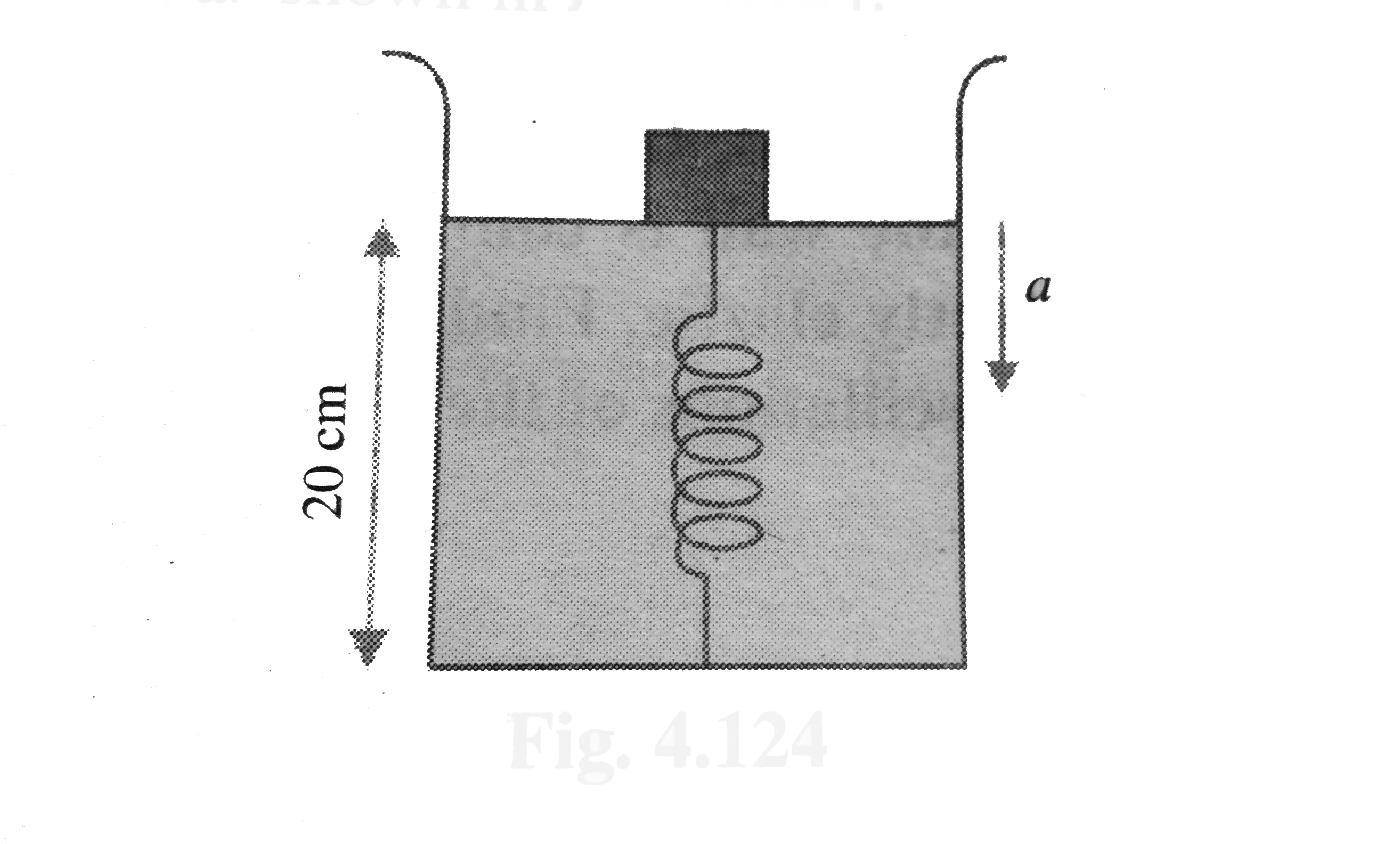
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.
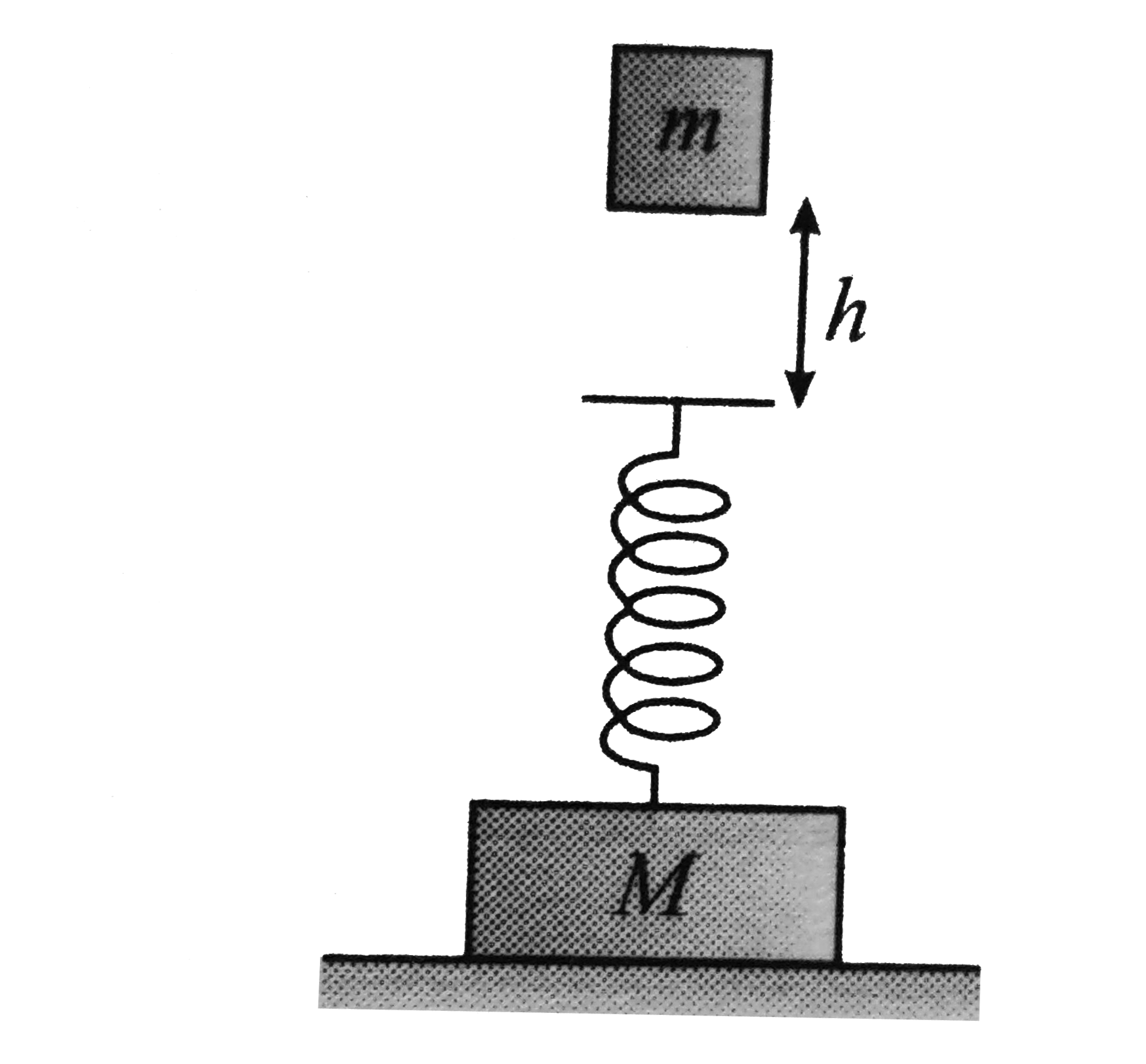
View SolutionA block of mass m is dropped onto a spring of constant k from a height h. The second end of the spring is attached to a second block of mass M as shown in figure. Find the minimum value of h so that the block M bounces off the ground. If the block of mass m sticks to the spring immediately after it comes into contact with it.
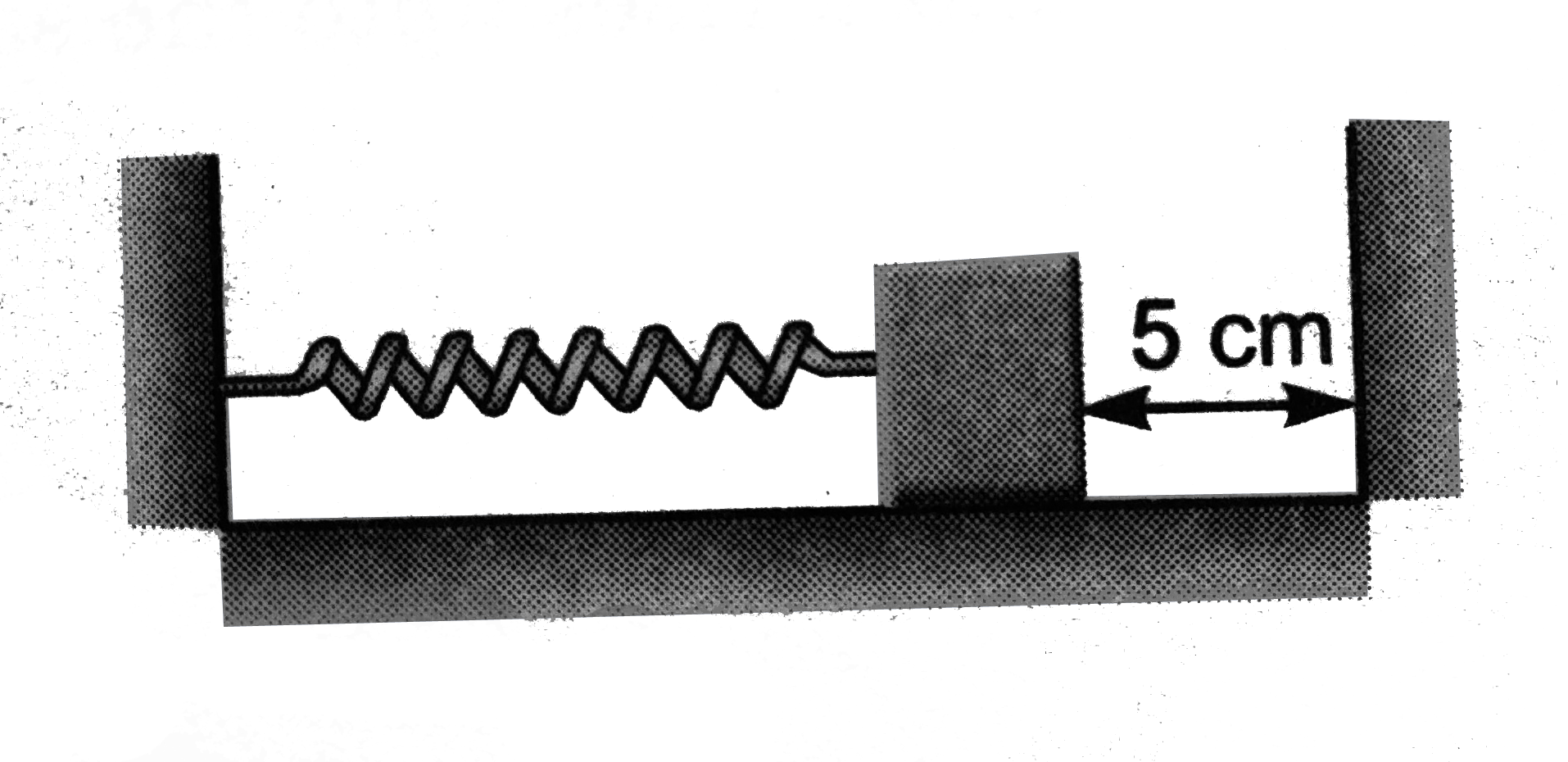
View SolutionA block of mass 100g attached to a spring of stiffness 100N/m is lying on a frictionless floor as shown. The block is moved to compress the spring by 10cm and released. If the collision with the wall is elastic then find the time period of oscillations.
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionA block of mass 2kg is attached with a spring of spring constant 4000Nm−1 and the system is kept on smooth horizontal table. The other end of the spring is attached with a wall. Initially spring is stretched by 5cm from its natural position and the block is at rest. Now suddenly an impulse of 4kg−ms−1 is given to the block towards the wall.
Find the velocity of the block when spring acquires its natural length
View SolutionA block of ice with volume 2.76m3 has a mass of 2530 kg, find its density.
View Solution
