A block of mass is attached with a spring of spring constant and the system is kept on smooth horizontal table. The other end of the spring is attached with a wall. Initially spring is stretched by from its natural position and the block is at rest. Now suddenly an impulse of is given to the block towards the wall.
Approximate distance travelled by the block when it comes to rest for a second time (not including the initial one) will be (Take )
(a)
(b)
(c)
(d)
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Calculate the initial velocity of the block after the impulse
The impulse given to the block is J=4kg m/s and the mass of the block is m=2kg. We can use the impulse-momentum theorem:
J=mΔv
Here, Δv=v−u (where u is the initial velocity, which is 0 since the block is at rest). Therefore, we have:
4=2(v−0)⟹v=42=2m/s
Step 2: Apply the work-energy theorem
The work-energy theorem states that the work done on the block is equal to the change in kinetic energy. Initially, the block has kinetic energy due to the velocity we just calculated, and it will come to rest when the spring does work on it.
The initial kinetic energy KEi is:
KEi=12mv2=12×2×(2)2=4J
The work done by the spring when the block comes to rest is given by:
W=−12kx2
where k=4000N/m is the spring constant and x is the compression/stretch of the spring from its natural length.
Step 3: Set up the equation using the work-energy theorem
The work done by the spring will equal the initial kinetic energy:
KEi=−W
4=12kx2
Substituting k:
4=12×4000×x2
4=2000x2⟹x2=42000=0.002⟹x=√0.002=0.04472m≈4.472cm
Step 4: Calculate the total distance traveled by the block
Initially, the spring was stretched by 5cm. After the impulse, the block travels 4.472cm back towards the wall. It will then compress the spring and travel back out again.
The total distance traveled when the block comes to rest for the second time is:
Total distance=Initial stretch+Distance to rest+Distance back
The distance back is the same as the distance to rest, so:
Total distance=5cm+4.472cm+4.472cm=5+8.944=13.944cm
Step 5: Approximate the distance traveled for the second time
Since we are looking for the distance traveled when it comes to rest for the second time, we need to consider the total distance traveled after the initial stretch:
Total distance=5cm+2×4.472cm≈5+8.944≈13.944cm
However, we need to check the options provided in the question. The closest option to our calculated distance is 25cm.
Final Answer
Thus, the approximate distance traveled by the block when it comes to rest for a second time is ≈25cm.
---
The impulse given to the block is J=4kg m/s and the mass of the block is m=2kg. We can use the impulse-momentum theorem:
The work-energy theorem states that the work done on the block is equal to the change in kinetic energy. Initially, the block has kinetic energy due to the velocity we just calculated, and it will come to rest when the spring does work on it.
The work done by the spring will equal the initial kinetic energy:
Initially, the spring was stretched by 5cm. After the impulse, the block travels 4.472cm back towards the wall. It will then compress the spring and travel back out again.
Since we are looking for the distance traveled when it comes to rest for the second time, we need to consider the total distance traveled after the initial stretch:
Thus, the approximate distance traveled by the block when it comes to rest for a second time is ≈25cm.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Comprehension Based Questions
3 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 More Than One Correct
8 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A block of mass 2kg is attached with a spring of spring constant 4000Nm−1 and the system is kept on smooth horizontal table. The other end of the spring is attached with a wall. Initially spring is stretched by 5cm from its natural position and the block is at rest. Now suddenly an impulse of 4kg−ms−1 is given to the block towards the wall.
Find the velocity of the block when spring acquires its natural length
View SolutionA block of mass m, attached to a spring of spring constant k, oscilltes on a smooth horizontal table. The other end of the spring is fixed to a wall. If it has speed v when the spring is at its naturla lenth, how far will it move on the table before coming to an instantaneous rest?
View SolutionA block of mass m, attacted to a string of spring constant k, oscillates on a smooth horizontal table. The other end of the spring is fixed to a wall. The block has a speed v when the spring is at its natural length. Before coming to an instantaneous rest. If the block moves a distance x from the mean position, then
View Solutiona block of mass 0.1 kg attached to a spring of spring constant400Nm pulled horizontally from x=0 tox1=10mm. Find the work done by the spring force
View SolutionA block of mass 0.1 kg attached to a spring of spring constant 400 N/m is putted rightward from x0=0 to x1=15 mm. Find the work done by spring force.
View SolutionA block attached with an ideal spring is kept on a smooth horizontal surface. Now the free end of the spring is pulled with a constant velocity u horizontally. Then the maximum energy stored in the spring and bock system during subsequent motion is :
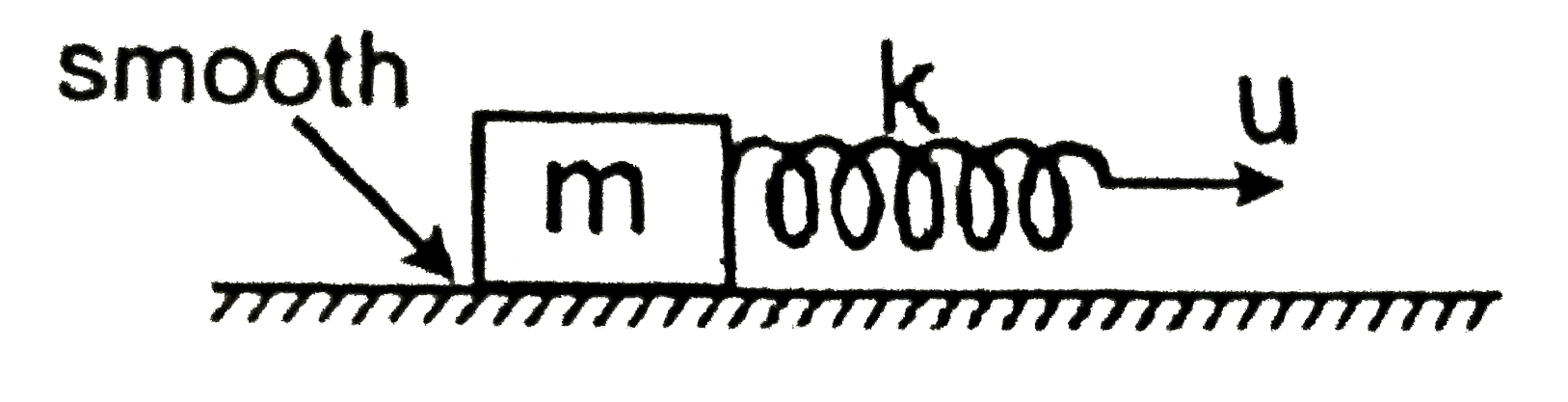
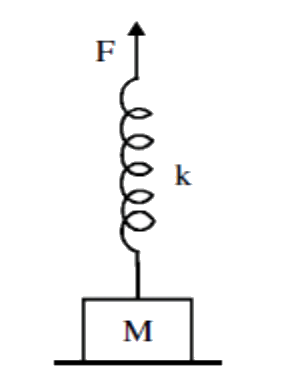
View SolutionA block of mass m is kept on a rough horizontal surface and is attached with a massless spring of force constant k. The minimum constant force applied on the other end of the spring of lift the block is
View SolutionA block of mass m is connected to another block of mass M by a massless spring of spring constant k. the blocks are kept of a smooth horizontal plane and are at rest. The spring is unstretched when a constant force F starts acting on the block of mass M of pull it. Find the maximum extension of the spring

View SolutionA spring has natural length 40cm and spring constant 500N/m. A block of mass 1kg is attached at one end of the spring and other end of the spring is attached to a ceiling. The block is relesed from the position, where the spring has length 45cm.
View SolutionA block of mass m is connect to another block of mass M by a massless spring of spring constant k. The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is upstretched when a constant force F starts acting on the block of mass M to pull it. Find the maximum extension of the spring.

View Solution
