A block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?

(M+m)(μ2−μ1)g
(M−m)(μ2−μ1)g
(M−m)(μ2+μ1)g
(M+m)(μ2+μ1)g
The correct Answer is:d
Here the force applied should be such that friction force acting on the upper block of m should not be more than the limiting friction(=μ1mg) let the system moves with acceleration a Then for whole system
F−μ2(M+m)g=(M+m)a...(i)
For block of m.
f1=maorμ1mg=maora=μ1g...(ii)
From Eqs (i) and (ii) we get
F=(M+m)g(μ1+μ2)
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct2videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Linked Comprehension52videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective21videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
Find the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Coefficient of static friction between the two blocks in Fig. 7.124 is μ and the table is frictionless . Maximum horizontal force which can be applied on the lower such that the blocks move together , is :
 Aμm1gBμm2gCμ(m1−m2)gDμ(m1+m2)g
Aμm1gBμm2gCμ(m1−m2)gDμ(m1+m2)g - Question 2 - Select One
A block of mass m is placed on the top of another block of mass M as shown in the figure. The coefficient of friction between them is μ.

The maximum acceleration with which the block M may move so that m also moves along with it isAμgBμMmgCμmMgDgμ - Question 3 - Select One
A block of mass m is placed at rest on an inclination θ to the horizontal.If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
AmgBμmgcosθCmgsinθDμmgtanθ
The coefficient of static friction between the two blocks shown in figure is μ and the table is smooth. What maximum horizontal forced F can be applied to he block of mass M so that the block move together?
 View Solution
View SolutionA block of mass m is pulled by a constant power P placed on a rough horizontal plane. The coeficient of friction between the block and surface is μ. Find the maximum velocity of the block.
View SolutionA block of mass m is kept over another block of mass 2m. Coefficient of friction between two blocks is μ and that of between lower blood from the horizontal surface is zero. A horizontal force F=43μmg is applied on the block of mass 2m. Acceleration of lower block is
View SolutionTwo blocks of masses 'm' and and 'M' are arranged as shown in the The coefficient of friction between the two blocks is 'μ', where as between the lower block and the horizontal surface is zero. Find the force 'F' to be applied on the upper block for the system to be under equilibrium ? .
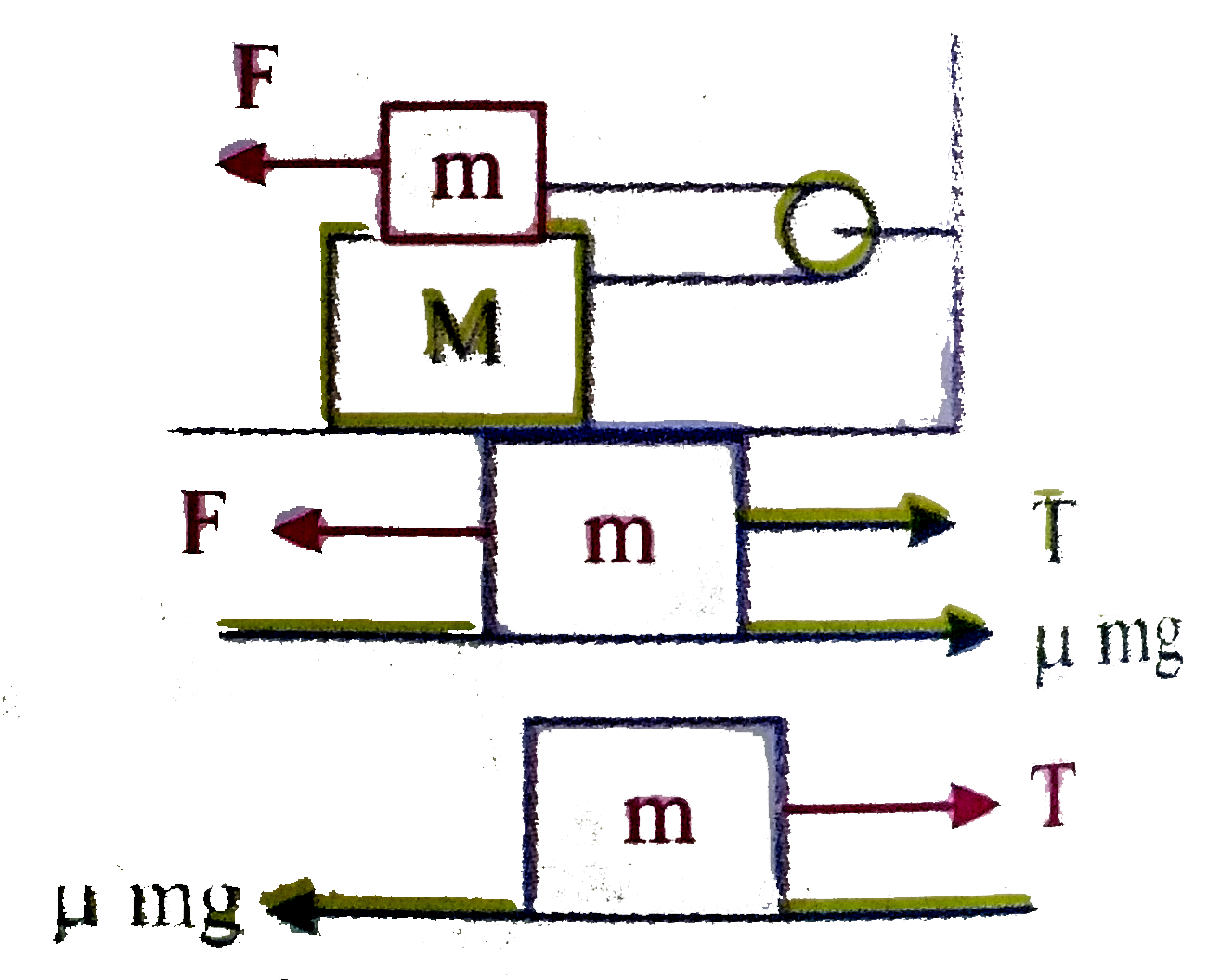 View Solution
View SolutionA block of mass m is pulled by a force of constant power P placed on a rough horizontal plane. The friction coefficient between the block and the surface is μ. Then
View Solution

