A block of mass m is thrown upwards with some initial velocity as shown in figure. On the block
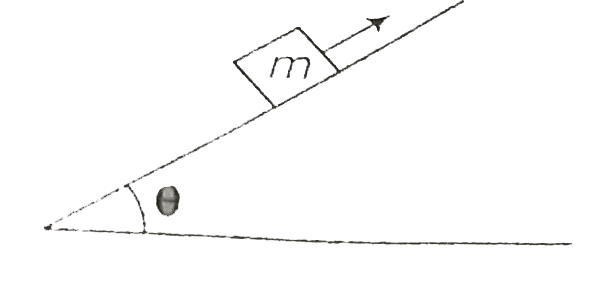
Column IColumn II(A) Net force in horizontal direction(p) Zero(B) Net force in vertical direction(q)m(gsinθ+μgcosθ)(C) Net force along the plane(r)m(gsinθcosθ+μgcos2θ)(D) Net force perpendicular to plane(s)m(gsin2θ+μgsinθcosθ)
The correct Answer is:A→r,B→s,C→q,D →p
Force of friction and mgsinθ both are downwards.
∴ Acceleration of the block is a=(gsinθ+μgcosθ) down the plane
Now, net force in any direction is equal to, F=m(component of acceleration in that direction)
Topper's Solved these Questions
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Medical entrances gallery
Explore 39 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Chapter exercises (A) Taking it together
Explore 71 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Level 2 Subjective
Explore 18 Videos
Similar Questions
Block of mass 'm' is sliding up on the smooth incline plane, with some constant velocity. Now, on the block match the magnitude value of the forces on 'm' in the given directions take the components of all the forces in the given direction)
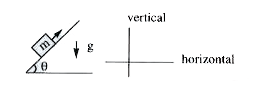
Column - IColumn -IIA) Net force component is horizontal direction P) zeroB) Net - force compnent in vertical directionQ) mgsinθcosθC) Net force component along the incline plane R)mgsinθD) Net force component perpendicular to the incline plane S) mg(1−cos2θ)
View Solutionasin2θ+bcos2θ=c⇒tan2θ=
View SolutionGiven : 4sinθ=3cosθ, find the value of :
(i) sinθ
(ii) cosθ
(iii) cot2θ−cosec2θ
(iv) 4cos2θ−3sin2θ+2
View SolutionIf 16sinθ,cosθ and tanθ are in G.P. then the general solution for θ is
View SolutionLet l=sinθ,m=cosθandn=tanθ.
Q. If θ=7 radian, then :
View SolutionIf sinθ+cosθ=p and secθ+cosecθ=q; show that q(p2−1)=2p
View SolutionThe value of (sinθ+icosθ)n is (A) sinnθ+icosnθ (B) cosnθ−isinnθ (C) cos(nπ2−nθ)+issin(nπ2−nθ) (D) none of these
View SolutionFind the value of θ in each of the following: (i)2sin2θ=√3 (ii)2cos3θ=1
View Solutionc(1+cos2θ)= (A) c2sin2θ (B) c2cos2θ (C) c2tan2θ (D) none of these
View SolutionFind the A.M. between:
(cosθ+sinθ)2 and (cosθ−sinθ)2
View Solution