A bob of mass m is suspended by a light string of length L. It is imparted a horizontal velocity v0 at the lowest point A such that it completes a semi-circular trajectory in the vertical plane with the string becoming slack on reaching the topmost point C, figure, Obtain an expression for (i) v0 (ii) the speeds at points B and C, (ii) the ration of kinetic energies (KB/KC) at B and C.
Comment on the nature of the trajectory of the bob after it reahes the poing C.
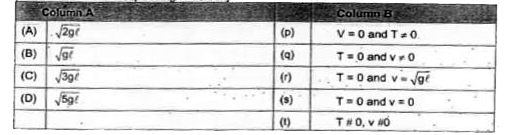
A-p,B-q,C-s,D-r
A-q,B-r,C-q,D-s
A-r,B-s,C-q,D-p
A-s,B-p,C-r,D-q
The correct Answer is:C
There are two external forces on the bob: gravity (mg) and tension (T) in the string. The tension does no work as displacement is always perpendicular to the string.Total mechanical energy (E) of the system is conserved.
If we take potential energy of the system to be zero at the lowest point A, then
At A, E=12mv20...(i)
From Newton's second law
TA−mg=mv20L ...(ii)
where TA is the tension in the string at A.
At the highest point C, to complete the circle tension in string will be minimum (zero).
At C, E =12mv2C+mg(2L)...(iii)
From Newton's second law
mg=mv2CL ...(iv)
From (iv), vC=√gL,C-q
From (iii) , E=12m(gL)+2mgL=52mgL...(v)
Using (i),12mv20=52mgL,
v0=√5gL,∴A−r...(vi)
At B, E=12mv2B+mg(L)
or 12mv2B=E−mg(L)=52mgL−mgL (Using (v))
12mv2B=32mgL
∴vB=√3gL,∴B−s
The ratio of kinetic energies at B and C is ∴KBKC=12mv2B12mv2C=3gLgL=31,∴D−p
Topper's Solved these Questions
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - NCERT
18 Videos
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - ASSERTION & REASON
15 Videos
- WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - Assertion And Reason
15 Videos
Similar Questions
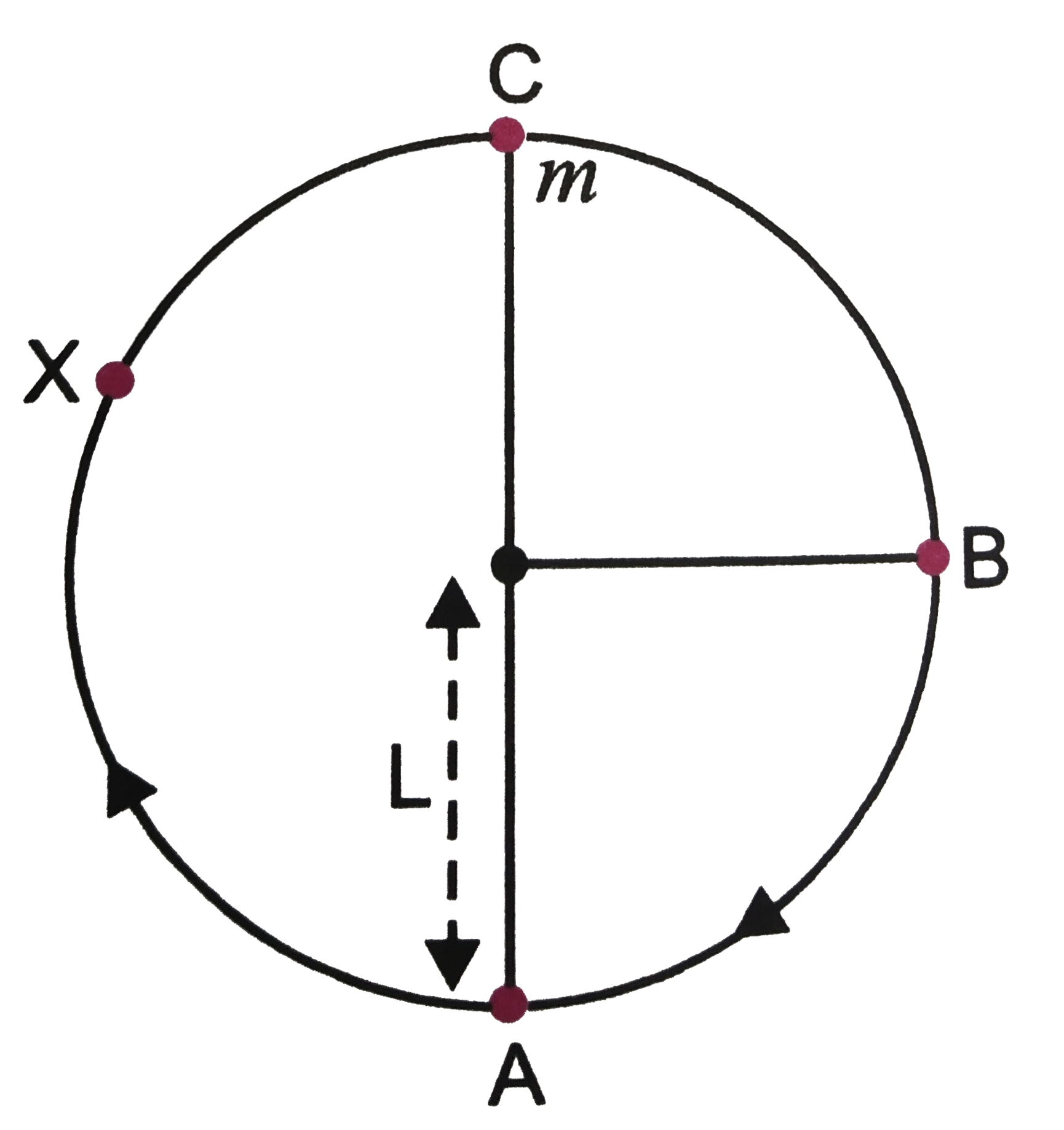
A bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure . What will be the trajectory of the particle if the string is cut at
(a) Point B ? (b) Point C? (c) Point X?
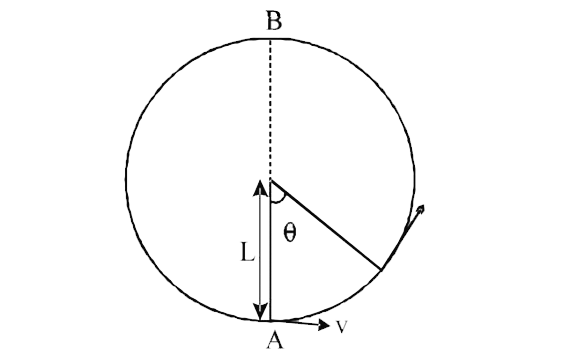
View SolutionA bob of mass M is suspended by a massless string of length L. The horizontal velocity v at position A is just sufficient to make it reach the point B. The angle θ at which the speed of the bob is half of that at A, satisfies
View SolutionThe maximum velocity at the lowest point, so that the string just slack at the highest point in a vertical circle of radius l.
View SolutionA heavy particle hangs from a point O, by a string of length a. It is projected horizontally with a velocity u=√(2+√3)ag . The angle with the downward vertical, string makes where string becomes slack is :
View SolutionA cricket ball of mass m is hitted at the angle 45∘ to the horizontal with velcoity v its kinetic energy at the topmost point is
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
View SolutionA particle in projected with velocity √3gL at point A (lowest point of the circle) in the vertical plane. Find the maximum height about horizontal level of point A if the string slacks at the point B as shown in figure.

View SolutionA particle of mass m is attached to a string of length L and given velocity √10gL in the horizontal direction at the lowest point. Find tension in the string when the particle is at (i) lowest position (ii) highest position,
View SolutionA bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
View Solution
