A bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
To solve the problem of finding the tension in the string when the bob deflects through an angle of 120∘ from the vertical, we will follow these steps:
Step 1: Understand the system
We have a bob of mass m attached to a string of length l. The bob is given an initial speed of √6gl and swings to an angle of 120∘ from the vertical.
Step 2: Determine the conservation of mechanical energy
Since there are no non-conservative forces acting on the system (like friction), we can apply the principle of conservation of mechanical energy. The initial kinetic energy (KE) plus the initial potential energy (PE) will equal the final kinetic energy plus the final potential energy.
- Initial kinetic energy (at the lowest point):
KEi=12mv2i=12m(√6gl)2=3mgl
- Initial potential energy (at the lowest point):
PEi=0(taking the lowest point as the reference level)
Step 3: Calculate the height at 120∘
At 120∘, we need to find the height h the bob has risen. The vertical height can be calculated using trigonometry:
- The vertical position of the bob at 120∘ can be calculated as:
h=l−lcos(120∘)=l−l(−12)=l+l2=3l2
Thus, the height h from the lowest point is:
h=l(1+sin(30∘))=l(1+12)=3l2
Step 4: Calculate the final potential energy
The potential energy at this height is:
PEf=mgh=mg⋅3l2=3mgl2
Step 5: Set up the energy conservation equation
Using conservation of energy:
KEi+PEi=KEf+PEf
Substituting the values:
3mgl+0=12mv2f+3mgl2
This simplifies to:
3mgl=12mv2f+3mgl2
Rearranging gives:
12mv2f=3mgl−3mgl2=3mgl2
Thus:
v2f=3gl⇒vf=√3gl
Step 6: Analyze forces at 120∘
At this position, we have two forces acting on the bob:
1. The tension T in the string acting along the string.
2. The weight mg acting downward.
The component of the weight acting along the direction of the string is:
mgcos(60∘)=mg⋅12=mg2
Step 7: Apply the centripetal force equation
The net force providing the centripetal acceleration is given by:
T−mgcos(60∘)=mv2fl
Substituting the values:
T−mg2=m⋅3gll
This simplifies to:
T−mg2=3mg
Thus:
T=3mg+mg2=6mg2+mg2=7mg2
Final Answer
The tension in the string when the bob deflects through an angle of 120∘ from the vertical is:
T=7mg2
We have a bob of mass m attached to a string of length l. The bob is given an initial speed of √6gl and swings to an angle of 120∘ from the vertical.
Since there are no non-conservative forces acting on the system (like friction), we can apply the principle of conservation of mechanical energy. The initial kinetic energy (KE) plus the initial potential energy (PE) will equal the final kinetic energy plus the final potential energy.
KEi=12mv2i=12m(√6gl)2=3mgl
- Initial potential energy (at the lowest point):
PEi=0(taking the lowest point as the reference level)
At 120∘, we need to find the height h the bob has risen. The vertical height can be calculated using trigonometry:
- The vertical position of the bob at 120∘ can be calculated as:
h=l−lcos(120∘)=l−l(−12)=l+l2=3l2
Thus, the height h from the lowest point is:
h=l(1+sin(30∘))=l(1+12)=3l2
The potential energy at this height is:
PEf=mgh=mg⋅3l2=3mgl2
Using conservation of energy:
KEi+PEi=KEf+PEf
Substituting the values:
3mgl+0=12mv2f+3mgl2
This simplifies to:
3mgl=12mv2f+3mgl2
Rearranging gives:
12mv2f=3mgl−3mgl2=3mgl2
Thus:
v2f=3gl⇒vf=√3gl
At this position, we have two forces acting on the bob:
1. The tension T in the string acting along the string.
2. The weight mg acting downward.
mgcos(60∘)=mg⋅12=mg2
The net force providing the centripetal acceleration is given by:
T−mgcos(60∘)=mv2fl
Substituting the values:
T−mg2=m⋅3gll
This simplifies to:
T−mg2=3mg
Thus:
T=3mg+mg2=6mg2+mg2=7mg2
The tension in the string when the bob deflects through an angle of 120∘ from the vertical is:
T=7mg2
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Exercise 8.5
Explore 15 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Subjective
Explore 23 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Exercise 8.3
Explore 26 Videos
- VECTORSBook:CENGAGE PHYSICS ENGLISHChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
An iron ball of mass m, suspended by a light inextensible string of length l from a fixed point O, is shifted by θ0 as shown so as to strike the vertical wail perpendicularly.The maximum angle made by the string with vertical after the first collision, if e is the coefficient of restitution, is
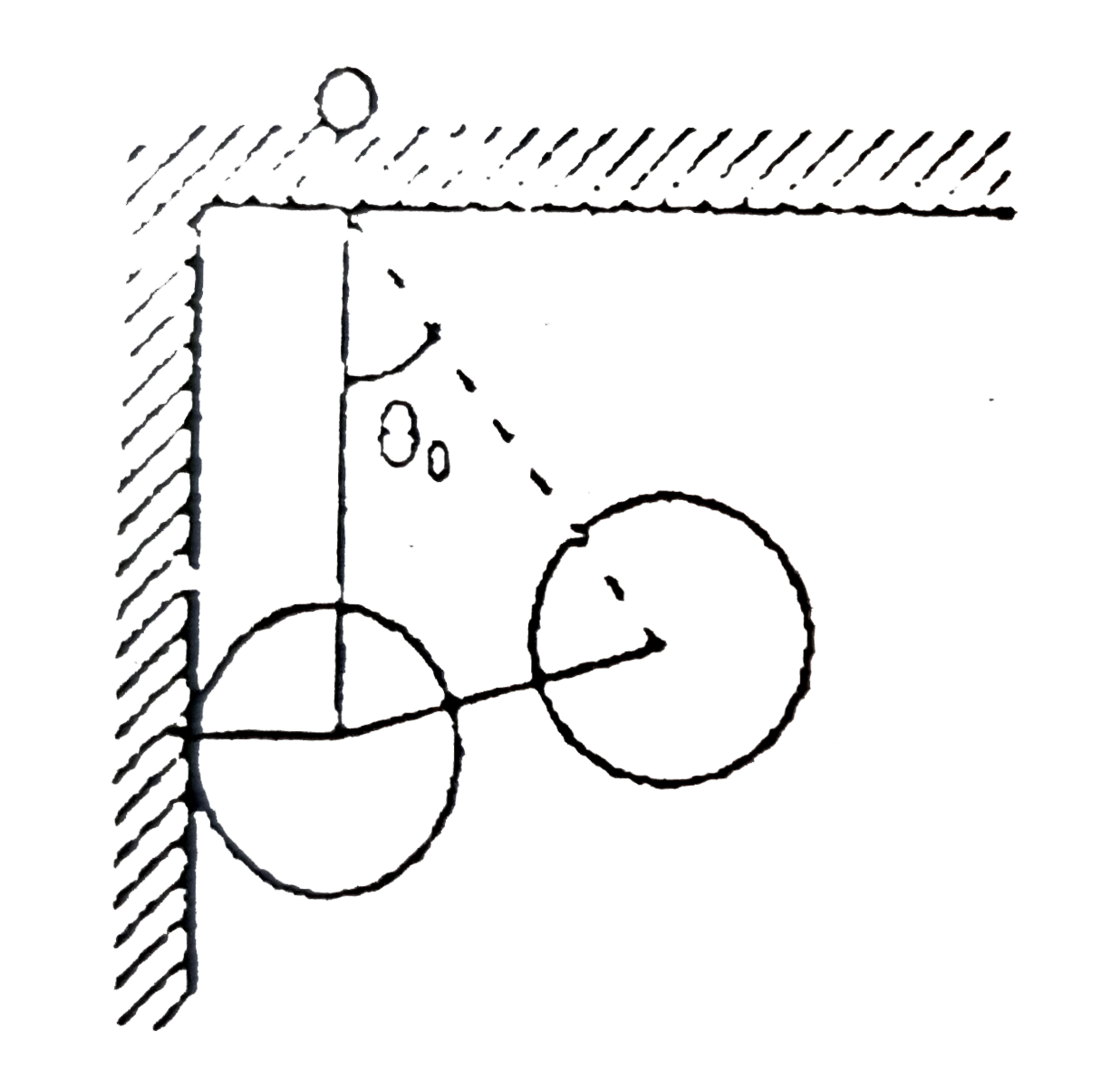
View SolutionA particle is suspended by a light vertical inelastic string of length 1 from a fixed support. At its equilbrium position it is projected horizontally with a speed √6gl. Find the ratio of the tension in the string in its horizontal position to that in the string when the particle is vertically above the point of support.
View SolutionA particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity √7gl/2. The string will slack after swinging through an angle equal to
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizonally with speed √gl . Find the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string is equal to the weight of the particle.
View SolutionA heavy particle hanging from a fixed point by a light inextensible string of length l is projected horizontally with speed (gl) . Then the speed of the particle and the inclination of the string to the vertical at the instant of the motion when the tension in the string equal the weight of the particle-
View SolutionA pendulum consisting of a small sphere of mass m, suspended by a inextensible and massless string of length 1 , is made to swing in a vertical plane. If the breaking strength of the string is 2 mg, then the maximum angular amplitude of the displacement from the vertical can be
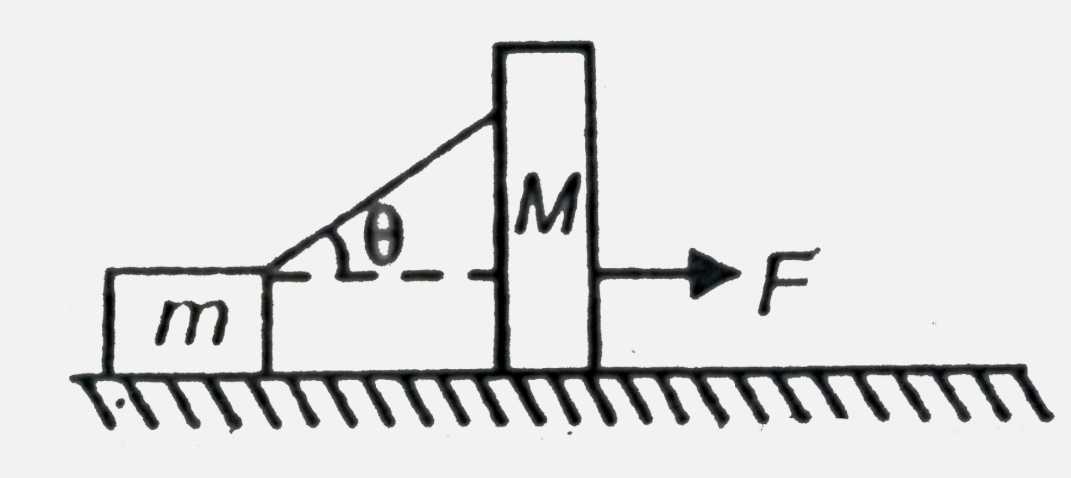
View SolutionTwo blocks of masses m and M are connected by an inextensible light string . When a constant horizontal force acts on the block of mass M. The tension in the string is
View SolutionA ball of mass 0.6kg attached to a light inextensible string rotates in a vertical circle of radius 0.75m such that it has speed of 5ms−1 when the string is horizontal. Tension in the string when it is horizontal on other side is (g=10ms−2).
View SolutionA bob of mass 1 kg is suspended from an inextensible string of length 1 m. When the string makes an angle 60∘ with vertical, speed of the bob is 4 m/s
Net acceleration of the bob at this instant is
View SolutionA particle of mass m is suspended by a string of length l from a fixed rigid support. A sufficient horizontal velocity =√3gl is imparted to it suddenly. Calculate the angle made by the string with the vertical when the accekleration of the particle is inclined to the string by 45∘ .
View Solution