A body is vibrating with SHM of amplitude 15 cm and frequency 4 Hz. Compute the maximum values of the acceleration and velocity.
To solve the problem of finding the maximum values of acceleration and velocity for a body vibrating with Simple Harmonic Motion (SHM), we can follow these steps:
Step 1: Identify the given values
- Amplitude (A) = 15 cm = 0.15 m (convert to meters)
- Frequency (f) = 4 Hz
Step 2: Calculate the angular frequency (ω)
The angular frequency (ω) can be calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2×π×4Hz
ω≈2×3.14×4≈25.12rad/s
Step 3: Calculate the maximum velocity (V_max)
The maximum velocity in SHM is given by the formula:
Vmax=ωA
Substituting the values of ω and A:
Vmax=25.12rad/s×0.15m
Vmax≈3.77m/s
Step 4: Calculate the maximum acceleration (A_max)
The maximum acceleration in SHM is given by the formula:
Amax=ω2A
Substituting the values of ω and A:
Amax=(25.12rad/s)2×0.15m
Calculating ω²:
ω2≈25.122≈631.06(rad/s)2
Now substituting this value:
Amax≈631.06×0.15≈94.66m/s2
Final Answers
- Maximum Velocity (V_max) ≈ 3.77 m/s
- Maximum Acceleration (A_max) ≈ 94.66 m/s²
- Amplitude (A) = 15 cm = 0.15 m (convert to meters)
- Frequency (f) = 4 Hz
The angular frequency (ω) can be calculated using the formula:
ω=2πf
Substituting the given frequency:
ω=2×π×4Hz
ω≈2×3.14×4≈25.12rad/s
The maximum velocity in SHM is given by the formula:
Vmax=ωA
Substituting the values of ω and A:
Vmax=25.12rad/s×0.15m
Vmax≈3.77m/s
The maximum acceleration in SHM is given by the formula:
Amax=ω2A
Substituting the values of ω and A:
Amax=(25.12rad/s)2×0.15m
Calculating ω²:
ω2≈25.122≈631.06(rad/s)2
Now substituting this value:
Amax≈631.06×0.15≈94.66m/s2
- Maximum Velocity (V_max) ≈ 3.77 m/s
- Maximum Acceleration (A_max) ≈ 94.66 m/s²
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Multiple Choice Question-I
21 Videos
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Multiple Choice Question-II
14 Videos
- OSCILLATIONS AND WAVESBOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Fill In The Blanks
20 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
Similar Questions
A particle executing S.H.M. having amplitude 0.01 m and frequency 60 Hz. Determine maximum acceleration of particle.
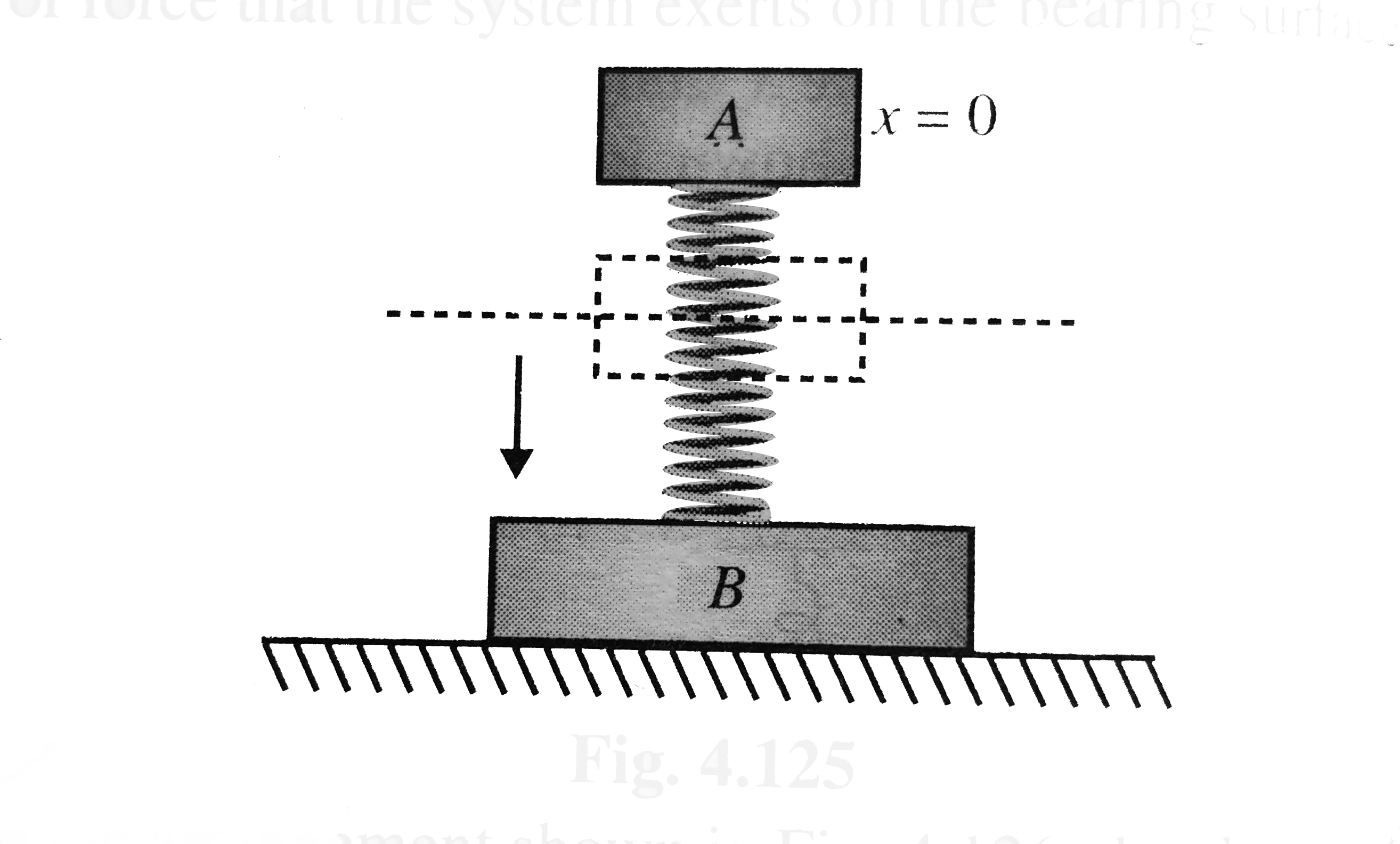
View SolutionA body A mass m1=1kg and body B of mass m2=4.1kg. The body A perform free vertical harmonic oscillations with the amplitude 1.6cm and frequency 25Hz. Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
View Solution
Knowledge Check
A particle executes S.H.M. with an amplitude 0.5 m and frequency 100 Hz. The maximum speed of the particle will be
Aπm/sB0.5 m/sC5π×10−5m/sD100π m/sA body is vibrating in simple harmonic motion with an amplitude of 0.06 m and frequency of 15 Hz . The velocity and acceleration of body is
A5.65m/sand5.32×102m/s2B6.82m/sand7.62×102m/s2C8.91m/sand8.21×102m/s2D9.82m/sand9.03×102m/s2A particle is executing simple harmonic motion with an amplitude of 0.02 metre and frequency 50 Hz . The maximum acceleration of the particle is
A100m/s2B100π2m/s2C100m/s2D200π2m/s2
The amplitude of a particle executing S.H.M. with frequency of 60 Hz is 0.01 m. The maximum value of the acceleration of the particle is
View SolutionA particle executes SHM with a amplitude 0.5cm and frequency 100s−1. The maximum speed of the particle is (in m/s)
View SolutionA particle is executing SHM with an amplitude of 2 m. The difference in the magnitudes of its maximum acceleration and maximum velocity is 4. The time period of its oscillation is
View SolutionA particle executing simple harmonic motion with an amplitude A and angularr frequency ω. The ratio of maximum acceleration to the maximum velocity of the particle is
View SolutionA particle of mass 1 kg is moving in SHM with an amplitude 0.02 m and a frequency of 60 Hz. The maximum force (in N) acting on the particle is
View Solution