A body of mass 8kg is in limiting equilibrium over an inclined plane of inclination . If the inclination is made , the minimum force required to prevent the body from sliding down is
80 N
The correct Answer is:B
To solve the problem step by step, we will analyze the forces acting on the body on the inclined plane and use the concept of limiting equilibrium.
Step 1: Identify the forces acting on the body
When a body is on an inclined plane, the forces acting on it are:
- The weight of the body (mg), acting vertically downwards.
- The normal force (N), acting perpendicular to the inclined plane.
- The frictional force (f), acting parallel to the inclined plane, opposing the motion.
Step 2: Resolve the weight into components
The weight of the body can be resolved into two components:
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
For our case, we have:
- Mass (m) = 8 kg
- Gravitational acceleration (g) = 10 m/s²
- Inclination angle (θ) = 30°
Calculating the weight:
mg=8kg×10m/s2=80N
Step 3: Write the equilibrium condition for limiting friction
In limiting equilibrium, the frictional force (f) is given by:
f=μN
Where μ is the coefficient of friction. The normal force (N) is equal to the perpendicular component of the weight:
N=mgcosθ
Thus, we have:
f=μ(mgcosθ)
Step 4: Set up the equation for limiting equilibrium
At 30° inclination, the body is in limiting equilibrium, so:
mgsin30°=f
Substituting for f:
mgsin30°=μ(mgcos30°)
Step 5: Solve for the coefficient of friction (μ)
Substituting the values:
80sin30°=μ(80cos30°)
80×12=μ(80×√32)
Cancelling 80 from both sides:
12=μ√32
Thus:
μ=1√3
Step 6: Analyze the situation at 60° inclination
Now, when the inclination is changed to 60°, we need to find the minimum force (F) required to prevent the body from sliding down. The equation becomes:
F+f=mgsin60°
Substituting for f:
F+μ(mgcos60°)=mgsin60°
Step 7: Substitute the values
Substituting the known values:
F+1√3(80×12)=80×√32
F+40√3=40√3
Step 8: Solve for F
Rearranging gives:
F=40√3−40√3
To simplify, find a common denominator:
F=40√3⋅√3√3−40√3
F=120−40√3=80√3
Final Answer
The minimum force required to prevent the body from sliding down is:
F=80√3N
When a body is on an inclined plane, the forces acting on it are:
- The weight of the body (mg), acting vertically downwards.
- The normal force (N), acting perpendicular to the inclined plane.
- The frictional force (f), acting parallel to the inclined plane, opposing the motion.
The weight of the body can be resolved into two components:
- Perpendicular to the incline: mgcosθ
- Parallel to the incline: mgsinθ
- Mass (m) = 8 kg
- Gravitational acceleration (g) = 10 m/s²
- Inclination angle (θ) = 30°
mg=8kg×10m/s2=80N
In limiting equilibrium, the frictional force (f) is given by:
f=μN
Where μ is the coefficient of friction. The normal force (N) is equal to the perpendicular component of the weight:
N=mgcosθ
f=μ(mgcosθ)
At 30° inclination, the body is in limiting equilibrium, so:
mgsin30°=f
Substituting for f:
mgsin30°=μ(mgcos30°)
Substituting the values:
80sin30°=μ(80cos30°)
80×12=μ(80×√32)
Cancelling 80 from both sides:
12=μ√32
Thus:
μ=1√3
Now, when the inclination is changed to 60°, we need to find the minimum force (F) required to prevent the body from sliding down. The equation becomes:
F+f=mgsin60°
Substituting for f:
F+μ(mgcos60°)=mgsin60°
Substituting the known values:
F+1√3(80×12)=80×√32
F+40√3=40√3
Rearranging gives:
F=40√3−40√3
To simplify, find a common denominator:
F=40√3⋅√3√3−40√3
F=120−40√3=80√3
The minimum force required to prevent the body from sliding down is:
F=80√3N
Topper's Solved these Questions
- APPENDICES (REVISION EXERCISE)Book:AAKASH SERIESChapter:APPENDICES (REVISION EXERCISE)Exercise:MOTION IN A PLANE
Explore 87 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MAGNETISM AND MATTER )
Explore 52 Videos
- ATOMSBook:AAKASH SERIESChapter:ATOMSExercise:PRACTICE EXERCISE
Explore 21 Videos
Similar Questions
A solid cylinder of mass 3kg is placed on a rough inclined plane of inclination 30∘. If g=10ms2, then the minimum frictional force required for it to roll without slipping down the plane is

View SolutionA body of mass m is kept on an inclined plane . The force required to just move a body up the inclined plane is double the force required to just prevent the body from sliding down the plane. If the coefficient of friction is μ, what is the inclination of the plane ?
View Solution
Knowledge Check
A body of mass 100 g is sliding from an inclined plane of inclination 30∘. What is the frictional force experienced if μ=1.7 ?
A1.7×√2×(1/√3)NB1.7×√3×(1/2)NC1.7×√3 ND1.7×√2×(1/3) N
A wooden box of mass 8kg slides down an inclined plane of inclination 30∘ to the horizontal with a constant acceleration of 0.4ms−2 What is the force of friction between the box and inclined plane ? (g=10m/s2) .
View SolutionA wooden box of mass 8kg slides down an inclined plane of inclination 30∘ to the horizontal with a constant acceleration of 0.4ms−2 What is the force of friction between the box and inclined plane ? (g=10m/s2) .
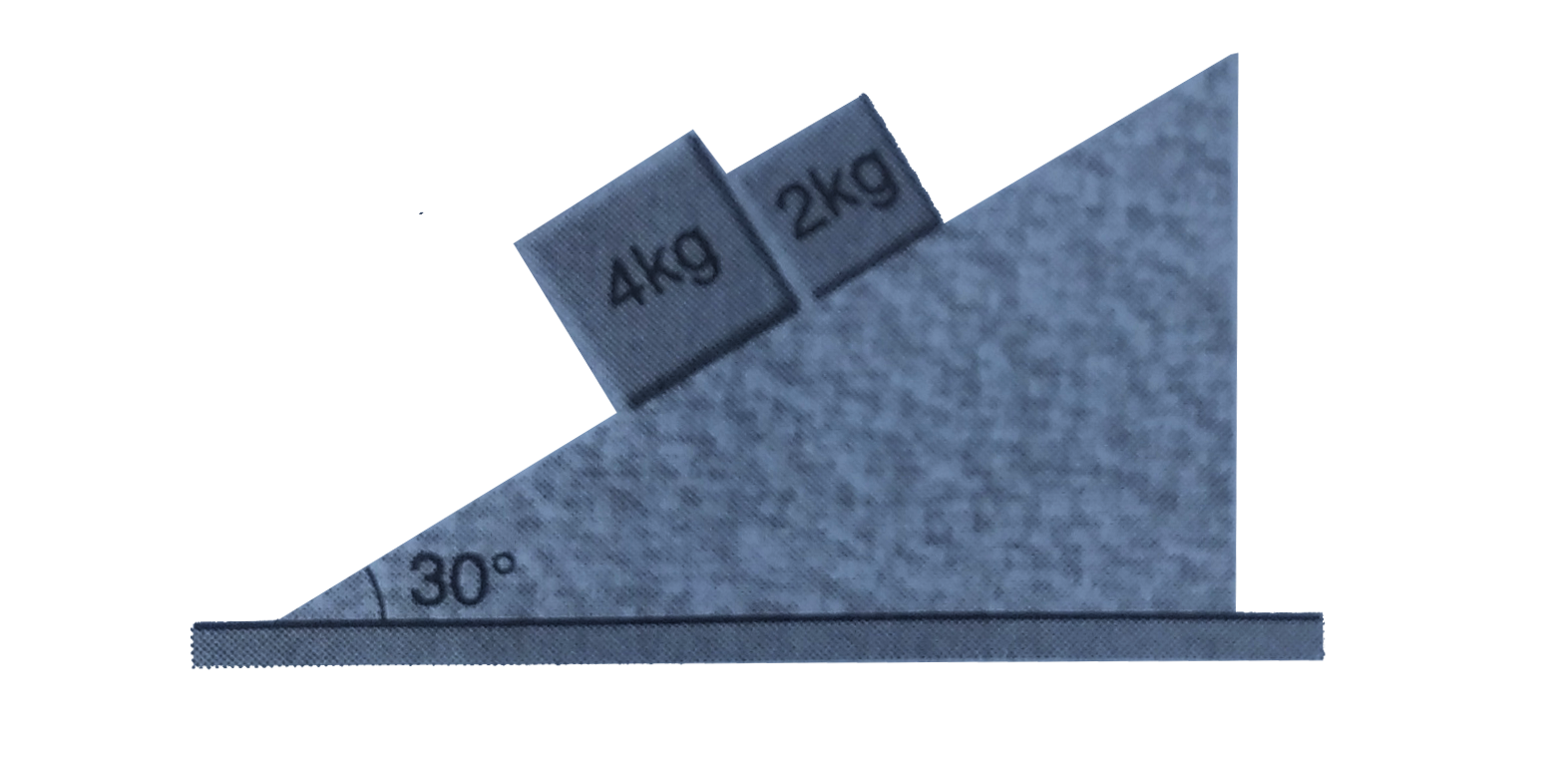
View SolutionTwo block of masses 2kg and 4kg are released from rest over a smooth inclined place of inclination 30∘ as shown in figure what is the normal force between the two blocks?
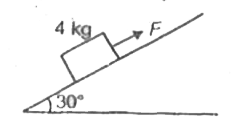
View SolutionA block of mass 4 kg is pulled along a smooth inclined plane of inclination 30∘ with constant velocity 3 m/s as shown, power delivered by the force is
View SolutionA block slides down on inclined plane (angle of inclination 60∘ ) with an accelration g/2 Find the coefficient friction

View SolutionA body of mass m is kept on a rough fixed inclined plane of angle of inclination θ=30∘. It remains stationary. Then magnitude of force acting on the body by the inclined plane is equal to :
View SolutionA block of mass 10 kg is kept on a fixed rough (μ=0.8) inclined plane of angle of inclination 30∘. The frictional force acting on the block is

View Solution