A block is gently placed on a conveyor belt moving horizontally with constant speed. After t = 4s, the velocity of the block becomes equal to the velocity of the belt. If the coefficient of friction between the block and the belt is μ=0.2, then the velocity of the conveyor belt is (g=10ms−2)
2ms−1
4ms−1
64ms−1
8ms−1
The correct Answer is:D
To solve the problem, we need to find the velocity of the conveyor belt (v) given the time (t = 4 seconds) and the coefficient of friction (μ = 0.2).
1. Understanding the Problem:
- A block is placed on a conveyor belt moving at a constant speed. The block starts from rest and accelerates due to friction until its velocity matches that of the conveyor belt after 4 seconds.
2. Identify the Forces:
- The force of friction (F_friction) acting on the block is what causes it to accelerate. This force can be expressed as:
Ffriction=μ⋅m⋅g
- Where:
- μ=0.2 (coefficient of friction)
- g=10m/s2 (acceleration due to gravity)
- m is the mass of the block (which will cancel out later).
3. Calculate the Frictional Force:
- Substituting the values into the equation:
Ffriction=0.2⋅m⋅10=2mN
4. Using Newton's Second Law:
- According to Newton's second law, the acceleration (a) of the block can be expressed as:
Ffriction=m⋅a
- Therefore, we have:
2m=m⋅a⟹a=2m/s2
5. Finding the Final Velocity:
- The final velocity (v) of the block after time t can be calculated using the equation of motion:
v=u+a⋅t
- Since the initial velocity u=0:
v=0+2⋅4=8m/s
6. Conclusion:
- The velocity of the conveyor belt, which is the final velocity of the block after 4 seconds, is:
v=8m/s
Final Answer:
The velocity of the conveyor belt is 8m/s.
- A block is placed on a conveyor belt moving at a constant speed. The block starts from rest and accelerates due to friction until its velocity matches that of the conveyor belt after 4 seconds.
- The force of friction (F_friction) acting on the block is what causes it to accelerate. This force can be expressed as:
Ffriction=μ⋅m⋅g
- Where:
- μ=0.2 (coefficient of friction)
- g=10m/s2 (acceleration due to gravity)
- m is the mass of the block (which will cancel out later).
- Substituting the values into the equation:
Ffriction=0.2⋅m⋅10=2mN
- According to Newton's second law, the acceleration (a) of the block can be expressed as:
Ffriction=m⋅a
- Therefore, we have:
2m=m⋅a⟹a=2m/s2
- The final velocity (v) of the block after time t can be calculated using the equation of motion:
v=u+a⋅t
- Since the initial velocity u=0:
v=0+2⋅4=8m/s
- The velocity of the conveyor belt, which is the final velocity of the block after 4 seconds, is:
v=8m/s
The velocity of the conveyor belt is 8m/s.
Topper's Solved these Questions
- APPENDICES (REVISION EXERCISE)BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - MOTION IN A PLANE
87 Videos
- APPENDICES ( REVISION EXERCISE )BOOK - AAKASH SERIESCHAPTER - APPENDICES ( REVISION EXERCISE )EXERCISE - REVISION EXERCISE (MAGNETISM AND MATTER )
52 Videos
- ATOMSBOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - PRACTICE EXERCISE
21 Videos
Similar Questions
A block is gently placed on a conveyor belt moving horizontal with constant speed After t=4s the velocity of the block becomes equal to velocity of the belt If the coefficient of friction between the block and the belt is μ=0.2, then the velocity of the conveyor belt is .
View SolutionA horizontal force of 10 N is necessary to just hold a block stationary against a wall. The coefficient of friction between the block and the wall is 0.2. the weight of the block is (g=10m/s2)

View SolutionA block of mass m is gently placed over a massive plank moving horizontal over a smooth surface with velocity 10ms−1. The coefficient of friction between the block and the plank is 0.2. The distance travelled by the block till it slides on the plank is [g=10ms−2]
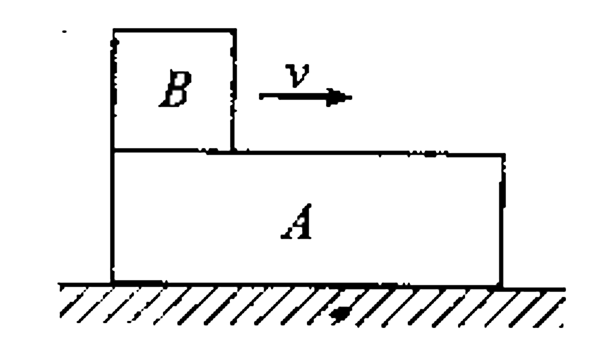
View SolutionA long block A is at rest on a smooth horizontal surface. A small block B, whose mass is half of A, is placed on A at one end and projected along A with some velocity u. The coefficient of friction between the blocks is μ:
View SolutionA block of mass 4kg is placed on a rough horizontal plane A time dependent force F=kt2 acts on the block where k=2N/s2 Coefficient of friction μ=0.8 force of friction between the block and the plane at t=2s is
View SolutionA block of mass 10 kg is placed on the rough horizontal surface. A pulling force F is acting on the block which makes an angle θ above the horizontal. If coefficient of friction between block and surface is 43 then minimum value of force required to just move the block is (g=10ms2)
View SolutionA conveyor belt is moving with constant speed of 6m/s. A small block is just dropped on it.Coefficient of friction between the two is μ=0.3. Find
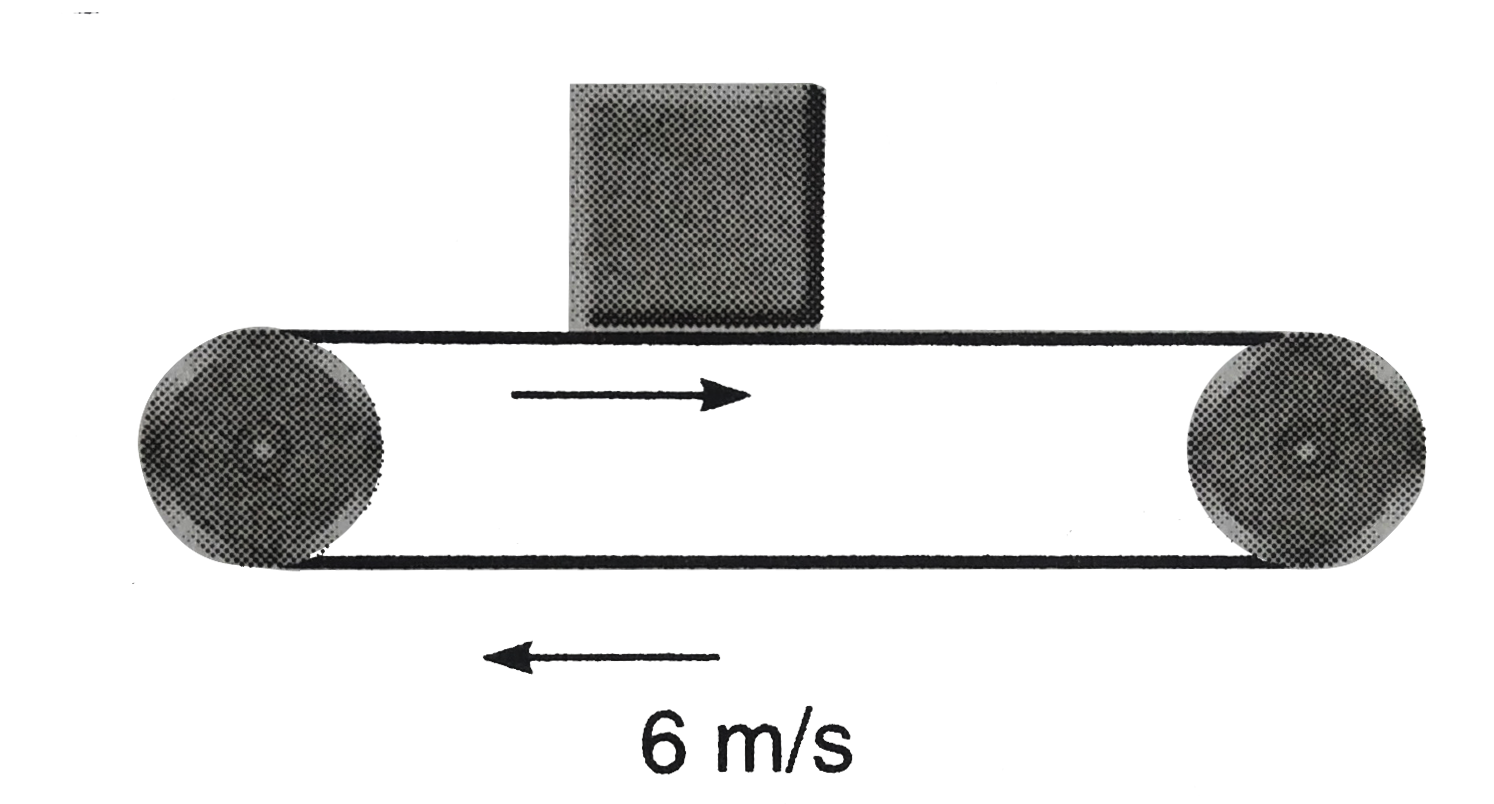
(a) The time when relative motion between them will stop
(b) Displacement of block upto that instant (g=10m/s2).
View SolutionA block of mass 2kg lying on ice when given a velocity of 6ms−1 is stopped by friction in 5s. The coefficient of friction between the block and ice is (g=10 ms−2)
View SolutionSand particles drop vertically at the rate of 2kgs−1 on a conveyor belt moving horizontally with a velocity of 0.2ms−1.
The extra force required to keep the belt moving is
View SolutionSand particles drop vertically at the rate of 2kgs−1 on a conveyor belt moving horizontally with a velocity of 0.2ms−1.
The extra force required to keep the belt moving is
View Solution
