A body of mass m0 is placed on a smooth horizontal surface . The mass of the body is decreasing exponentially with disintegration constant , λ . Assuming that the mass is ejected backwards with a relative velocity u . If initially the body was at rest , the speed of body at time t is
ueλt
uλt
ue−λt
u(1−e−λt)
To solve the problem step by step, we will analyze the situation involving a body of mass m0 that is losing mass exponentially over time due to disintegration. We will derive the speed of the body at time t given the conditions in the problem.
Step 1: Understand the mass decay
The mass of the body decreases exponentially with a disintegration constant λ. The mass at time t can be expressed as:
m(t)=m0e−λt
where m0 is the initial mass.
Step 2: Find the rate of change of mass
To find how the mass changes over time, we differentiate m(t):
dmdt=−m0λe−λt
This represents the rate at which the mass is decreasing.
Step 3: Relate mass loss to thrust force
The mass is ejected backwards with a relative velocity u. The thrust force F due to the mass loss can be expressed as:
F=u(−dmdt)=um0λe−λt
Step 4: Apply Newton's second law
According to Newton's second law, the thrust force is also equal to the rate of change of momentum:
F=m(t)dvdt
Substituting m(t) into the equation gives:
um0λe−λt=m0e−λtdvdt
Step 5: Simplify the equation
We can cancel m0e−λt from both sides (assuming m0 and e−λt are not zero):
uλ=dvdt
Step 6: Integrate to find velocity
Now, we integrate both sides with respect to time t:
∫dv=∫uλdt
This gives:
v=uλt+C
where C is the constant of integration.
Step 7: Determine the constant of integration
Since the body was initially at rest, when t=0, v=0:
0=uλ(0)+C⟹C=0
Thus, the equation simplifies to:
v=uλt
Conclusion
The speed of the body at time t is given by:
uλt
The mass of the body decreases exponentially with a disintegration constant λ. The mass at time t can be expressed as:
m(t)=m0e−λt
where m0 is the initial mass.
To find how the mass changes over time, we differentiate m(t):
dmdt=−m0λe−λt
This represents the rate at which the mass is decreasing.
The mass is ejected backwards with a relative velocity u. The thrust force F due to the mass loss can be expressed as:
F=u(−dmdt)=um0λe−λt
According to Newton's second law, the thrust force is also equal to the rate of change of momentum:
F=m(t)dvdt
Substituting m(t) into the equation gives:
um0λe−λt=m0e−λtdvdt
We can cancel m0e−λt from both sides (assuming m0 and e−λt are not zero):
uλ=dvdt
Now, we integrate both sides with respect to time t:
∫dv=∫uλdt
This gives:
v=uλt+C
where C is the constant of integration.
Since the body was initially at rest, when t=0, v=0:
0=uλ(0)+C⟹C=0
Thus, the equation simplifies to:
v=uλt
The speed of the body at time t is given by:
uλt
Topper's Solved these Questions
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - More a than one option is Correct
1 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Medical entrances gallery
27 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective
21 Videos
Similar Questions
If mass of a body is M on the earth surface, then the mass of the same body on the moon surface is
View SolutionA body of mass 5 kg is at rest on a smooth horizontal surface. A horizontal force 'F' acts on it for 4s and then ceases to act. If the body travels 40 m in the next 5s, the value of F is,
View SolutionThe horizontal component of the weight of a body of mass m is
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

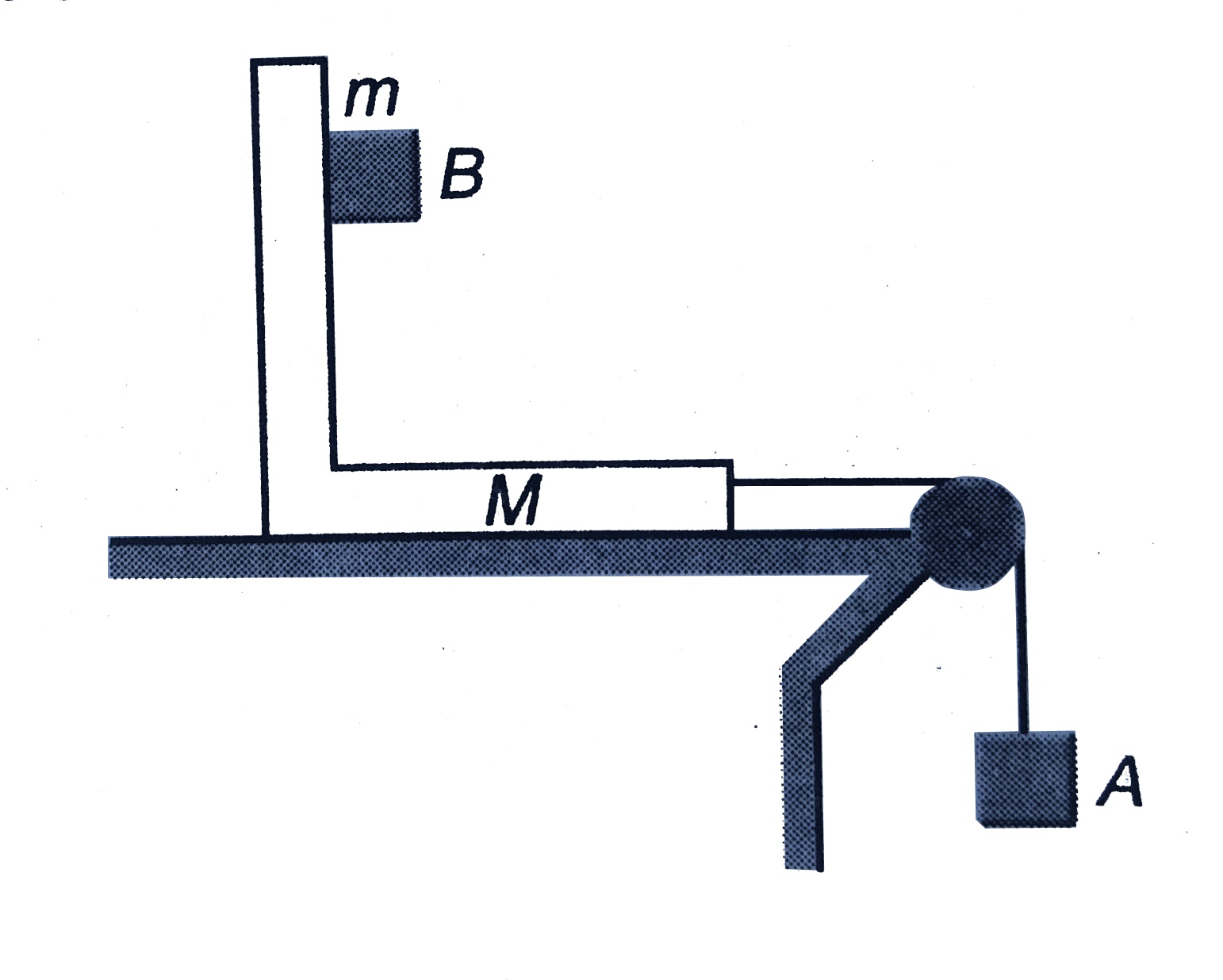
View SolutionThe figure an L shaped body of mass M placed on smooth horizontal surface. The block A is connected to the body by means of an inexiensible string , which is passing over a smooth pulley of negligible mass.Another block B of mass m is placed against a vertical wall of the body. Find the minimum value of the mass of block A so that B remain stationary relative to the wall. Coefficient of friction between the block B and the vertical wall is μ.
View SolutionA body of mass m is placed at the centre of the spherical shell of radius R and mass M. The gravitation potential on the surface of the shell is
View SolutionA body of mass m is placed on a rough surface with coefficient of friction μ inclined at θ. If the mass is in equilibrium , then
View SolutionA body of mass m is placed on a rough surface with coefficient of friction μ inclined at θ. If the mass is in equilibrium , then
View SolutionA body of mass m is projected with intial speed u at an angle θ with the horizontal. The change in momentum of body after time t is :-
View SolutionA man of mass m is standing on a plank of equal mass m resting on a smooth horizontal surface. The man starts moving on the plank with speed u relative to the plank. The speed of the man relative to the ground is

View Solution
