A body of weight 27N hangs on a long spring of such stiffness that an extra force of 9N stretches the spring by 0.05m. If the body is pulled downward and released, what is the period ?
F=kx
∴k=Fx=90.05=180N/m
m=Wg=2710=2.7kg
T=2π√mk=2π√2.7180
=0.78s
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 Single Correct
Explore 28 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Level 2 More Than One Correct
Explore 8 Videos
- SIMPLE HARMONIC MOTIONBook:DC PANDEYChapter:SIMPLE HARMONIC MOTIONExercise:Level 1 Single Correct
Explore 24 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBook:DC PANDEYChapter:SEMICONDUCTORS AND ELECTRONIC DEVICESExercise:More than One Option is Correct
Explore 3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBook:DC PANDEYChapter:SOLVD PAPERS 2017 NEET, AIIMS & JIPMERExercise:Solved paper 2018(JIPMER)
Explore 38 Videos
Similar Questions
A body of mass 12 kg is suspended by a coil spring of natural length 50 cm and force constant 2.0×10Nm−1. What is the stretched length of the spring ? If the body is pulled down further stretching the spring to a length of 59 cm and then released, what is the frequency of oscillation of the suspended mass ? (Neglect the mass of the spring).
View SolutionA sand bag of mass m is hanging from a light spring of stiffness k. Find the elongation of the spring. If we pull the sand bag down by an additional distance x and release it, find its acceleration and maximum velocity of block.
View Solution
Knowledge Check
When a mass m is hung on a spring, the spring stretched by 6 cm. If the loaded spring is pulled downward a little and released, then the period of vibration of the system will be
A0.27 sB0.35 sC0.49 sD0.64 sA body of weight 30N hangs from one end of a long, vertical spring whose other end is attached to a rigid support. The spring extends by 5cm when a force of 10N is applied to it. The time period of the vertical oscillations of the body is
A2√(15π)sB(π/5)√(3/2)secC(π/10)√(3/2)secD20)√(2/3)secA body of mass 100 g is attached to a hanging spring force constant is 10N/m. The body is lifted until the spring is in its unstretched state and then released. Calculate the speed of the body when it strikes the table 15cm release point .
A1m/sB0.866m/sC0.225m/sD1.5m/s
A body of mass 10 kg is suspended by a massless coil spring of natural length 40 cm and force constant 2.0×103Nm−1. What is stretched length of the spring.? If the body is pulled down further stretching the spring to a length 48cm and then released, what is the frequency of oscillations of the suspended mass ? g=10ms2
View SolutionWhen a body of mass 2.0 kg is suspended by a spring, the spring is stretched. If the body is pulled down slightly and released, it coscillated up and down. What force is applied on the body by the spring when it passes through the mean position ? (g=9.8Nkg−1)
View SolutionA block with a mass of 3.00kg is suspended from an ideal spring having negligible mass and stretches the spring by 0.2m.
(a) What is the force constant of the spring?
(b) What is the period of oscillation of the block if it is pulled down and released ?
View SolutionA 4-kg block extends a spring by 16 cm from its unstretched postion .The block is removed and a 0.5 -kg boby is hung from the same spring If the spring is then stretched and released , what is the time period of oscillations of the mass suspendrd ? What is the force constant of the spring ?
View SolutionA body of mass 0.4 kg is held between two massless springs whose ends are fixed to rigid walls as shown in the figure. The spring constants are 8 N/m and 2 N/m. If the body is displaced along the direction of the lengths of the springs and released, the time period (in second) of the oscillations will be (ignore vertical oscillation, if any )
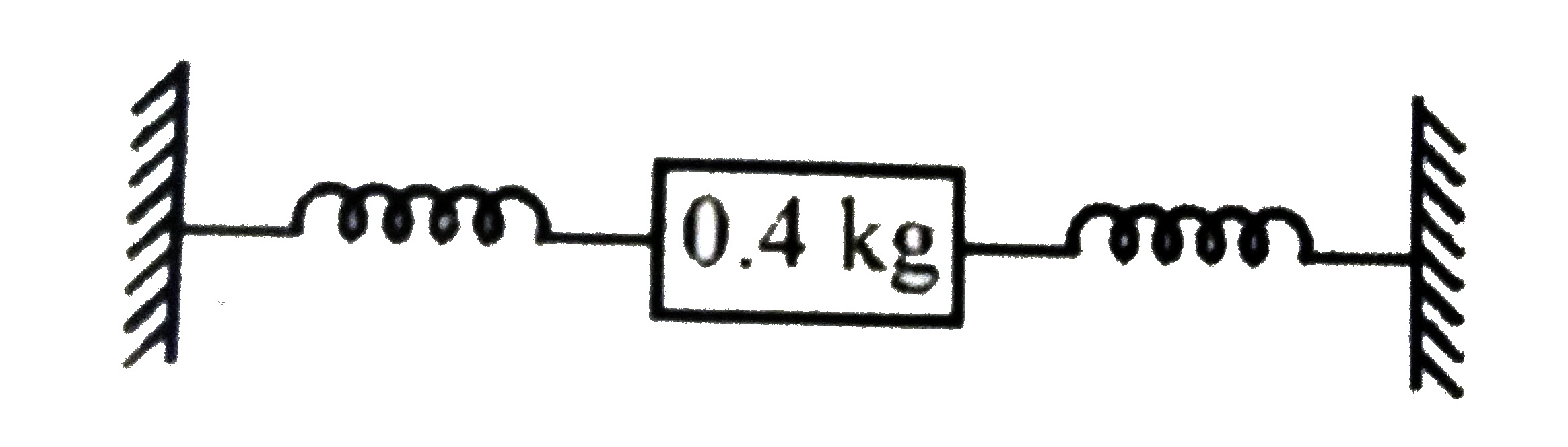
View Solution
