A body P of mass 20 g and another body Q of mass 40 g are projected at the same time from points A and B on the earth's surface. Velocity of projection for each was 49 m⋅s−1 and it was directed at an angle of 45∘ with the horizontal. Distance AB = 245m. P and Q collide on the same vertical plane. After collision, P retraces its path to the ground. Find the position where Q touches the ground. How long will Q take to reach the ground after the collision? [ g = 9.8 m⋅s−2]
![A body P of mass 20 g and another body Q of mass 40 g are projected at the same time from points A and B on the earth's surface. Velocity of projection for each was 49 mcdot s^(-1) and it was directed at an angle of 45^(@) with the horizontal. Distance AB = 245m. P and Q collide on the same vertical plane. After collision, P retraces its path to the ground. Find the position where Q touches the ground. How long will Q take to reach the ground after the collision? [ g = 9.8 mcdot s^(-2)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/CHY_DMB_PHY_XI_P1_U03_C01_SLV_030_Q01.png)
As the two bodies P and Q projected at the same time the same velocity u = 49 m ⋅s−1 and angle = 45∘, horizontal range for both P and Q is the same and equal to.
u2sin2αg=(49)2×sin90∘9.8=245m.
Hence the two bodies P and Q will meet at a point on the perpendicular bisector of AB.
Let the time when P,Q meet after projection be t. Hence horizontal distance moved be each
x = 49 cos45∘×t=2452, or,t=5√2s
During the collision, vertical velocity of P and Q
uy=usin45∘−gt=49√2−9.8×5√2=0
Horizontal velocity of P and Q
ux=ucos45∘=49√2m⋅s−1
Hence horizontal component of the total momentum befor collision
Px=(0.02ux−0.04ux)kg⋅m⋅s−1
P retraces its path after collision. Hence horizontal velocity of P after collision = -ux and for Q it is = v (say).
Hence horizontal component of the total momentum of the system after the collision
=(−0.02ux+0.04v)kg⋅m⋅s−1
From the law of conservation of momentum,
= −0.02ux+0.04ux=(−0.02ux+0.04v)
or, v = 0
Thus Q does not have only horizontal componet fo velocity and hence if falls down vertically at the midpoint of AB, at a distance 2452 = 122.5 m from both A and B Let t = time required by Q to reach the ground after the colision.
t=usin45∘g=49×1√2×9.8 = 3.53 s.
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - SECTION RELATED QUESTION10videos
NEWTON'S LAWS OF MOTION
- BOOK - CHHAYA PUBLICATIONCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - HIGHER ORDER THINKING SKILL (HOYS) QUESTIONS26videos
NEWTON'S LAWS OF MOTION
- BOOK - CHHAYA PUBLICATIONCHAPTER - NATURE OF VIBRATIONEXERCISE - EXERCISE (CBSE SCANNER)2videos
NATURE OF VIBRATION
- BOOK - CHHAYA PUBLICATIONCHAPTER - NEWTONIAN GRAVITATION AND PLANETARY MOTIONEXERCISE - CBSE SCANNER19videos
NEWTONIAN GRAVITATION AND PLANETARY MOTION
Similar Questions
Particles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)
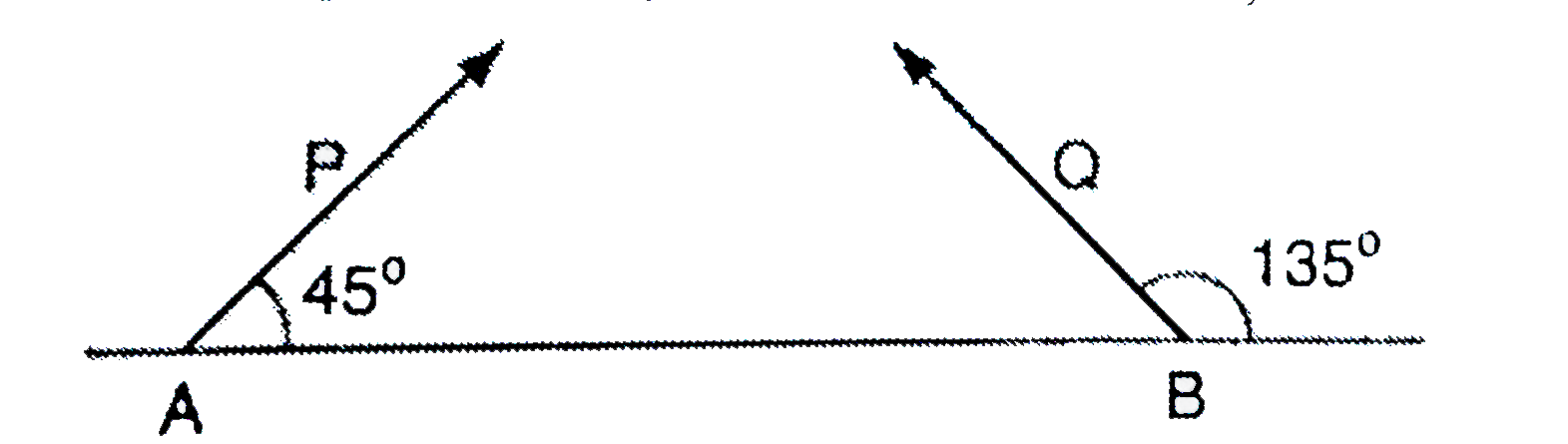 View Solution
View SolutionParticles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A body is projected at an angle of 60∘ with ground. It covers a horizontal distance of 100m. If the same body is projected at 60∘ with vertical with same velocity, the new range is
A50mB100mC200mD150m - Question 2 - Select One
The escape velocity for a body projected vertically upwards from the surface of earth is 11km/s. If the body is projected at an angle of 45∘ with the vertical, the escape velocity will be
A11√2kmsB22km/sC11km/sD11√2kms - Question 3 - Select One
The escape velocity for a body projected vertically upwards from the surface of earth is 11km/s. If the body is projected at an angle of 45∘ with the vertical, the escape velocity will be
A11√2 km/sB11√2 km/sC22 km/sD11 km/s
Particles P and Q of mass 20g and 40g respectively are simu ltaneously proejected from points A and B on the ground. The initial velocities of P and Q make 45∘ and 135∘ angles respectivley with the horizontal AB as shown in the Fig. 5.44 Each particle has an initial speed of 49m/s . the separation AB is 249m. both particles travel in the same vertical plane and undergo a collision. After collision P retraces its path. Determine the position of q when it hits the grou.d How much time after the collision does the particle Q take to reach the ground? (Take g=9.8m/s2)
 View Solution
View SolutionA body of mass m1 collides elastically with another body of mass m2 at rest. If the velocity of m1 after collision is 23 times its initial velocity, the ratio of their masses is :
View SolutionThe escape velocity for a body projected vertically upwards from the surface of the earth is 11.2kms−1. If the body is projected in a direction making an angle 45∘ with the vertical, the escape velocity will be
View SolutionParticles P and Q of masses 20g and 40g, respectively, are projected from positions A and B on the ground. The initial velocities of P and Q make angles of 45∘ and 135∘, respectively with the horizontal as shown in the fig. Each particle has an initial speed of 49m/s. The separation AB is 245m. Both particles travel in the same vertical plane and undergo a collision. After the collision P retraces its path. The separation of Q from its initial position when it hits the ground is
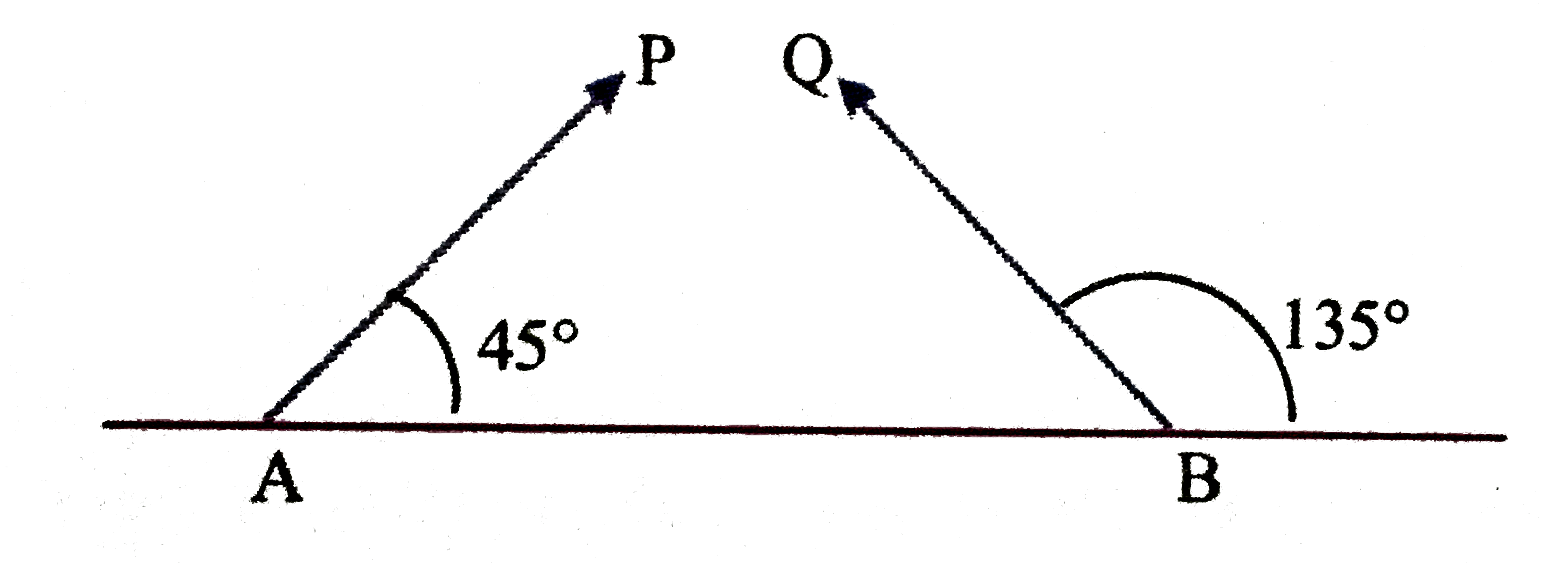 View Solution
View SolutionA body of mass m1 collides elastically with another body of mass m2 at rest. If the velocity of m1 after collision is 23 times its initial velocity, the ratio of their masses is :
View Solution
