A body sliding on a smooth inclined plane requires 4s to reach the bottom, starting from rest at the at the top. How much time does it take to cover ont-foruth the distance startion from rest at the top?
1 s
2s
4s
16s
The correct Answer is:B
To solve the problem, we need to determine the time it takes for a body sliding down a smooth inclined plane to cover one-fourth of the distance when starting from rest at the top. We know that the body takes 4 seconds to reach the bottom of the incline.
1. Understanding the Motion:
The body is sliding down the incline under the influence of gravity. The motion can be described using the equations of uniformly accelerated motion.
2. Using the Second Equation of Motion:
The second equation of motion states:
s=ut+12at2
where:
- s is the distance covered,
- u is the initial velocity (which is 0 since it starts from rest),
- a is the acceleration (which is gsinθ for the incline),
- t is the time taken.
3. Distance for the Entire Incline:
For the entire distance l covered in 4 seconds:
l=0⋅4+12(gsinθ)(42)
Simplifying this gives:
l=12(gsinθ)(16)=8gsinθ
4. Distance for One-Fourth of the Incline:
Now, we want to find the time t taken to cover l4:
l4=12(gsinθ)t2
Substituting l=8gsinθ:
8gsinθ4=12(gsinθ)t2
This simplifies to:
2gsinθ=12(gsinθ)t2
5. Cancelling Common Terms:
We can cancel gsinθ from both sides (assuming gsinθ≠0):
2=12t2
6. Solving for t2:
Multiplying both sides by 2 gives:
4=t2
Taking the square root:
t=2 seconds
Final Answer:
The time taken to cover one-fourth of the distance starting from rest at the top is 2 seconds.
---
The body is sliding down the incline under the influence of gravity. The motion can be described using the equations of uniformly accelerated motion.
The second equation of motion states:
s=ut+12at2
where:
- s is the distance covered,
- u is the initial velocity (which is 0 since it starts from rest),
- a is the acceleration (which is gsinθ for the incline),
- t is the time taken.
For the entire distance l covered in 4 seconds:
l=0⋅4+12(gsinθ)(42)
Simplifying this gives:
l=12(gsinθ)(16)=8gsinθ
Now, we want to find the time t taken to cover l4:
l4=12(gsinθ)t2
Substituting l=8gsinθ:
8gsinθ4=12(gsinθ)t2
This simplifies to:
2gsinθ=12(gsinθ)t2
We can cancel gsinθ from both sides (assuming gsinθ≠0):
2=12t2
Multiplying both sides by 2 gives:
4=t2
Taking the square root:
t=2 seconds
The time taken to cover one-fourth of the distance starting from rest at the top is 2 seconds.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Graphical Concept17videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Graphical cancept1videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Subjective29videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer9videos
KINEMATICS-2
Similar Questions
A body dropped from the top of a tower hits the ground after 4 s. How much time does it take to cover the first half of the distance from the top of the tower?
View SolutionA block is released from top of a smooth inclined plane.It reaches the bottom of the plane in 6 sec.The time taken by the body to cover the first half of the inclined plane is:
View Solution
Knowledge Check
- Question 1 - Select One
A solid sphere rolls down a smooth inclined plane of height h. If it stats from rest then the speed of the sphere when it reaches the bottom is given by
A√ghB√107ghC√47ghD√54gh - Question 2 - Select One
A solid sphere, resting at the top of a smooth inclined plane of inclination 30∘ with the horizontal, rolls down the plane and reaches the bottom, which is at 15.75 m from the top. How much time it will take to reach the bottom ?
A2 sB2.5 sC3 sD4 s - Question 3 - Select One
Two identical solid cylinders run a race starting from rest at the top of an inclined plane. If one cylinder slides and the other rolls :
ASliding cylinder will reach the bottom first with greater speed.BRolling cylinder will reach the bottom first with greater speed.CBoth will reach the bottom simultaneously with the same speed.DBoth will reach the bottom simultaneously but with different speeds.
A block is released on an smooth inclined plane of inclination θ. After how much time it reaches to the bottom of the plane?
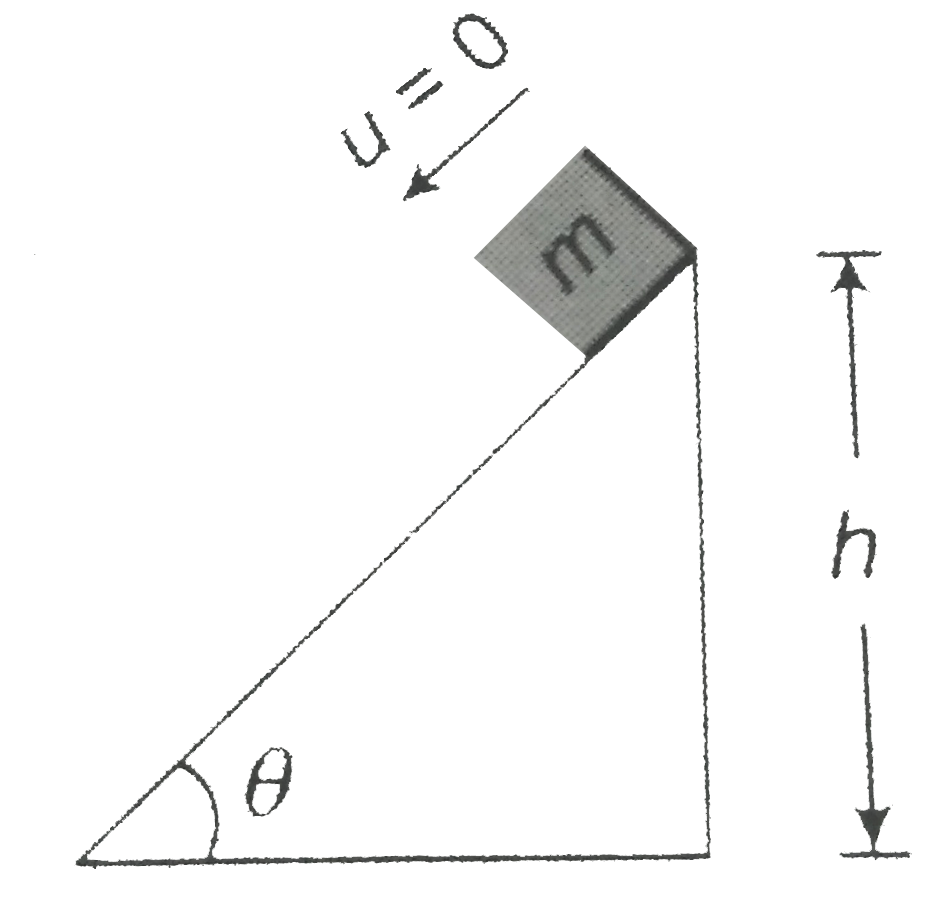 View Solution
View SolutionIf the body starts from rest from the top of the rough inclined plane of length 1, time taken to reach the bottom of the plane is
View SolutionA block is released from top of a smooth inclined plane It reaches the bottom of the plane in √2s The time taken (in second) by the body to cover Ist half of inclined plane is .
View SolutionA smooth inclined plane is inclined at an angle θ with the horizontal. A body starts from rest and slides down the inclined surface. The time taken by the body to reach the bottom is
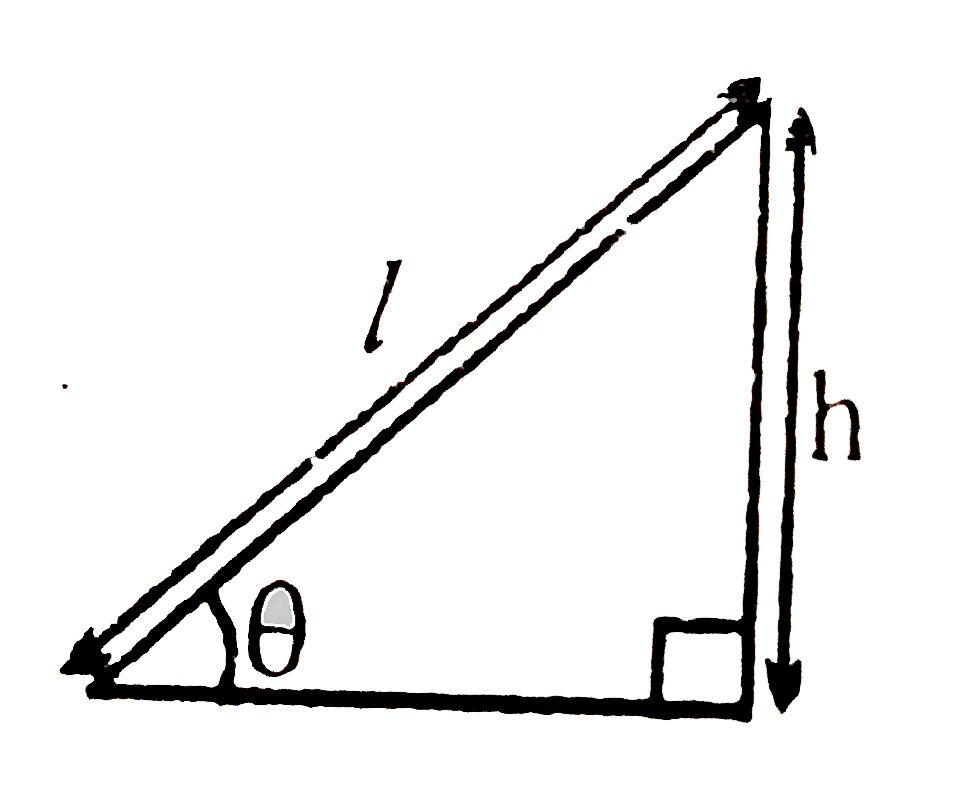 View Solution
View SolutionA block released from rest from the top of a smooth inclined plane of angle θ1 reaches the bottom in time t1. The same block released from rest from the top of another smooth inclined plane of angle θ2 reaches the bottom in time t2 If the two inclined planes have the same height, the relation between t1andt2 is
View Solution

