A body starts falling from the top of a smooth sphere of radius r. What angle does the body subtend at the centre of the sphere when it just loses contact with the sphere and what will be its velocity then ?
Let the angle subtended by the body at the centre of the sphere when it loses contact with the sphere be θ.
The body just loses contact with the sphere when the component of gravitational force cannot provide the necessary centripetal force to the body
so that it can continue in the circular path.
So, according to Fig .1.36.

mgcosθ=mv2r...(1)
(v= velocity of the body when it just loses contact with the sphere)
According to the principle of conservation of energy,
12mv2=mgr(1−cosθ)
[OC=OA=r,OB=rcosθBC=OC−OB=r−rcosθ=r(1−cosθ)]
or, v2=2gr(1−cosθ)
From equations (1) and (2) we get,
mgcosθ=mr⋅2gr(1−cosθ)
or, cosθ=2(1−cosθ)
or,cosθ=23or,θ=cos−123=48.2∘
again ,v2=2gr(1−cosθ)=2gr(1−23)=23gr
∴v=√23gr.
Topper's Solved these Questions
- WORK AND ENERGYBook:CHHAYA PUBLICATIONChapter:WORK AND ENERGYExercise:SECTION RELATED QUESTIONS
Explore 24 Videos
- WORK AND ENERGYBook:CHHAYA PUBLICATIONChapter:WORK AND ENERGYExercise:HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
Explore 38 Videos
- WBCHSE QUESTION PAPER -2019Book:CHHAYA PUBLICATIONChapter:WBCHSE QUESTION PAPER -2019Exercise:SECTION - II ( GROUP -D)
Explore 10 Videos
Similar Questions
Steradian is the solid angle subtended at the centre of the sphere by its surface whose area is equal to ____ of the sphere.
View SolutionA small body A starts sliding off the top of a smooth sphere of radius R. Find the angle θ (figure) corresponding to the point at which the body breaks off the sphere, as well as the break-off velocity of the body.

View Solution
Knowledge Check
A particle moving on the inside of a smooth sphere of radius r describing a horizontal circle at a distance r/2 below the centre of the sphere. What is its speed ?
A√5grB√4gr/3C√3gr/2D√√3grThe radius of gyration of a solid sphere of radius r about a certain axis is r. The distance of this axis from the centre of the sphere is
ArB0.5 rC√0.6rD√0.4rA small body of mass 'm' is placed at the top of a smooth sphere of radius 'R' placed on a horizontal surface as shown, Now the sphere is given a constant horizontal acceleration a0=g. The velocity of the body relative to the sphere at the moment when it loses contact with the sphere is
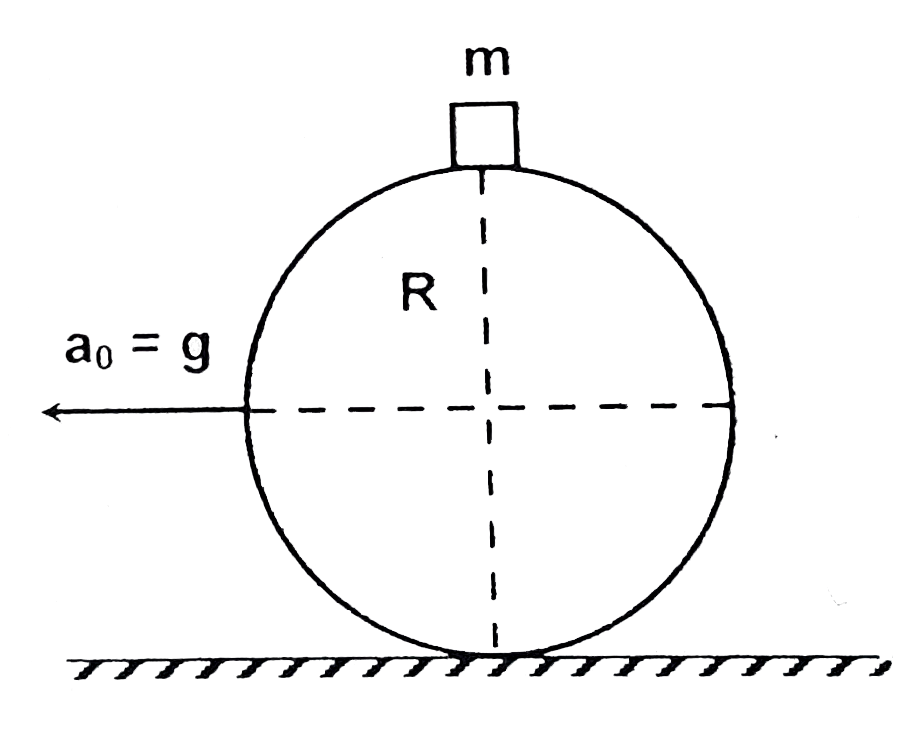 A√gR3B√2gR3C√5gR3D√7gR3
A√gR3B√2gR3C√5gR3D√7gR3
A particle is projected with velocity u horizontally from the top of a smooth sphere of radius a so that it slides down the outside of the sphere. If the particle leaves the sphere when it has fallen a height a4 , the value of u is
View SolutionA small body of mass 'm' is placed at the top of a smooth sphere of radius 'R' placed on a horizontal surface as shown, Now the sphere is given a constant horizontal acceleration a0=g. The velocity of the body relative to the sphere at the moment when it loses contact with the sphere is

View SolutionA particle slides on the surface of a fixed smooth sphere starting from the topmost pont. Find the angle rotated by the radius through the particle, when it leaves contact with the sphere.
View SolutionA particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
View SolutionA particle of mass m starts to slide down from the top of the fixed smooth sphere. What is the tangential acceleration when it break off the sphere ?
View Solution
